A SOUTHERN AFRICAN SILVER ANNIVERSARY MEETING, 2014 SOMP

 

Geomechanics challenges of contemporary deep mining: a suggested model for increasing future mining safety and productivity

 

 

F.T. Suorineni; B. Hebblewhite; S. Saydam

UNSW Australia School of Ming Engineering, Australia

 

 

---

SYNOPSIS

This paper pays tribute to the pioneers in geomechanics, and addresses some of the most pressing issues of our time related to deep mining, and how to mitigate these issues. Solutions developed by our predecessors seem to have reached their limits as mines continue to go deeper. The International Society for Rock Mechanics (ISRM) guidelines for rock mass characterization for excavation design also require re-thinking to reflect current knowledge and experience. Thus, a whole new approach is urgently required to increase mine safety and productivity. The paper will draw on the challenges and experiences in medicine and science that have been overcome through genuine collaboration, advances in technology, and impressive funding, which can be adopted to provide solutions to contemporary geomechanics challenges for increased safety and productivity as mines continue to go deeper.

Keywords: pioneers in geomechanics, pressing issues, new thinking, collaboration.

---

 

 

Introduction

Rock mechanics, and therefore geomechanics, is a relatively young subject compared to soil mechanics in geotechnical engineering. Suorineni (2013a) discussed in detail the difference between geomechanics and geotechnical engineering. Most failure criteria and test procedures in rock mechanics are adopted from soil mechanics and the mechanics of solids such as steel. The Mohr-Coulomb failure criterion (Mohr, 1900) is one such example and remains popular in rock mechanics today. Approaches to rock testing (such as triaxial testing) are adopted from soil mechanics. However, there is a fundamental difference between soil, concrete, steel, and rock, and in particular between soil and rock. Craig (1982) defines soil as any uncemented or weakly cemented accumulation of mineral particles formed by the weathering of rocks, the void space between the particles containing particles and/or air. On the other hand, in geology, a rock is a naturally occurring solid aggregate of one or more minerals or mineraloids (http://en.wikipedia.org/wiki/Rock(geology)). The key words in these definitions are 'uncemented' and 'aggregate' for soil and rock respectively. These keywords explain why a failure criterion for soils such as the Mohr-Coulomb failure criterion (Equation [1]) will work for soils, but is not applicable to rock.

where 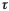is the shear strength, c is cohesion, and is angle of internal friction.

As noted by others (e.g. Hajiabdolmajid et al., 2000) Equation [1] implies that the shear strength of soil is determined by simultaneous mobilization of its cohesive resistance and frictional resistance. This is correct for 'uncemented' materials such as soil, but incorrect for an 'aggregate' of particles or minerals as in rocks. In the latter case the bond (cohesion) between the minerals need to be broken to generate frictional resistance and therefore the cohesive and frictional strength components cannot be simultaneously mobilized. Hence, some adoptions of experience in soil mechanics into rock mechanics can be misleading.

While soil and steel can be assumed to satisfy the criteria of continuity, homogeneity, isotropicity, linearity, and elasticity (CHILE), applying these assumptions to the rock leads to difficulty. Muller (1966) was first to recognize that the rock mass is a discontinuum, and has continuously emphasized the importance of geology in rock mechanics or geomechanics throughout his distinguished life and career. In 1988, Muller wrote:

'Geology is the indispensable base of all the appliedgeosciences. Therefore, never Rock Mechanics without Engineering Geology;... But should I become confronted with the alternative: Rock Mechanics without Engineering Geology or Engineering Geology

On the passing of Dr J.A. Franklin in June 2012, Professor M.B. Dusseault sent out the following message:

"... John profoundly understood the intersection between geosciences and rock engineering, an attitude that pervaded his career.'

Franklin (1993) wrote: 'Empirical methods often prove "closer" to the "truth" than the apparently more precise predictions of theoretical analysis, ... based on real data empirical methods provide a standard against which theoretical predictions are measured and can be judged.'

Similarly, Mathews et al. (1981), in developing the stability graph, stated that empirical methods based on back-analysis are powerful predictive tools, particularly if combined with numerical modelling and analysis techniques. Note that this statement regards theoretical predictions as supporting tools rather than stand-alone tools.

The importance of geology in rock mechanics, geomechanics, or geotechnical engineering cannot be overemphasized. This fact is underlain by the complexity of the rock mass as opposed to soil, concrete, or steel. The complexity of the rock mass cannot be fully accounted for in our constitutive models that underlie numerical models. While these numerical models can be tricked into giving us the answers we want, the rock mass is so idiosyncratic that it will behave in the manner it wants. Muller (1988) noted that the dominating geological conditions at site do not care what kind of theoretical ideas we may have and what our economic situation may be. He continued to state that the rock mass will act in the way that is predetermined by geological conditions on the one hand, as well as by the manner in which we treat it during excavation and support.

Pells (2008) posed the ultimate question: 'what happened to the mechanics in rock mechanics and the geology in engineering geology?' Pells's question separated rock mechanics and engineering geology and treated them independently. As shown above, rock mechanics cannot be treated from the purely mechanistic viewpoint without due consideration to the underlying geological complexities of the rock mass. As rightly pointed out by Peck (see Muller, (1988) 'where has all our judgement gone?' According to Peck, a good rock mechanics engineer should have good intuition to guide his decisions - a characteristic that the majority of the young generation of engineers today lacks, as a consequence of deficient knowledge in geology and field experience. Peck's concern is re-echoed by Karl Terzaghi: 'The geotechnical engineer should apply theory and experimentation but temper them by putting them into the context of the uncertainty of nature. Judgement enters through engineering geology.'

At the 44th United States Rock Mechanics Symposium, which was also the 4th United States-Canada Rock Mechanics Symposium, a pre-conference workshop was organized with invited panellists including Don Banks, William Pariseau, Maurice Dusseault, John Curran, Richard Goodman, and Charles Dowding, with Priscilla Nelson as moderator, to present their perspectives on the important achievements of rock mechanics and engineering in the past 50 years, and to identify what we did not achieve. The most common issue and problem identified was the deficiency in the training of rock mechanics engineers today. That deficiency is the absence of sufficient geology in the curricula of civil and mining engineering programmes.

It cannot be overemphasized that the great pioneers of rock mechanics, geomechanics, and engineering geology (including Karl Terzaghi, Ralph Peck, Leopold Muller, Evert Hoek, John Franklin, Denis Laubscher, Nick Barton, Z.T. Bieniawski, and Rimas Pakalnis) recognized the importance of geology, observed and learnt from the idiosyncratic rock behaviour, and guided by their intuition managed to discipline it. Empirical methods are the outcome of patient observations, intuition, and a keen interest in geology.

This paper pays tribute to these great men, but recognizes that although their contributions worked well to solve the problems of their time, they have now reached their limits and new thinking is urgently required. The paper draws on lessons from medicine and science to suggest that for significant breakthroughs in rock mechanics or geomechanics, genuine multidisciplinary approach supported by generous funding and rigorous overview is required. The next sections address these requirements in detail.

 

Empirical methods - state-of-the-art

The following empirical methods are discussed in view of their popularity and widespread use in geomechanics:

(i) The Rock Mass Rating (RMR) system (Bieniawski, 1973)

(ii) The Laubscher rules in block cave mining (Laubscher, 1994)

(iii) The open stope stability graph (Mathews et al., 1981; Clark and Pakalnis, 1997)

(iv) The hard-rock pillar design graph (Lunder and Pakalnis, 1997)

(v) The Tunnelling Quality Index (Q) system (Barton et al., 1974)

(vi) The Hoek and Brown failure criterion (Hoek and Brown, 1980).

These methods all depend on proper characterization of the rock mass. Critical factors in these methods depend on the purpose. Such purposes include support selection, caveability prediction, determination of rock mass properties for design, and evaluation of the stability of open stopes. In each specific case there are insufficient guidelines from the International Society for Rock Mechanics (ISRM) Suggested Methods for Rock Characterization, Testing and Monitoring (Brown 1981). Obvious difficulties are encountered in the caveability of rock masses. The ISRM suggestion that induced fractures be ignored in geotechnical mapping for rock mass characterization is suspect when the purpose of such a mapping is for support selection.

The Rock Mass Rating (RMR) system

RMR (Bieniawski 1973) became the most widely accepted and used rock mass classification system following its development in 1973. Its popularity stemmed from the fact that it could be used for excavation design in rock with significant capacity to predict excavation stand-up time. RMR was also adopted by Hoek and Brown (Hoek and Brown, 1980) for the determination of the Hoek and Brown failure criterion parameters. RMR was replaced by the Geological Strength Index (GSI) in 1995 (Hoek et al., 1995) for that role because it became ineffective in performing that function for values of RMR less than about 25.

Various critiques of RMR have been published, out of which evolved the Modified or Mining Rock Mass Rating (MRMR) system (Laubscher and Taylor, 1976). Milne et al. (1998) outline a chronological development of the method between 1973 and 1989. Within this period the RMR factors have been modified as more experience in its application was gained.

Suorineni (2013b) critically re-examined the original RMR database for its validity, robustness, and application independent of the geological environments or rock types and depth. The validity of the method with regard to its stand-up time prediction was also examined. Figure 1 summarizes the composition of the RMR database in terms of rock origin as published in Bieniawski (1989). The figure shows that the RMR (1989) database consists of 63% sedimentary rocks, 17% metamorphic, and 20% igneous rocks. Of the 63% sedimentary rocks, about 45% are shale (Figure 2). Figure 3 is a plot of depth against frequency of data points. The figure shows that about 90% of the data came from depths less than 500 m below surface.

 

 

 

 

 

 

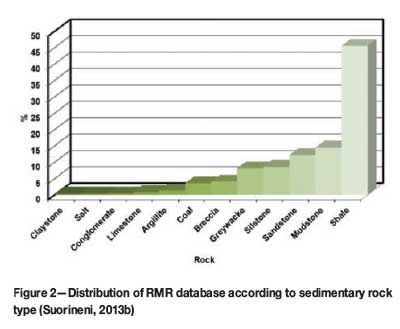
From Figures 1 to 3 it is obvious that the RMR database comprises mainly soft rocks, dominated by shales, from depths less than 500 m below surface. The significance of this revelation for the composition of the RMR database is discussed under the Laubscher block caving rules (Laubscher, 1994, 2001) later in this paper. The implication of the database as it relates to stand-up time (Figure 4) of excavations is discussed here.

 

 

Sedimentary rocks, and in particular, shales, are susceptible to the vagaries of the environment on exposure, depending on their composition. Domination of the RMR database by sedimentary rocks gives logic to the stand-up time concept. While this may also be true for some igneous rock such as olivine-rich rocks, it is difficult to argue the concept of stand-up time for excavations in these rocks. As pointed out by Muller (1988), while timing is important in rock engineering or geomechanics it can hardly be computed or even assessed without deep geological knowledge and intuition. This is further buttressed by the fact that 'one cannot wait until the rock itself announces its stand-up time by roof falls and slabbing of the side walls' (Muller, 1988). One must make decisions before the stand-up time is reached. Isaacson (2007) states that Albert Einstein's intelligence and breakthroughs hinged on his ability to mix intuition with a feel for the patterns to be found in experimental data. He adds 'The scientist has to worm these general principles out of nature by discerning, when looking at complexes of empirical facts, certain general features.'

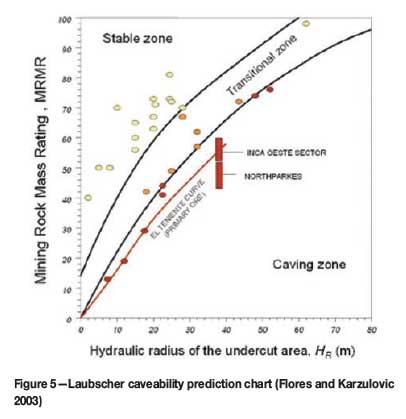
Laubscher block caving rules

The Laubscher block caving rules are based on a modified RMR, the MRMR. Figure 5 is a chart for predicting the critical undercut size (hydraulic radius - HR) in a rock mass of given MRMR quality to induce natural caving under gravity. RMR, and for that matter MRMR, were developed between 1973 and 1976 respectively. The recent modification to the MRMR termed IRMR (Laubscher and Jakubec, 2000) is not significantly different (Dyke, 2008) from the MRMR. At time of development of RMR and MRMR most block caving mines were operating at depths less than 450 m (Figure 6). The rocks in the RMR database involved are mainly weak and dominated by shales, with most data coming from depths less than 500 m below surface. Block caving mines today are located at depths far in excess of 500 m and in metamorphic and/or igneous geological environments. Under these conditions, stronger rocks at depths will be subjected to higher confinements and the Laubscher caving rules become suspect. It is the opinion of the authors that while the Laubscher caving rules would have been effective at the time of their development, they have reached their limits in contemporary block caving practice and new thinking is required.

 

 

 

 

The stability graph

The stability graph was developed by Mathews et al. (1981) as a tool for guiding bulk mining methods. It is one of the most discussed empirical methods in geomechanics. Suorineni (2010, 2011, 2012) discusses in detail the modifications to the stability graph since its development in 1981. The paper concludes as follows:

(i) As an empirical method, the reliability of the stability graph method is largely dependent on the size, quality, and consistency of the database. Hence, there must be consistency in the determination of the stability graph factors and accepted stope stability state transition zones

(ii) The present tendency for authors to arbitrarily choose between the original and modified stability number factors results in incomparable data that cannot be combined

(iii) The different transition zones produced by different authors result in different interpretations of the stability state of stopes

(iv) There is need for factors that account for stope stand-up time, blast damage, and a gravity factor that is stress-factor dependent

(v) There is a need to develop procedures for determining stability of open stope surfaces that consist of backfill

(vi) The stability graph should be used with caution when applied to narrow vein orebodies because no version of the graphs accounts for orebody thickness in the definitions of the stability states.

In addition to these conclusions, some criticisms of the stability graph emanate from authors who do know the assumptions behind the development of the stability graph but do not know the limits of the database. Suorineni (2010, 2011, 2012) discusses in detail the assumptions behind the development of the stability graph. Obviously, if the underlying assumptions are understood, there need not be criticism about the stress factor not accounting for tension, or the stability graph not being applicable to narrow vein stopes and shallow-dipping stopes. Additionally, while open stope mining is limited to good quality rock masses, the method has been extended to poor quality rock masses, resulting in unacceptable dilutions for which the method has been blamed.

A positive contribution to the stability graph is its quantification by Lunder and Pakalnis (1997). The original and modified stability graphs are qualitative. A stope is stable, unstable, or caved. The miner is keen to know his dilution numbers and whether those numbers are acceptable or not. The equivalent linear overbreak slough (ELOS) stability graph introduced by Lunder and Pakalnis (1997) overcame this deficiency. However, it is still uncertain how orebody size is accommodated in the ELOS stability graph. This has implications for the application of the graph to wide and narrow vein situations.

The extended Mathews stability graph (Trueman and Mawdesley, 2003) also contributed positively to the stability graph by expanding the database from 175 case histories to over 400. However, unfortunately, the authors used the original stability graph factors based on 26 case histories instead of the calibrated factors from 175 case histories. More importantly, not all the case histories are from open stope mining and hard rock environments. Figure 7 shows the composition of the database by mining method.

 

 

The hard-rock empirical pillar design chart

Credit is given to Professor Rimas Pakalnis for his endeavours in promoting and developing empirical methods that are simple, practical, and easy to use by the mining/rock engineer.

The ELOS stability graph, the critical span graph, and pillar design charts are widely used in mining camps and by consultants around the world. Dr Pakalnis continues to serve the industry in more practical issues, remote from the academic quest. 'An academic career in which a person is forced to produce scientific writings in great amounts creates a danger of intellectual superficiality' (Einstein).

It is argued here that mechanistic approaches and the use of numerical modelling have become pervasive in today's geomechanics practice because they are governed by rules (equations) such that presumably they are used without thinking and beautiful computer graphics outputs are all that we need in reports and theses. There is an urgent need for more effort in field data collection to understand the behaviour of the rock mass so that we can effectively and efficiently use our fast machines and complex models to make more reliable predictions.

Admittedly, empiricism alone does not solve all geomechanics problems (neither will mechanistic approaches alone), but the latter must be treated with more caution in geomechanics when used by inexperienced engineers with little or no field exposure.

There are benefits to the use of technology and computers in geomechanics, but these must be guided by reason. Einstein stated that Instead of being a liberating force it (technology) has enslaved men to machines.' (Isaacson, 2007).

The Tunnelling Quality Index: Q-system

The Q-system (Barton et al., 1974) is well detailed in several publications, and the discussion here is limited to what many never venture to look at - the footnotes and assumptions. These apparent oversights have resulted in unwarranted criticisms of the method. Here are some significant points from Barton et al., (1974):

(i) Joint orientation relative to tunnel axis did not appear to be a significant factor because the database is from civil tunnels, which are often placed in the best orientation

(ii) Different personal, national, and continental engineering practices lead inevitably to variations in methods of support, even for the same quality of rock. In mining, regulations will dictate support levels and practice

(iii) Support recommendations in the Q-system are for permanent support

(iv) Support recommendations assume good blasting or excavation practice. For better drilling and blasting or poorer drilling and blasting (deviation from average) the support recommended by the Q-system may tend to be conservative or inadequate respectively. To account for poor blasting practice, adjust Jn and RQD accordingly

(v) The joint set with minimum Jr/Ja should always be used in computing Q.

The Q-system is a major contribution to rock mechanics/geomechanics and Barton et al. (1974) deserve commendation. Today, there is a general feeling that its applications have been too much extended beyond its database limits. QTBM is one such version of extended application. Palmstrom and Broch (2006) provide a critical review of the Q-applications and chronicle its various developments between 1974 and 2002. They advise that potential users of the Q-system, and for that matter any other empirical method, should carefully study the limitations of the system before taking it into use.

What seems to be an implicit problem is the definition of the term 'block size'. In the equation expressing the Q-system:

the following interpretations are assigned to the quotients on the right hand side:

= Block size

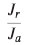= Interblock shear strength

= Measure of active stresses

The block size and interblock shear strength definitions seem to imply that every rock mass is made up of discrete blocks defined by continuous joints. This interpretation implies that the three rock masses shown in Figure 8 will have the same Q value and therefore the same self-supporting capacities. Obviously, this is not the case, either intuitively or in our experience.

 

 

Since most of the initial data came from civil engineering tunnels, it is not surprising that relative orientation was not considered a significant factor for inclusion in the Q-system, since most civil tunnels are aligned in the best orientation for stability. In mining, engineers have no such luxury of choosing the drift alignment in the most stable orientation, but often have to deal with the consequences and the effect of relative orientation of excavations with geologic structures that must be accounted for in stability analysis.

Another implicit problem with the Q-system is the difficulty in applying it to weak rock masses. In weak rock masses the Q-system parameters are difficult to determine and excessive deformation may be the mode of failure rather than structural or brittle failure. This weakness of the Q-system appears not to be obvious to many users of the method. L0set (1999) states:

'The Q-system was primarily suited to hard jointed rocks ... but for the classes of poorest rock quality the system has not provided detailed description of the support constructions. This means that for weak rock masses the dimensioning of the support must usually be verified by numerical modelling or some other means of calculation.'

Løset (1999) identify six types of weak rock masses as follows:

(i) Heavily jointed strong rocks (Q<0.01)

(ii) Zones with altered or strong rock (squeezing or swelling may take place)

(iii) Weak rocks with joints (young sedimentary rocks (sandstone) or weakly metamorphic rocks such as shale, slate, or phyllites)

(iv) Weak rocks with excavation-induced fractures (e.g. young homogeneous sedimentary rocks (chalk, sandstone) or low-grade metamorphic rocks (shale, phyllites)

(v) Weak rocks without joints or induced excavation fractures (young homogeneous sedimentary rocks such as chalk, sandstone, and mudstone)

(vi) Weathered rocks.

The Hoek-Brown failure criterion

The Hoek and Brown failure criterion (Equation [3]) has dominated the rock mechanics/geomechanics world in terms of use and acceptability.

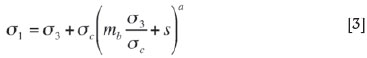
where σ₁ and σ₃ are the effective major and minor principal stresses at failure respectively, σ_c is the intact rock uniaxial compressive strength, and a, m_b, and s are constants that depend upon the characteristics of the rock mass.

Hoek and Marinos (2007) provide a summary of the various modifications to the Hoek and Brown failure criterion and Geological Strength Index (GSI) (Hoek et al. 1995) from 1980 to 2006. The most recent version of the Hoek and Brown failure criterion is given in Hoek et al. (2002). In this update, a new parameter referred to as the rock mass disturbance factor (D) is introduced to deal with blast damage and other disturbances. This factor allows for the determination of more appropriate Hoek-Brown parameters depending on the degree of disturbance inflicted on the rock mass by the excavation method.

The Hoek-Brown failure criterion is based on the assumption that a jointed rock mass is fundamentally weaker in shear than intact rock (Diederichs et al., 2004). Although the Hoek-Brown failure criterion has been widely accepted, it is not free from criticism. Following these criticisms, various authors including (Martin, 1994; Pelli et al., 1991; Diederichs et al., 2004; Carter et al., 2008) have offered some modifications to the criterion, claiming that it is not universally applicable to the full range of rock mass qualities as defined by the GSI.

In 1994 Hoek, in a letter to editor of International Society for Rock Mechanics (ISRM) News Journal, wrote:

'In writing Underground Excavations in Rock 15 years ago Professor E T Brown and I developed the Hoek-Brown failure criterion to fill a vacuum which we saw in the process of designing underground excavations. Our approach was entirely empirical and we workedfrom very limited data of rather poor quality. Our empirical criterion and our estimates of the input parameters were offered as a temporary solution to an urgent problem.

'The fact that the criterion works, more by goodfortune than because of its inherent scientific merits, is no excuse for the current lack of effort or even apparent desire to find a better way:

What is surprising is that a flawed 'temporary solution' has become a permanent solution. As further alluded to by the authors, instead of engineers of today going back to the fundamentals to develop a better failure criterion based on a good understanding of geology and 'physics', more time is spent criticizing the criterion - sometimes without suggesting solutions. Nor is any effort made to collect field data to back up any criticisms or suggested modifications.

More time is spent on computer modelling, with little fieldwork to validate such models. The input parameters to these models mostly cannot be justified, as the current generation of engineers does not understand the significant role of geology and the resulting uncertainties governing the input parameters and failure criteria. Hence it is not surprising to come across research results like those shown in Figure 9. Ironically, after knowing how the tunnel Figure 9 failed, we are then able to capture the failure (as in Hadjiabdolmajid 2001; Carter et al., 2008) after manipulating the input parameters and failure criterion.

 

 

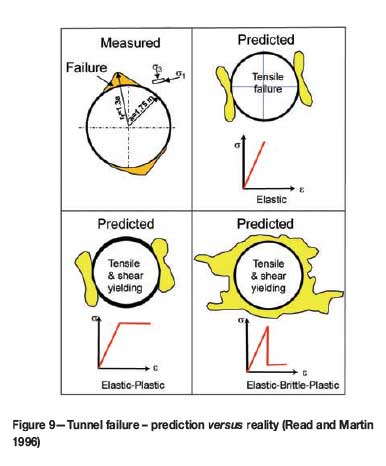
Thus in most cases we are able to correctly predict the behaviour of excavations in our numerical models only after the fact, by manipulating input parameters to match observations under the cover of so-called 'back-analysis' or 'model calibration'. While we will most often satisfy ourselves this way in our offices, the rock mass is so idiosyncratic that in our next project it will defy our prediction and behave again in its own way.

 

Challenges of our time

Our predecessors made efforts to solve the problems of their time. Today we are faced with challenges beyond the solutions of our predecessors. Those solutions have reached their limits. The challenges in our time include development of failure criteria suitable for rock, guidelines for optimized block cave mine design based on a deep understanding of caving mechanics, predicting rockbursts, and seeing through the in situ rockmass.

We need ways to see inside the block cave and to resolve issues like unexpected ground conditions. The prospect of rockburst prediction still remains remote and all we can do is monitor, mitigate, and identify potential high-risk areas. We are also faced with the issue of reducing in situ stress measurement errors to acceptable levels. Excavation face fatalities continue to occur.

Recent events in the mining industry, including the Northparkes airblast fatalities, Grasberg mine disaster, Beaconsfield fatalities, and fatalities in mines in the Sudbury Basin, have exposed the limits of our current knowledge. The reports from these investigations mostly concluded that the circumstances leading the incidents could not have been foreseen. Our inability to foresee such circumstances indicates the limitations of our current knowledge. We need technology to see behind total cover surface support systems such as shotcrete and thin spray-on liners (TSLs). Ultimately, we need technology to see behind the excavation face and to monitor the inside of block caves in real time. We need technology to predict and mitigate rockbursts. The phrase 'unexpected ground conditions' is an example of an excuse that stifles the urgent need for technology required to overcome our limitations.

There are other valuable applications of technology in the mining industry. This is captured by Peterson et al. (2001), who states: 'It is the knowledge management benefits of new IT technology that will provide the greatest benefit to the industry (Mining). Although mine operations are generating more data, such information is rarely well utilized.'

To bridge and expand our knowledge to cope with current challenges require new thinking. Lessons learnt from science and medicine indicate the path to developing solutions in geomechanics through core rather than peripheral research. These are discussed next.

 

Lessons from medicine

The field of medicine faces serious challenges at any one time. However, these challenges are often met with enduring efforts by the medical community to understand their origins and develop the appropriate technologies to offset their impact. Until recently these efforts have been generally individualistic with various experts working in 'protective silos'.

While the silo approach to research in medicine produced results, it often took several decades and generous funding to produce significant results and or breakthroughs. A good example is the race for a cancer cure. For several decades, the search has been carried out by individuals working in silos. Each individual has been an 'authority' in his own right. This approach has not resulted in any major or significant breakthroughs.

The difficulty in curing cancer lies in the fact that it is not just one disease. Cancer has potentially thousands of causes, and not all cancers are caused by just one agent. Hence, it is now recognized the challenge to unravel the cancer myth and find a cure, cannot be achieved through the 'silo' research approach.

The Stand Up to Cancer (SU₂C) organization (Park, 2013) has brought a paradigm shift to medical research by bringing science and medicine together, fostering genuine collaborative core research by breaking down individual expert research silos, and providing generous funding to collaborative core research groups.

 

 

With this strategy, individual experts working in defensive silos in secrecy on various aspects of cancer are brought together to form multidisciplinary collaborative core (not peripheral) research groups in which egoistic barriers are broken and former competitors are working together. This approach brings the best talents together and is seen to lead to significant medical breakthroughs in relatively short timeframes.

Park (2013) notes that multidisciplinary collaborative breakthroughs in medicine are enhanced and accelerated by development and use of relevant technology. Technological advances in medicine have come from bioengineering, nanotechnology, new drug compounds, data gathering, and cheaper and more powerful computers. Collaboration results in strength in numbers, and combined with technology, leads to dazzling scientific and research advances.

 

Lesson from science

Like the lessons in medicine, breakthrough in science in recent times is also a result of technology and genuine collaboration.

The recent discovery of the Higgs boson, the so-called 'God particle' which had eluded physicists for nearly five decades since its existence was proposed, was a result of genuine multidisciplinary collaboration and availability of the relevant technology backed by generous funding. The A Toroidal Large Hadron Collider (LHC) Apparatus (ATLAS) Collaborations (2012) reports that in this project nearly 2000 physicists from US institutions (89 universities and seven Department of Energy laboratories) participated in the ATLAS and Compact Muon Solenoid (CMS) experiments, making up about 23 per cent of the ATLAS collaboration and 33 percent of CMS at the time of the Higgs discovery. The LHC apparatus cost US$10 billion.

The LHC is the enabling technology in the project that was built by the Centre Europeen de Recherche Nucleaire (CERN) particle physics laboratory on the Swiss-French border. This was a multinational genuine collaborative research project.

The prediction of the existence of Higgs boson as part of the Standard Model (SM) in 1964 and its eventual discovery (proof) through experiments was a demonstration of the strength of genuine multidisciplinary collaboration backed by persistence and generous funding. The SM explains the prevailing theory that describes the basic constituents of matter and the fundamental forces by which they interact (Veltman, 1986) and is the most successful explanation of the universe to date.

 

Model for progress in geomechanics

If physics were geomechanics, the Higgs boson would never have been predicted, much less discovered. It is also frustrating that, unlike in medicine, silo rather than genuine collaborative research persists in geomechanics. Research silos exist and thrive in geomechanics and academia for the following reasons:

(i) Who owns the credit for what is achieved?

(ii) Who owns the intellectual property?

(iii) Who is the lead author of the paper?

(iv) How many papers can I publish?

(v) How much of the money can I get?

(vi) I should be better than all others

The collaboration of the best brains, independent of initial individual differences, leading to major breakthroughs in geomechanics is still decades away. Muller (1988) notes that no doubt much goodwill and intimate collaboration is required to translate into reality the synthesis of rock mechanics and engineering geology. Terzaghi had a similar ambition, that of a synthesis between soil mechanics and engineering geology. To the contrary, Muller notes:

'Unfortunately collaboration is rare between human beings and is still more rare between specialists. I consider the lack of real and through going collaboration one of our daily problems. Many failures, waste of many and even disastrous events and loss of life I have experienced by this reason:

Muller's statement, made in 1988, still hold true in geomechanics and among rock engineers, engineering geologists, and geologists. It is sad to note that there is no two-way communication between ground control engineers and geologists in our mining camps. Such constant communication could alleviate most of the fatalities on record.

The inconvenient truth is that rock is the most complicated material to deal with compared to soil, concrete, or steel. To control or manage this complicated material we have to understand it. Neither the geologists, rock engineers, nor engineering geologists have sufficient knowledge individually to understand rock behaviour. Understanding rock behaviour requires genuine multidisciplinary collaboration between the best brains in geoscience and engineering, independent of personal differences, coupled with development or adoption of the appropriate technology and generous funding.

The challenges facing geomechanics practice today can be solved through the following model adopted from the experiences in science and medicine:

'Bring the best and most talented possible brains from multidisciplines in earth science and engineering together independent of personal differences, fund them generously, oversee their progress rigorously in a tight schedule, and they will unravel the challenges in geomechanics:

The multidisciplinary collaboration should include the identification, adoption, and development of appropriate technologies for seeing through the rock mass in a manner similar to the way in which medical CT scanners can see through the human body to diagnose ailments. We need a 'transparent rock mass'.

Rockbursts are the 'cancer' in geomechanics. The phenomenon of rockbursts has been studied for over a century, and yet the causes remain poorly understood and the prospect of being able to predict them remains remote. Salamon, in 1983, stated 'A disconcerting feature of rockbursts is that they defy conventional explanation.'

This statement remains true today. We could solve rockbursts and prevent associated fatalities in our underground mines through genuine multidisciplinary collaboration and generous funding. We need to stand -up to rockbursts (SU₂R), just as the medical scientists are standing up to cancer (SU₂C).

We can remove workers from the work face by developing and adopting appropriate technologies. Technology exists for doing this. If the National Aeronautics and Space Administration (NASA) can send robots to explore Mars, there is no excuse for the mining industry's inability to remove workers from the excavation face and mine remotely. Unmanned robots such as the 'Rover' could drill, charge, blast, muck, and support. Steve Perry and Larry Knight would be alive today.

Current proposed technologies for mining seem to focus on peripheral issues rather than core issues, which if tackled successfully would bring about enormous benefits in terms of safety and productivity. Evidence exists (Figure 9) that step changes in technology result in equivalent increases in mining productivity.

A suitable failure criterion for rocks remains elusive, while the determination of rock mass properties remains a challenge. Our inability to overcome the myth of 'unexpected ground conditions' remains. The ability to see behind total cover surface support systems (such as shotcrete and TSLs remains daunting. Errors in in situ stress measurements continue to be unacceptable, and are continuously becoming worse as we mine at deeper levels. Hoek (1994) states:

'Techniques for measuring in situ stress while greatly improvedfrom what they were still give an amount of scatter which would be unacceptable in almost any other branch of engineering:

The solutions to these problems require a multidisciplinary genuine collaborative research in geoscience and engineering, coupled with the development and adoption of appropriate technologies.

 

Conclusions and recommendations

Our predecessors developed solutions to the problems of their time that we continue to use, albeit with mixed results. As our mines continue to go deeper, so do the solutions of our predecessors continue to become less adequate in terms of their predictive abilities.

Increasing computing power is not accompanied by a similar ability to collect and determine appropriate rock properties for our powerful and complex numerical modelling codes. Indeed, field work and laboratory investigations are now being replaced with computer simulations and laboratories are shutting down. We need to reverse course, as computer simulations need realistic inputs to be valid.

To control and manage structures in rock, we need to understand rock. Geology is the pathway to understanding rock. Geology should be emphasized in mining and civil engineering programmes.

Experience in science and medicine shows that the problems in geomechanics can be overcome through genuine interdisciplinary collaboration, generous funding, and development of appropriate technologies. The future of safe and productive mining lies in the development/adoption of relevant technologies that can assist our understanding of rock behaviour and remove man from the excavation face.

 

References

Barton, N., Lien, R., and Lunde, J. 1974. Engineering classification of rock masses for the design of rock support. Rock Mechanics, vol. 6, no. 4. pp. 189-236.         [ Links ]

Bieniawski, Z.T. 1973. Engineering classification of jointed rock masses. Transactions of the South African Institute of Civil Engineers, vol. 15. pp. 335-344.         [ Links ]

Bieniawski, Z.T. 1989. Engineering Rock Mass Classifications. Wiley, New York.         [ Links ]

Brown, E.T. (ed.). 1981. ISRM Suggested Methods for Rock Characterization, Testing and Monitoring. Pergamon, Oxford.         [ Links ]

Carter, T.G., Diederichs, M.S., and Carvalho, J.L. 2008 Application of modified Hoek-Brown Transition Relationships for assessing strength and post-yield behaviour at both ends of the rock competence scale. Proceedings of the 6th International Symposium on Ground Support in Mining and Civil Engineering Construction, Cape Town, South Africa, 30h March - 3 April 2008. Southern African Institute of Mining and Metallurgy, Johannesburg. pp. 325-338.         [ Links ]

Clark, L.M. and Pakalnis, R.C. 1997. An empirical design approach for estimating unplanned dilution from open stope hangingwalls and footwalls. Proceedings of the 99th Annual General Meeting, Vancouver, Canada. 27 April - 1 May 1997. Canadian Institute of Mining, Metallurgy and Petroleum.         [ Links ]

Craig, R.F. 1982. Soil Mechanics. 2nd edn. Van Nostrand Reinhold, London.         [ Links ]

Diederichs, M.S., Kaiser, P.K., and Eberhardt, E. 2004. Damage initiation and propagation in hard rock during tunnelling and the influence of near-face stress rotation. International Journal of Rock Mechanics and Mining Sciences, vol. 41. pp. 785-812.         [ Links ]

Dyke, G.P. 2008. Rock mass characterization: a comparison of the MRMR and IRMR classification systems. Journal of the Southern African Institute of Mining and Metallurgy, vol. 108. pp. 657-659.         [ Links ]

Flores, G. and Karzulovic, A. 2003. Geotechnical guidelines for a transition from open pit to underground mining. Report to the International Caving Study Stage II. University of Queensland, Brisbane.         [ Links ]

Franklin, J.A. 1993. Empirical design and rock mass characterisation. Comprehensive Rock Engineering. Hudson, J.A. (ed.). Pergamon, New York. vol. 2, pp. 759-806.         [ Links ]

Hajiabdolmajid, V., Martin, C.D., and Kaiser, P.K. 2000. Modelling brittle failure. Proceedings of the 4th North American Rock Mechanics Symposium, Seattle, Washington. 31 July - 3 August 2000. American Rock Mechanics Association, Alexandria, Virginia. pp. 991-998.         [ Links ]

Hajiabdolmajid, V.R. 2001. Mobilization of strength in brittle failure of rock, PhD thesis, Department of Mining Engineering, Queen's University, Kingston. 268 pp.         [ Links ]

Hoek, E. and Brown, E.T. Underground Excavation in Rock. Institution of Mining and Metallurgy, London.         [ Links ]

Hoek, E. and Marinos, P. 2007. A brief history of the development of the Hoek- Brown failure criterion. Soils and Rocks, no. 2. pp. 2-13.         [ Links ]

Hoek, E., Carranza Torres, C.T., and Corkum, B. 2002. Hoek-Brown failure criterion: 2002 edition. Proceedings of the 5th North American Rock Mechanics Symposium.. Toronto, Canada, 7-10 July 2002. pp. 267-274.         [ Links ]

Hoek, E., Kaiser, P.K., and Bawden, W.F. 1995. Support of Underground Excavations in Hard Rock. A.A. Balkema, Rotterdam.         [ Links ]

Isaacson, W. 2007. Einstein: His Life and Universe. Simon and Schuster, New York.         [ Links ]

Laubscher, D.H. 1994. Cave mining-the state of the art. Journal of the South African Institute of Mining and Metallurgy, vol. 94, no. 10. pp. 279-293.         [ Links ]

Laubscher, D.H. and Taylor, H.W. The importance of geomechanics of jointed rock masses in mining operations. Proceedings of the Symposium on Explorationfor Rock Engineering, Cape Town, South Africa. 1-5 November 1976, A.A. Balkema, Cape Town. pp. 119-128.         [ Links ]

Løset, F. 1999. Use of the Q-system in weak rock masses, NGI report no. 592048-1. NGI, Oslo, Norway.         [ Links ]

Lunder, J. and Pakalnis, R. 1997. Determining the strength of hard rock mine pillars. CIM Bulletin, vol. 90, no. 1013. pp. 51-55.         [ Links ]

Mathews, K.E., Hoek, E., Wyllie, D.C., and Stewart, S.B.V. 1981. Prediction of stable excavation spans at depths below 1000m in hard rock mines. CANMET Report, DSS Serial No. OSQ80-00081. 127 ppLinks ] Arial, Helvetica, sans-serif" size="2">.

Milne, D., Hadjigeorgiou, J., and Pakalnis, R. 1998. Rock mass characterization for underground hard rock mines. Tunnelling and Underground Space Technology, vol. 13, no .4. pp. 383-391.         [ Links ]

Mohr, O. 1900. Welche Umstande bedingen die Elastizitatsgrenze und den Bruch eines Materials? Zeit. Ver Deut. Ing, vol. 44. pp. 1524-1530Links ] Arial, Helvetica, sans-serif" size="2">.

Müller, L. 1966. Der progressive bruch in geklufteten median. Proceedings of the First Congress of the International. Society of Rock Mechanics, Lisbon, Portugal, 25 September - 1 October 1966. ISRM. pp. 679-686.         [ Links ]

Müller, L. 1988. The influence of engineering geology and rock mechanics in tunnelling. Bulletin of the International Association of Engineering Geologists, vol. 38. pp. 5-13.         [ Links ]

Park, A. 2013. The hero scientist who defeats cancer will likely never exist. The Times, 1 April 1 2013. pp. 32-38.         [ Links ]

Pelli, F., Kaiser, P.K., and Morgenstern, N.R. 1991. An interpretation of ground movements recorded during construction of the Donkin-Morien tunnel. Canadian Geotechnical Journal, vol. 28, no. 2. pp. 239-254.         [ Links ]

Pells, P.J.N. 2008. What happened to the mechanics in rock mechanics and the geology in engineering geology? Journal of the Southern African Institute of Mining and Metallurgy, vol. 108. pp. 309-323.         [ Links ]

Peterson, D.J., Latourrtette, T., and Bartis, J.T. 2001. New forces at work in mining. Industry Views of Critical Technologies. RAND, Science and Technology Policy Institute, Santa Monica, California. pp. 33-52.         [ Links ]

Read, R.S. and Martin, C.D. 1996. Technical summary of AECL's Mine-by Experiment. Phase1 excavation response. Atomic Energy of Canada Limited Report, AECL-11311. 169 pp.         [ Links ]

Salamon, M.D.G. 1983. Rockburst hazard and the fight for its alleviation in South African gold mines. Proceedings of the Conference on Rockbursts, Prediction and Control, 20 October 1983. Institute of Mining and Metallurgy, London. pp. 11-52.         [ Links ]

Suorineni, F.T. 2010. The stability graph after three decades in use - experiences and the way forward. International Journal of Mining, Reclamation and Environment, vol. 24, no. 4. pp. 307-3392.         [ Links ]

Suorineni, F.T. 2011. Factors influencing overbreak in the Barkers orebody, Kundana Gold mine: narrow vein case study. Paper by: P.C. Stewart, R. Trueman and I. Brunton, 2011: Mining Technology, vol. 120 (2), pp. 80-89. Letter to the Editor, Transactions of the Institution of Mining and Metallurgy Section A): Mining Technology.         [ Links ]

Suorineni, F.T. 2012. A critical review of the stability graph method for open stope design. MassMin 2012, Sudbury, Ontario.         [ Links ]

Suorineni, F.T. 2013a. Geomechanics challenges and its future direction - Food for thought. Ghana Mining Journal, vol. 14. pp. 14-20.         [ Links ]

Suorineni, F.T. 2013b. The geomechanics challenges of contemporary deep mining: technology as the pathway to increased safety and productivity. 16th Kenneth Finlay Lecture, University of New South Wales, Australia.         [ Links ]

Trueman, R. and Mawdesley, C. 2003. Predicting cave initiation and propagation. CIMBulletin, vol. 96. pp. 54-59.         [ Links ]

Veltman, M.J.G. 1986. The Higgs Boson. Scientific American, November 1986. pp. 76-84.         [ Links ]