ORIGINAL RESEARCH

 

Children's development of an understanding of number: A model for Grade R teachers

 

 

Lerato B. Ndabezitha

Department of Childhood Education, Faculty of Education, University of Johannesburg, Johannesburg, South Africa

Correspondence

 

 

---

ABSTRACT

BACKGROUND: Teachers teach mathematics by focusing on procedural knowledge (what steps to follow when doing mathematics) and pay scant attention to the conceptual understanding that underlies these procedures such as double-digit addition or subtraction and overall additive and multiplicative relations. They teach concepts in a haphazard way instead of looking at them from a convergent perspective of concepts that a child builds, one after the other
AIM: This study explores what Grade R teachers know about young children's specific developmental numerical cognition and if they infuse their understanding of number concept development in their pedagogy
SETTING: Samples of 15 female Grade R teachers were purposefully selected from five schools in what was previously a racially segregated living area in South Africa
METHODS: This research design is a descriptive case study. Data were collected using semi-structured interviews and observation notes documented during workshops and when the teachers implemented their learnings in their classrooms
RESULTS: The main finding was that although teachers could reflect on the model of number concept development they had learned, they found it hard to infuse their knowledge into a strictly structured curriculum
CONCLUSION: Despite the Meerkat Maths programme offered to the teachers, they could not rely on their intuitive pedagogy, coupled with the model of number concept development that they had learned because of the strict demands of the school curriculum content with which they had to comply

Keywords: Grade R; number concept development; pedagogical content knowledge; numerical cognition; mathematics; teacher knowledge.

---

 

 

Introduction

South African schools are faced with an alarming challenge, which is evident from evaluations that were conducted by the Department of Basic Education (2022) as part of the literacy and numeracy strategy from 2006 to 2016. For example, the results in 2009 indicated that only 35% of the learners in Grade 3 were competent in performing typical tasks in mathematics. Dlamini (under review) studied two Grade 3 classes in a Johannesburg school recently and found that learners could perform basic calculation tasks but continued to rely on unit counting and struggled to read word problems. In addition, the Department of Basic Education presented findings from Trends in International Mathematics and Science Study 2019 (TIMSS) comparing South African learners with the rest of the world. The results revealed that South African learners scored in the bottom three countries (Writer 2020).

Bezuidenhout (2022) discussed results of 130 learners in five Johannesburg schools where the findings also show that children do not progress well in mathematics at the beginning of their school career. A more recent research study, mentioned in The Reading Panel (2022), shows that the COVID-19 pandemic, with the loss of learning time, had a severe effect on a sample of children who were assessed in the Limpopo province. Much of early grade mathematics depends on an understanding of number, which predicts later attainment in mathematics (DeSoete 2015). The research findings by the Research triangle institute (RTI) (https://www.rti.org/) in early grades learning in Kenya showed that along with early literacy competence, early mathematics is an important predictor of later performance (Piper 2022).

Apart from salient findings, it has long been our experience in working with teachers on the ground in five partner schools of our university that teachers find it hard to adapt their teaching of early numeracy for various reasons; one of these is that they teach number facts and procedures for simple calculations, with little thought given to the concepts of numeracy (Bezuidenhout 2020, in press; Kortjass et al. 2021; Ndabezitha 2018; Ntsoane 2018; Simelane 2018).

Yet, much of the mathematics learning in the early grades involve number concept knowledge as foundation for calculation. The pedagogy of numeracy requires that the teachers know how number concepts develop in children of this age group (Clements & Sarama 2009, 2015). Fritz et al. (2013) have argued that the Grade 1 curriculum in South Africa requires at the very least an understanding of number cardinality. This level of understanding comes with some instruction and is developmentally dependent on how children count and how they sequence and how they use the natural language numerals for quantities (Dowker & Nuerk 2016).

Generally, from the author's experience in the five partner schools, as well as the primary school on our campus, which is affiliated to the teacher education programme of the university, it has been consistently observed that teachers do not invoke knowledge of numerical cognition in their teaching. In informal discussions with teachers during workshops, the discourse of number pedagogy has been mostly limited to words that refer to mathematical notations, such as +, −, =, ×, ÷ or to Arabic number symbols. In referring to, for example, +, they use the term 'plus' as a verb, as a noun or in the gerund ('plussing'). In most instances, the children regard the signage itself as the primary semiotic tool, which is preferable to natural language. This may well be why they find the cohesive reading of word problems a greater challenge than the number notation system problems (Dlamini [under review]) and why they also struggle with reading science texts across sentences (Dlamini [under review]).

It is for these reasons that studies on teachers' knowledge of numerical cognition are important, especially how they apply their understanding of numeracy concepts of young children to their teaching of number. In the study reported in this article, the author wanted to find out what teachers know about a specific developmental numerical cognition of young children and if they infuse their understanding of number concept development in their pedagogy. Thus, the author wanted to look for a connection of their 'content knowledge' (developmental numerical cognition) and their pedagogy - their pedagogical content knowledge (PCK) (Shulman 1986) of teaching number to young children. The author started the research with a question: what do Grade R teachers know about developmental mathematics - specifically numerical cognition? The author translated the question into isiZulu for my own clarity. The author found this type of translation useful: Yini abayaziyo othisha bebanga likaGrade R ngezibalo ezithuthukayo - ikakhulukazi ukuqonda izinombolo?

Number concepts in early childhood

The argument for this article is that if teachers know more about how children's mathematical concepts (including number concepts) develop, they are likely to include that in their pedagogy and teach with an understanding of how they should approach children's learning; they would take cognisance of the developmental cognitive psychology features of learning about number. The argument from the perspective of a specific teacher PCK is: children's early number concepts develop, step by step and hierarchically.

Fritz, Ehlert and Balzer (2013) developed a theoretical model according to which number concepts develop in this way at conceptual levels, with calculation skills learned in tandem. However, as the concepts become increasingly more abstract (see Figure 1), they sometimes revert to an earlier level, such as doing unit counting when they add and when they subtract numbers. It is furthermore suggested by these authors that for learners to move from one level of conceptual understanding to another, they need stable knowledge at one level, which then leads to another, higher level as building blocks (Clements & Sarama 2009) or stepping stones (Fritz et al. 2013) for further conceptual development. The conceptual change they refer to has to do with the increase in numerical understanding from (1) the one-to-one correspondence in counting of objects, to (2), the sequence or order of numbers, then (3), the cardinality of a number, followed by (4), the part-part-whole composition of any number and (5), the relationship of numbers with one another - specifically how their value changes from one whole number to the next, which is always by one.

 

 

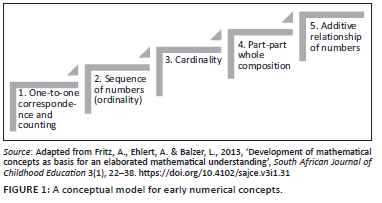
Fritz et al. (2013) described the levels of development as follows (See also Henning et al. 2021:2-4):

• Level 1 indicates how children count orally, enabling them to recite the words for numbers. However, mental representation does not yet exist; hence, they just recite the 'counting list' and begin to count material objects one by one. Gradually, they begin to grasp the one-to-one correspondence of objects that are being counted.

• Level 2 describes how children begin to understand that numbers come in sequence in a type of mental number line (Dehaene 2011). For example, they can distinguish numbers 'before' and 'after', each other, but they do not differentiate yet between 'more' and 'fewer' in the linear numerical presentation.

• Level 3 competence indicates that they understand that a number is a composite unit, and therefore, it can be decomposed. This is the level of development at which children can count out objects and know the sum.

• Level 4 describes the competence of recognising that a number consists of two or more parts and a whole; this mental competence is known as the 'part-part whole' concept. Numbers consist of different parts that make up the whole.

• Level 5 of the model of concept development shows the relationship between congruent units on the number line.

Gallistel (1999) referred to 'cognitive structures' that are formed neuronally. These structures have strengths and weaknesses that depend on how the concepts have been introduced into children. According to Dehaene (2011), children's encounters with instruction in early numeracy are crucial for how the young learners build their 'cognitive structures' and advance their sense of number. When the author set out to conduct the research, it was uppermost in her mind that she should try to understand what teachers think about their teaching of numeracy, especially after they had been introduced into a variety of pedagogies that relate to teaching numerical concepts along with procedures. The author was keen to find how teachers reflect on and analyse children's arithmetical competence according to the Fritz et al. (2013) model to which they had been introduced.

One of the studies encountered in the author's reading was Fuson (1988), who proposed that there are several abilities involved when analysing children's arithmetic abilities. She explains that there are abilities that are connected to number sequence, counting, cardinal procedures and solution procedures. Number sequence, she proposes, is the ability to count forward or backward. Counting can be assessed by ensuring one-to-one correspondence by the ability to point at one object at a time, corresponding with saying number words and keeping track of which objects have been counted and which have not. Fuson (1988), like Fritz et al. (2013), also explained that cardinal number competence means being able to break numbers into smaller components and vice versa. Solution procedures indicate the ability to count from smallest to largest - forward and backward - to solve different kinds of problems. These ideas are some of the theoretical work that Fritz et al. (2013) utilised to formulate a model, which they then validated empirically with children (n = 1200+).

Another researcher that is cited by Fritz et al. (2013) is Wynn (1990, 1992), who performed the famous 'give men' objects experiment, by asking young children who had not yet developed the principle of cardinality and also had yet not developed the one-to-one correspondence principle, to give her one object, then two, then more. Most children could not grasp the quantity of two and just gave her a handful of objects. Older children (3-6 years) could subitize and give the correct number of objects without counting. Wynn (1992) commented that at the time of her early research, the discussions in maths education were ongoing after Gelman and Gallistel's (eds. 1986) ground-breaking research on children's number knowledge. She notes that number concepts have to connect with language:

The problem that children must solve, then, is that of mapping these number concepts onto words. In this, children are faced with the problems inherent to any word-learning task-from an infinity of logically possible meanings, they must somehow infer the correct meaning of a word. This is made more difficult for children by the fact that the number words do not refer to individual items, or to properties of individual items, but rather to properties of sets of items. (Wynn 1992:221)

Carey (2009), a cognitive scientist, suggested that children cannot be expected to know how to (truly) count without knowing what the number name means. When children learn or mimic number names without understanding, it creates a semantic gap because there is no connection between the digits of the number symbol and the number name. She explicitly articulates how human beings acquire concepts. Although the innate number knowledge that humans have is a building block that captures concepts, it is through language that the number concept acquires a semantic representation in the brain. Before children acquire language, they have different mental representations of mathematical concepts. They have an unarticulated 'number sense' (Dehaene 2011). For example, they are able to see that there are three birds on a tree and one flew away, and that there are then two. They are able to differentiate quantity, even though they have not understood the one-to-one correspondence. Their 'ancestral' approximate number sense is activated.

When the idea was first discussed, the teachers were surprised. It had not been part of their practice to reflect on innate knowledge or on developmental matters of numeracy.

Teachers' knowledge

As mentioned in the first section of this article, the theoretical framework for this study is the hierarchical model of number concept development for children in the age group of 4-8 years (Fritz et al. 2013). Admittedly, this model alone does not give the teachers all they need for their practice. Their general pedagogical knowledge of classroom practice and their sense of how to differentiate in their teaching and so forth are not discussed in this article.

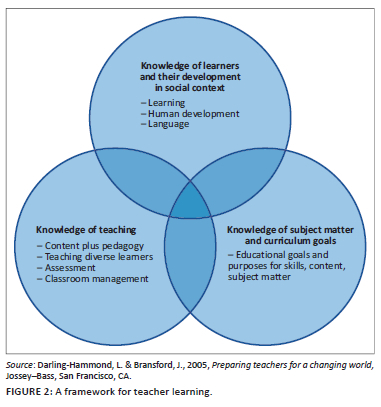
However, in terms of teacher knowledge, Darling-Hammond and Bransford (2005:19) is included as an overarching 'template' for what is regarded as basics in teachers' knowledge. In their leading handbook on the preparation of teachers 'for a changing world', they accentuate knowledge about the learner as a primary variable in the model of teaching that they suggest as a conceptual framework for teacher education. From this view, the author could further argue that as much as the teacher needs to know mathematics and the pedagogy of mathematics, she also needs to know how to judge a child's learning and development. For that, teachers need to understand young children's mathematical cognitive development. This view is expressed by Darling-Hammond and Bransford (2005:11) in their study of how people learn, derived from earlier work by Bransford, Brown and Cocking (1999).

In the Venn diagram shown in Figure 2, 'knowledge of learners and their development in social context' suggests that teachers should have ample knowledge of child development. For the purpose of the data of this study, the 'knowledge of learners' included only children's number concept development as a cognitive foundation. The 'social context', was, however, an aspect that teachers referred to in the interviews and which was also observed during classroom observations. An example of a 'social context' observed and referred by the teachers is that learners come from low-income families, and these learners are at risk of poor school performance. Nevertheless, there will not be much focus on this in the discussion of the data.

 

 

Generally, early grades teachers in South Africa are not au fait with cognitive science (Henning 2016). It is seldom part of their preservice education. For the teachers in this study, the introduction to one component of children's cognitive development came as a surprise. The programme of teacher development in which the author participated as a practitioner researcher became known as the Meerkat Maths programme (see Figure 3) (Herzog, Fritz & Jansen van Vuuren 2018; Van Vuuren, Herzog & Fritz 2021). It has since been implemented in several teacher development programmes. The name of the programme was derived from the figures that illustrated the MARKO-D SA diagnostic test for early number concept development (Henning et al. 2020, 2021).

 

 

Methods

The sample of participants (n = 15) was selected as an intact group of Grade R teachers/practitioners who participated in weekly teacher development workshops during one semester. Thus, the selection was purposeful (Creswell 2014; Merriam 2009) because the teachers who took part in the development were from the five partner schools. During the weekly sessions, the teachers were observed in the training workshops by the director of the local university research centre and the author. Recordings were made of the last two sessions. Prior to that fieldnotes were made to capture the essence of the sessions. All the teachers were interviewed in isiZulu or English. The interviews (following Kvale 1983) were transcribed and translated where needed by the researcher. Teachers were subsequently also observed in two lessons during the semester workshops. These lessons were video-recorded and transcribed.

The analysis of the transcribed texts was conducted largely inductively, following the units of meaning as they were identified by the primary researcher, who then coded them (Charmaz 2002, 2007; Henning, Van Rensburg & Smit 2004; Strauss & Corbin 1999). The units of meaning were related to the main research question. However, deductive coding occurred when elements of the conceptual model of number concept development were identified. The classroom observation notes and transcriptions were analysed in a similar fashion. After the coding of the data, the codes were scrutinised and two researchers categorised the codes to form categories and to construct a thematically based set of findings. The processes of data capturing and of the analysis of the data were recorded throughout.

Ethical considerations

In order to address ethics in this research, the author worked in accordance with the prescribed set of procedures of the university. The specific measures were as follows: (1) applying for overall ethical approval via faculty processes, (2) requesting informed consent from participants to be part of the research, (3) as part of informed consent, the ethics procedure was explained to participants prior to interviewing them and also coming for observations in their classrooms, and it was stated that the data will only be used for the purpose of this study and will be reflected in a research report, which may be viewed by others and (4) that the findings will not expose the identities of the participants'. The participants' interviews and observations were treated with a strong measure of confidentiality and information obtained was discussed only with the author's research supervisors who are also cognisant of ethical measures.

 

Results

The findings of this study reveal that the teachers were not only attentive in the workshops but also aware of child development, in general, and specifically of number concept development. An example of one set of codes (see Figure 4) illustrates this finding. It was, firstly, evident that the teachers were wary of the very strictly scripted national curriculum, which, according to them, is fast paced and was written by:

'[P]eople who don't know what it is like in the classroom. Youcan't move from one concept to the next just like that.' (Teacher 4, female, Rita [is the pseudonym])

Furthermore, the general discourse in the interviews showed that the teachers had begun using terminology of number concept development with insights and in a critical manner. Teachers also spoke about the importance of the development of mathematical terminology at home, explaining, often with detailed examples, how children make their world mathematical. One of the participants (Teacher 9: female: Lina [is the pseudonym]) said:

'Let's say one stays at Quthu. The father and the mother they are here in Johannesburg. Then the granny, … perhaps the granny might say a number accidentally. Like saying there are four of us. Then the child will wonder what is that four that they are talking about. If all the households were the same where learners come from urban areas it would have been much [easier]. So there in rural areas it's not good. When the granny dies they bring the child here in Joburg. Did the child learn mathematics?'

The same teacher commented:

'It's everyday life whether I like it or not - numbers are there. So eeeh that's why I said at a Grade R level at home we must be accustomed to the culture of making our children talk verbally.'

This teacher, like most others, appreciated the sessions about seriation and categorisation very much and used the materials, with the Meerkat characters and their environment effectively, and also joyfully:

'[E]eeeh the best thing about the Meerkat Math programme at XX is that you don't have to follow one method or one option to do mathematics because it was one story, which was turned up to cover all these concepts of mathematics. What we know as teachers is that you just count orally, you count physically, and you subtract you minus. You multiply sing it 2 times two whatever. So at XX they taught me that there is a different way to teach a child how to count. I also realized that ok if I can apply this to these children perhaps somewhere somehow it can be meaningful.'

From the interviews with the teachers during the semester and also subsequently, it was evident that they had become aware of number concept development and also, interestingly, about vocabulary for mathematics, in general. By the same token, however, their practice during and after the intervention showed only minimal infusion of their newly acquired insight. In the classroom observations, the author was surprised to see teachers almost 'brushing off' what they had learned and just mimicking the curriculum directives. Some of the pertinent observations were as follows:

• Starting lessons with chorus imitation of expressions such as, 'so, what do you know about three?'

• 'Show me on the board what one more than three is'

• 'Draw a picture of how many there are'.

• Teachers refraining from assessing prior knowledge (levels of number concept development).

• Teachers following the weekly curriculum at the required pace. Limited questioning about learner experience in class or at home.

• Teachers are hesitant to pause when it is evident that learners may not be understanding what she is explaining or demonstrating.

• Haphazard reference of different languages and code-switching.

As an illustration of how the author put together various codes for interview excerpts and for the categories that were amalgams of a set of codes. Figure 4 shows how utterances from teachers were coded, categorised and then grouped in category groups that showed a central pattern. The author linked several categories to articulate the pattern. Despite what the teachers had learned in the programme and what they had accepted as worthwhile knowledge, they were not able to insert their knowledge into their practice. Upon reflection with a smaller group (n = 5) of them it was clear that they wanted to work more slowly and go more deeply into what the learners knew and understood but that they felt they had to 'move on' to the next topic. Unfortunately, in the work with which the author is involved in schools, it is clear that teachers are driven by the authority of the curriculum and that their own knowledge, whether newly constructed or based on experience, suggested otherwise. Figure 4 shows an example of how a central pattern from the data was constructed based only on the utterances of the teachers. However, fieldnote analysis corroborated this summation or integration.

Ultimately, with this 'glimpse' of data in the project, the author argues that the teachers, despite their ostensibly 'low', self-reported professional status, had progressed well during the duration of the programme, and that it is worth considering how preservice teacher education programmes for Grade R and for the foundation phase, in general, may inhibit their expertise, whether newly acquired or from experience. This was mainly evident in semi-structured interviews. The programmes not only have a very strong pedagogical content (De Villiers 2015), specifically with regard to general pedagogical knowledge, as described by Shulman (1986) but also PCK for teaching of early number concepts. In addition, the author argues that the strong position taken by Berch (2016), and with which she agrees, captures some of what we have witnessed in the programme. He proposes that the fields of cognitive science and mathematics education 'suffer' from a 'developmental discontinuity'. Mathematical cognition, a field in which much has been researched in the last 30 years (see, e.g., Carey 2009; Carey, Zaitchik & Bascandziev 2015; Feigenson, Dehaene & Spelke 2004; Henning & Ragpot 2015; Wynn 1992) and mathematical learning difficulties research (see ed. Chinn 2015) and conceptual change theory (ed. Barner & Baron 2016) and neuroscience (Dehaene 2011) has not been taken up by mathematics education researchers as much as they do in pedagogy and evaluation of programmes. The teachers in this study, as studied by Henning (2013), have shown that pedagogy can be strengthened by a good dose of mathematical cognition evidence and cognitive science, in general.

 

Acknowledgements

Competing interests

The author declares that they have no financial or personal relationships that may have inappropriately influenced them in writing this article.

Author's contributions

L.N. is the sole author of this article.

Ethical considerations

Ethical clearance to conduct this study was obtained from the University of Johannesburg Faculty of Education Research Ethics Committee (No. 2017-113).

Funding information

This research study received no specific grant from any funding agency in the public, commercial or not-for-profit sectors.

Data availability

Data sharing is not applicable to this article as no new data were created or analysed in this study.

Disclaimer

The views and opinions expressed in this article are those of the author and do not necessarily reflect the official policy or position of any affiliated agency of the author.

 

References

Barner, D. & Baron, A.S. (ed.), 2016, Core knowledge and conceptual change, Oxford University Press, Oxford.

Berch, D., 2016, 'Disciplinary differences between cognitive psychology and mathematics education: A developmental disconnection syndrome', Journal of Numerical Cognition 2(1), 42-47. https://doi.org/10.5964/jnc.v2i1.23        [ Links ]

Bezuidenhout, H.S., 2020, 'The interface between early numeracy, language and learning environments: Pedagogical implications', South African Journal of Childhood Education 10(1), a923. https://doi.org/10.4102/sajce.v10i1.923        [ Links ]

Bezuidenhout, H.S., (in press), 'Associations between early numeracy and mathematics-specific vocabulary', South African Journal of Childhood Education.

Bransford, S.M., Brown, A.L. & Cocking, R.R., 1999, How people learn: Mind, experience and school, National Academy Press, Washington, DC.

Carey, S., 2009, The origin of concepts, Oxford University Press, Oxford.

Carey, S., Zaitchik, D. & Bascandziev, I., 2015, 'Theories of development: In dialog with Jean Piaget', Developmental Review 38, 36-54. https://doi.org/10.1016/j.dr.2015.07.003        [ Links ]

Chinn, S. (ed.), 2015, The Routledge international handbook of dyscalculia and mathematical learning difficulties, Routledge, London.

Clements, D. & Sarama, J., 2015, 'Discussion from a mathematics education perspective', Mathematical Thinking and Learning 17(2-3), 244-252. https://doi.org/10.1080/10986065.2015.1016826        [ Links ]

Clements, D.H. & Sarama, J., 2009, Learning and teaching early math: The learning trajectories approach, Routledge, New York, NY.

Creswell, J.W., 2014, Research design: Qualitative, quantitative and mixed methods approaches, 4th edn., Sage, Thousand Oaks, CA.

Darling-Hammond, L. & Bransford, J., 2005, Preparing teachers for a changing world, Jossey-Bass, San Francisco, CA.

Dehaene, S., 2011, The number sense. How the mind creates mathematics, Oxford University Press, Oxford.

Department of Basic Education, 2022, Early grade mathematics research Indaba, DBE, Pretoria.

Desoete, A., 2015, 'Predictive indicators for mathematical learning disabilities/dyscalculia in preschool children', in S. Chinn (ed.), the international handbook for mathematical difficulties and dyscalculia, pp. 90-101, Routledge, London.

De Villiers, H., 2015, 'Die bruikbaarheid van die Afrikaanse weergawe van die MARKO-D toets [The usefulness of the Afrikaans version of the MARKO-D test]', Med thesis, Department of Childhood Education, University of Johannesburg, Johannesburg.

Dlamini, A., (under review), 'A linguistic perspective on Grade 3 learners word problem-solving', MEd dissertation in the Faculty of Education, University of Johannesburg, Johannesburg.

Dowker, A.D. & Nuerk, H., 2016, 'Linguistic influences on mathematics', Frontiers in Psychology 7, 1-4. https://doi.org/10.3389/fpsyg.2016.01035        [ Links ]

Feigenson, L., Dehaene, S. & Spelke, E., 2004, 'Core systems of number', Trends in Cognitive Sciences 8(7), 307-314. https://doi.org/10.1016/j.tics.2004.05.002        [ Links ]

Fritz, A. & Ehlert, A., 2016, 'Learning difficulties', in L. Ramrathan, L. le Grange & P. Higgs (eds.), Education studies for initial teacher development, pp. 365-382, Juta, Cape Town.

Fritz, A., Ehlert, A. & Balzer, L., 2013, 'Development of mathematical concepts as basis for an elaborated mathematical understanding', South African Journal of Childhood Education 3(1), 22-38. https://doi.org/10.4102/sajce.v3i1.31        [ Links ]

Fuson, K.C., 1988, Children's counting and concepts of number, Springer, New York, NY.

Gallistel, C.R., 1999, 'Coordinate transformations in the genesis of directed action', in B. Martin & D.E. Rumelhart (eds.), Cognitive science, pp. 1-42, Academic Press, Cambridge, MA.

Gelman, R. & Gallistel, C.R. (eds.), 1986, The child's understanding of number, Harvard University Press, Cambridge, MA.

Henning, E., 2013, 'Teachers' understanding of mathematical cognition in childhood: Towards a shift in pedagogical content knowledge?', Perspectives in Education 31(3), 139-154.         [ Links ]

Henning, E., 2016, 'Reading as learning in the primary school', South African Journal of Childhood Education 6(1), a504. https://doi.org/10.4102/sajce.v6i1.504        [ Links ]

Henning, E., Balzer, L., Ehlert, A. & Fritz, A., 2021, 'Development of an instrument to assess early number concept development in four South African languages', South African Journal of Education 41(4), 1-12. https://doi.org/10.15700/saje.v41n4a1979        [ Links ]

Henning, E. & Ragpot, L., 2015, 'Symbolic knowledge of pre-school children: The genesis of a cognition lab at a South African University', South African Journal of Psychology 45(1), 71-80. https://doi.org/10.1177/0081246314545199        [ Links ]

Henning, E., Van Rensburg, W. & Smit, B., 2004, Finding your way in qualitative research, Van Schaik Publishers, Pretoria.

Herzog, M., Fritz, A. & Jansen van Vuuren, E., 2018, 'Meerkat Maths - A comprehensive maths learning programme for Grade-R', South African Journal of Childhood Education 8(2), 1-10. https://doi.org/10.4102/sajce.v8i2.565        [ Links ]

Kortjass, M., Mphahlele, R., Van der Haar, H., Webb, L., Mathews, C. & Henning, E., 2021, 'Teacher educators of numeracy', in S. Gravett & E. Henning (eds.), Glimpses into primary school teacher education in South Africa, p. 104, Routledge, London.

Kvale, S., 1983, Interviews: An introduction to qualitative research interviewing, Sage, Thousand Oaks, CA.

Merriam, S., 2009, Qualitative research: A guide to design and implementation: Revised and expanded from qualitative research and case study applications in education, Jossey-Bass, San Francisco, CA.

Ndabezitha, L.B., 2018, 'Grade R teachers' pedagogical content knowledge about the development of children's numerical cognition', MEd Dissertation, Department of Childhood Education, University of Johannesburg, Johannesburg.         [ Links ]

Ntsoane, R., 2018, 'How student teachers engage with the making of teaching tools for mathematics pedagogy', Med thesis, Department of Childhood Education, University of Johannesburg, Johannesburg.         [ Links ]

Piper, B., 2022, Early numeracy interventions in Kenya, Early Grade Mathematics Research Indaba, London.

Shulman, L.S., 1986, 'Knowledge and teaching: Foundations of the new reform', Harvard Educational Review 57, 1-22. https://doi.org/10.17763/haer.57.1.j463w79r56455411        [ Links ]

Simelane, F., 2018, 'Early grade teachers and children's difficulties with mathematics learning', MEd dissertation, Department of Childhood Education, University of Johannesburg, Johannesburg.         [ Links ]

Strauss, A. & Corbin, J., 1999, Basics of qualitative research: Grounded theory procedures and techniques, Sage, Newbury Park, CA.

The Reading Panel, 2022, Learning losses due to the COVID- pandemic, Daily Maverick, Cape Town.

Van Vuuren, E.J., Herzog, M. & Fritz, A., 2021, 'Theory-based early numeracy programme adapted to learners' pre-knowledge', in S. Gravett & E. Henning (eds.), Glimpses into primary school teacher education in South Africa, pp. 115-128, Routledge, London.

Writer, S., 2020, 'The shocking state of maths and science education in South Africa', BussinessTech, viewed 01 April 2022, from https://businesstech.co.za/news/government/455406/the-shocking-state-of-maths-and-science-education-in-south-africa/

Wynn, K., 1992, 'Children's understanding of counting', Cognition 36, 155-193. https://doi.org/10.1016/0010-0277(90)90003-3        [ Links ]

 

 

Correspondence:
Lerato Ndabezitha
lndabezitha@uj.ac.za

Received: 22 Feb. 2022
Accepted: 13 Apr. 2022
Published: 28 July 2022