Services on Demand
Article
Indicators
Related links
-
 Cited by Google
Cited by Google -
 Similars in Google
Similars in Google
Share
South African Journal of Childhood Education
On-line version ISSN 2223-7682
Print version ISSN 2223-7674
SAJCE vol.3 n.2 Johannesburg 2013
Mediating learning number bonds through a Vygotskian lens of scientific concepts
Mike Askew
Monash University and the University of the Witwatersrand. Email address: mike.askew@monash.edu
ABSTRACT
Children's learning of early number bonds is a significant issue in South African schools because evidence shows that flexible and efficient (fluent and reasoned) knowledge of number bonds to 20 correlates with success at the end of primary schooling, yet the evidence is that many South African students are over-reliant on inefficient counting methods. This paper focuses on why and how treating early number bonds as scientific concepts may be the key to raising learners' attainment in these. The paper argues that teacher mediation is crucial and that mediation for learning scientific concepts has to be meaningful, relational and transcendent. This theoretical position is illustrated with examples from a dynamic assessment interview with a Grade 2 learner carried out as part of the Wits Maths Connect - Primary project. It concludes by suggesting the implications for teacher education and professional development.
Keywords: foundation phase, Vygotsky, mediation, number bonds, South Africa
Introduction
This paper examines how young learners develop early number bonds and the role that teaching plays in supporting their development. It does this by first examining views on learning in general and in particular recent positions on the Piagetian "developmental stages" view of young children's thinking. In the light of arguments that young children, given an appropriate context and support from more experienced others, can engage in abstract thinking I argue that they can learn about the structure of number and not simply commit number bonds to memory as an empirically established collection of unrelated facts. The paper then examines what research tells us about successful learners of number with regard to proficiency in learning simple calculations and understanding the numerical structure.
In the second part of the paper I consider teaching implications for supporting the learning of number structure. Turning to Vygotsky, I examine how his theory of mediation might be interpreted in this context, and how teacher mediation needs to be reciprocal and intentional, focused on meaning, and transcendent in aiming beyond immediate success (Feuerstein, 1990). I illustrate this in practice with extracts from a dynamic assessment interview with a Grade 2 learner, Sam (a pseudonym). In conclusion I consider the implications of what might be considered to be "good" early years numeracy teaching and suggest directions for both classroom practice and research.
The "developmental stages" view of learning
Although Piaget's theory of "developmental stages of thinking" is no longer so central to the discourses of early childhood education, in practice it does still seem to be a popularly held view that young children cannot reason in the same way that adults can. Teachers may no longer explicitly talk in terms of young learners needing to be developmentally "ready" to engage with the structure of number but in practice, teaching in foundation stage classrooms is still often characterised by practices that rest on assuming young learners can only learn about number through concrete, empirical experiences, and establishing number bonds as a discrete collection of "facts" (Venkat & Askew, 2012). Such a view can reinforce two tenets of foundation stage teaching:
- That the "concrete" is more important than the "abstract" for young children;
- That moving from the concrete to the abstract is developmentally based - that children's thinking has to have developed to a certain "stage" before they can reason mathematically.
Recent research by scholars following in the tradition of Piaget indicates, however, that this view of children's thinking moving through distinct, qualitatively different stages of development is mistaken: that the structures for thinking abstractly are in place from an early age. What distinguishes young children's thinking is not the quality of their ability to reason perse, but the limits of their experiences. For example, long-term Piagetian researchers Peter Bryant and Usha Goswami (2010) argue that this part of Piagetian theory is incorrect and that the evidence now shows that the structures for thinking used throughout our lives are in place from a very early age. It is not the case that young children cannot reason abstractly; rather, the range of things they can reason about is limited due to their lack of worldly knowledge. Experience is what is needed to help children expand their range of abstract reasoning, not waiting for them to "develop" into a particular form of thinking. Such research lends support to Vygotskian theory positioning development as following on from learning. The experiences that we help young learners engage with are what help them to develop, not that they have to have reached a certain stage of development before they can engage with abstract concepts. The claim that learning leads development establishes two new positions regarding learning and teaching in the foundation stage:
- That the "abstract" is just as important as the "concrete" for young children - it is lack of experience that accounts for children's thinking being less accomplished, not an inability to reason;
- That movement between the concrete to the abstract is experientially based - children have a similar quality of thinking and reasoning available to them as adults do.
Successful learning of proficiency in basic calculation
If young children are capable of abstract reasoning, then what are the implications for teaching early number, and in particular, fluency with number bonds to 20? Answering this question is important as there is strong evidence that single digit addition and subtraction is strongly correlated with being successful in mathematics at primary school (Cowan, Donlan, Shepherd, Cole-Fletcher, Saxton & Hurry, 2011). But there is also evidence that within the South African educational system, many learners are not progressing beyond empirical, counting-all, strategies to establish number bonds (Ensor, Hoadley, Jacklin, Kuhne, Schmitt, Lombard & Van den Heuvel-Panhuizen, 2009).
Research evidence shows that successful learners generally use three approaches to answering calculations like 5 + 6 or 13 - 7.
- Counting - either counting all (putting out five objects, another six and counting the total) or counting on from one of the numbers (and coming to appreciate the counting on from the larger number is more efficient) or counting back in the case of subtraction.
- Decomposition - splitting one or both of the numbers to make use of number facts that the learner can retrieve: for example thinking of 5 + 6 as 5 + 5 + 1 or double six minus 1, or using 14 - 7 = 7 (from rapid retrieval of doubles) to deduce that 13 - 7 must be 6.
- Retrieval - knowing the answer. Children can recall that 5 + 6 = 11 (with 3 seconds being the benchmark time used for counting learners as using retrieval).
A key question is: what is the relationship between and growth in the use of these three approaches? Two views are in evidence in the literature:
1. The progression view: Children progress from counting strategies, to derived (decomposition) strategies, to retrieval (Askew, 1998); and
2. The number sense view: That proficiency in basic calculations at any age means selecting an efficient strategy, not just retrieval (Baroody, 2006).
The "progression" view could be seen to align with a Piagetian stages or development view: that becoming more sophisticated in the approaches to calculating means moving through distinct, linear, stages of calculating. Research however, is showing more evidence for the number sense view, once again supporting a move to a Vygotskian perspective for learning leading development.
The evidence also shows that there is no clear hierarchy of these strategies: children will make use of all three, even until quite late in their primary schooling. Drilling children to try and improve retrieval does not make them any more likely to use that method and often they will revert to using a counting method to ensure that they are correct. Trying to move children on to use only retrieval does not appear to be that successful and children who use a mix of the methods seem to be most successful (Cowan et al., 2011).
Thus it seems that the view that children need to be fluent in the "basics" (that is being able to rapidly retrieve number bonds) before they can reason mathematically is challenged - fluency in calculation and reasoning about the number system are mutually entwined, each supporting and being supported by the other.
The assertion that reasoning about the number system is linked to proficiency in basic calculation, means we need to be clear about the sort of reasoning that young learners can engage in. Research, as summarised by Cowan et al. (2011) suggests two strands of reasoning to attend to: number system knowledge and calculation principles.
Number system knowledge
Learner knowledge, skills and reasoning here includes:
1. Fluent and accurate counting in ascending sequences (e.g. 294 to 310) and descending sequences (e.g. 425 to 417).
2. Knowledge of the number sequence: Given a number learners are able to say what number comes right after, just before, two numbers after, four numbers before and so forth.
3. Appreciating relative magnitude: learners can say which of a pair of numbers (e.g. 51 & 39) is larger.
4. A sense of numerical distance: identifying which of two numbers is closer to a target number (e.g. Which number is closer to 102: 178 or 109?)
5. Reasoning about relative differences: which of two pairs of numbers has the greater difference (e.g. Which difference is bigger: the difference between 25 & 20 or 25 & 11?
Calculation principles
Learner knowledge, skills and reasoning here would include:
- Using derived facts (e.g. Given the answer to 20 - 5 = 15 children can solve a linked problem by using that answer, for example 21 - 5 =?)
- Appreciation of six principles regarding addition and subtraction:
1. Commutativity of addition (e.g. Given that 48 + 87 = 135, knowing this will give the answer to 87 + 48 =?)
2. Subtrahend minus one (e.g. Given that 373 - 345 = 38 being able to reason that 373 - 344 will be one more than 38)
3. Subtraction complement principle (e.g. Deducing from 143 - 57 = 86 that 143 - 86 must be 57)
4. Doubles plus one pattern (e.g. From 46 + 46 = 92 figuring out that 46 + 47 would have to be 93)
5. Addition and subtraction as inverses (e.g. If 38 + 59 = 97 being able to say that 97 - 59 = 38)
6. Subtrahend plus one (e.g. From the answer that 373 - 345 = 38 reasoning that 373 - 346 must be one less than 38).
- Explaining patterns: Being able to explain patterns such as n + 1 whereby children can explain how when you add one to a number the answer is always the next number when you count. Other patterns that children need to explore and be able to articulate include:
n - n
n +10
n - 0
n - (n - 1)
n + 0
(The above adapted from Cowan et al., 2011.)
Number bonds: scientific or spontaneous concepts?
In the light of the above analysis of the reasoning involved in developing early number bonds, I take the position that we need to treat learning addition and subtraction bonds as, in Vygotskian terms, scientific concepts, in contrast to treating them as spontaneous concepts.
As Daniels (2001) points out, there is no clear definition of the distinction between the scientific concept and the spontaneous (which some writers refer to as the "everyday" - henceforth I will use spontaneous). Kozulin's distinction between learning content and appropriating scientific knowledge is helpful. He suggests that learning "content material often reproduces empirical realities with which students become acquainted in everyday life" (Kozulin, 1998:25). This does not mean that spontaneous content knowledge is never learnt in school, as Kozulin uses the example of capital cities to illustrate. The knowledge that, say, Pretoria is the capital of South Africa can be learned as part of everyday life or within the classroom, but such knowledge is spontaneous in a particular sense of that word, in that learning this fact requires no specialised instruction: one simply has to be told and commit the content to memory.
Appropriating scientific knowledge, however, requires a different type of learning, one that
presupposes (a) a deliberate, rather than spontaneous character of the learning process; (b) systemic acquisition of symbolic tools, because such tools are systemically organised; (c) emphasis on the generalised nature of symbolic tools and their application (Kozulin, 1998:25).
Early number bonds can be treated as spontaneous or scientific. They can be seen as spontaneous - as empirical content knowledge - as learners, through their everyday actions and "empirical realities", will come to know some number bonds without exposure to deliberate instruction. For example, many young children come to know that two plus two is four or double five is ten simply through their everyday experiences. Children do come to acquire a set of "known number facts".
But spontaneously coming to know number facts is not analogous to coming to know capital cities of the world, in that number facts are "systematically organised" and generalisable, whereas the names of capital cities are not. Knowing that Rome is the capital of Italy is of no help in establishing that Paris is the capital of France, whereas knowing that 5 + 5 = 10 can help in knowing that 5 + 4 = 9 or 5 + 6 =11. As the research summarised above shows even something as seemingly simple and empirical as learning the addition and subtraction bonds of numbers to twenty involves a number of systematic generalisations and that developing number sense rests on a blend of "known facts" (spontaneous) and "derived facts" (scientific).
As part of the Wits Maths Connect - Primary project, many lessons observed have been focused on teaching early number bonds with the majority of these seeming to treat number bonds as everyday, empirical - that is spontaneous - knowledge. For example, Venkat and Askew (2012) report on lessons where despite the presence of artefacts that could be used to model scientific aspects of number bonds - for example the abacus with the structure of ten - teachers repeatedly empirically established number bonds through counting in ones. The evidence from South African studies showing learners' continued use of unit counting strategies as applied to calculations beyond where this would be an effective approach (see, for example, Schollar, 2008) suggests that treating numbers as spontaneous concepts may be a dominant approach in South Africa.
Treating early number bonds as scientific concepts means teaching for these to become psychological tools (Vygotsky, 1978). Psychological tools, when internalised, allow the learner to do things that go beyond their "natural" psychological functions, such as perception, memory or attention. For example, there is evidence that a sense of "numerosity" is "natural" as shown by studies of very young children attending more to scenarios displaying numerically incorrect situations (one orange being removed from a set of two to reveal two oranges still remaining) than to similarly correct situations (one orange removed from two to leave one) (Dehaene, 1999). Such perceptual, natural, responses to numerical situations are, however, limited in their application and only through the appropriation of mathematics as a psychological tool can we carry out calculations such as 28 + 39 by reasoning that 28 plus 40 would be 68 and so adding 39 would be one less, that's 67. That type of reasoning is only possible through the appropriation of the cultural and historical tool of place value: without a collection of objects grouped into sets of ten such mental manipulation of the signs used to represent the quantities is not possible (imagine how much more challenging it would be to calculate XXVIII + XXXIX). At the heart of appropriation of such psychological tools is the Vygotskian construct of mediation.
Defining mediation
A central concern arising from Vygotskian approaches is to understand the sorts of relations and actions between the more knowledgeable other (usually an adult, but possibly a peer) that enable the less experienced to internalise psychological tools. Rogoff (1995) suggests three different perspectives on mediation: apprenticeship (community activity where the learner moves from novice to expert), guided participation (supporting the learner towards achieving a specific object) and appropriation (whereby individuals develop through their involvement in joint activities). The stance towards mediation taken here is therefore one of appropriation: to explore how mediation helps learners not only succeed with particular tasks but also aims to bring about the development of psychological tools. This is the nature of Vygotsky's claim for learning leading development - children do not develop into a "stage" whereby understanding the nature of addition becomes possible, it is working "as though" they understand addition that allows the development to occur. This is the essence of working in the Zone of Proximal Development - the adult and child working together to create a (metaphorical) space where more can be achieved jointly than by the child alone. Here Bruner's (1985) notion of the adult acting as a "vicarious consciousness" is more helpful than the notion of the adult "scaffolding" the learning. That vicarious consciousness has to have a dual nature. First, it has to be the consciousness of the more experienced other, the consciousness that has the awareness of what is possible that the learner is not yet aware of. Second it also has to have the quality of vicariously intuiting the consciousness of the learner at that moment in time: what the learner's responses and actions suggest they are (perhaps only implicitly) aware of.
Acting only with the awareness of the experienced other may "scaffold" the learner to achieve the desired outcome, but it may not affect the development of the learner: the learner may not appropriate any of the awareness or consciousness of the expert, and may simply pick up cues that enable them to complete the task at hand. In Piagetian terms, the adult acting as a vicarious consciousness has to assume that the learner will assimilate something of their consciousness and this needs to be accompanied by accommodation. Without accommodation, without some restructuring of the learner's existing ways of thinking, any assimilation is fragile. Hence, the second aspect of vicarious consciousness: building a model of what the learner's current consciousness may be. In Kozulin's terms, "human experience is always present in two different planes - the plane of actual occurrences and the plane of their internal cognitive schematizations" (Kozulin, 1998:10). For the teacher to act as a vicarious consciousness they need not only to be aware of the plane of their own cognitive schematisations but also through the interactions build a model of the learner's plane of cognitive schematisations - the mediating actions then arise from the dance between these two. Mediation is thus, in Prawat's (1991) interpretation of Vygotsky's later work, social, embodied and transactional.
Thus I argue that while the teaching of number bonds can be treated as simply the learning of spontaneous content knowledge, through appropriate mediation it may be possible to teach these in ways in which they become part of the psychological tool of mathematics, that learners appropriate an awareness of arithmetical structure that can function more generally across different tasks and contexts.
Kozulin notes that much of the research into mediation is data-driven - that is, arises from observational data of adult-child interactions from which the key aspects of mediation are identified. Fewer studies start with theoretical models of mediation, which are then examined in action. Notable in this theory driven respect is Feuerstein's (1990) theory of mediated learning experiences (MLE) which posits that mediation is only enacted when certain criteria are met: intentionality, reciprocal interactions, a focus on meaning and transcendence (in aiming to provoke learning at goes beyond the immediate situation being engaged in). These criteria fit with a perspective on mediation as appropriation and I show in the case example that follows the mediational moves that are directed towards this end.
Mediation in action
The Wits Maths Connect - Primary Project, begun in 2011, it is a five-year research and development focused project working with ten government primary schools in one district. As part of this project the author carried out a number of interviews with the Grade 2 learners in one of these schools, primarily to explore their understanding of part-part-whole relations. As the interviews unfolded they took on some characteristics of dynamic assessment (Lidz, 1987) in that the interaction between the interviewer and the students moved from foci primarily on eliciting learners prior understandings to having a more transactional character and exploring whether learners could be encouraged to think differently about number and in particular to move from a focus on counting strategies to developing and drawing on some of the understandings about the number system and calculating strategies listed above.
Two examples are used here from one such interview to illustrate the sorts of mediation that might help learners move towards treating number bonds as scientific concepts. The first extract comes about a third of the way into the interview when gaps in the learner's (Sam) understanding start to become apparent. The first part of the interview had focused on exploring part-part-whole relations through a series of questions based on placing counters in a bag. For example, if eight counters were put into the bag and five removed, could Sam say how many were left in the bag? I introduced Sam to using a bar diagram to record what was happening in terms of part-part-whole. For example, in this instance, the whole was eight, with one part being five. So this would be initially recorded as in Figure 1.
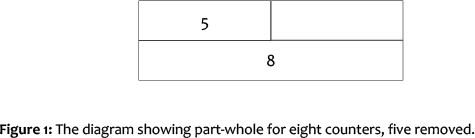
Once Sam had figured out the answer, he would write this in the diagram, as in Figure 2.
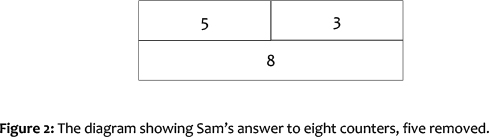
As Sam displayed little difficulty in answering such questions when the numbers involved were all within a total of ten I decided to move to totals in the teens. M is the interviewer, S is Sam.
Episode 1
M: Okay, we're going to put 2, 4, 6, 8 in?
S: Yes.
M: Eight. So where are you going to put the 8 on the diagram? S writes 8 in the first small block
M: Okay. Close your eyes. [M puts some counters(7) into the bag.] I've put some more in. How many do you think are in the bag now? S is silent for about 14 seconds, only moving his fingers.
M: Just have a guess. S sits quietly for about 21 seconds [This is an interesting moment - up until now in the interview Sam has been very confident and willing to give answers (all of which have been correct), but clearly he was unwilling to give an answer to which he could not know exactly, and unwilling even to guess.]
M: Okay, let's count them. [M empties the bag onto the desk.]
S: (Counting them singly, moving them as he counts.) 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12, 13, 14, 15. [I note first that Sam counts these singly and also that he effectively separates the counted from the yet-to-be-counted counters, ensuring that he neither double counts nor misses any. In previous examples, I have modelled counting in two's in the hope that Sam might appropriate this and move from counting in ones, but he does not do so here.]
M: Fifteen. S writes 15 in the large rectangle and then writes 3 in the other space - see Figure 3.
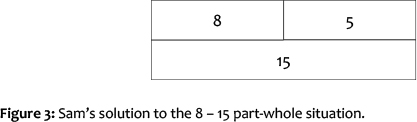
M: Okay, how do you know it's 3? [At this point there is no obvious logic, that I can see, that might lead to the incorrect answer of 3. The question is seeking genuine understanding - I cannot mediate through acting as a vicarious consciousness until I can enter into Sam's frame of thinking.]
S: Because 8 plus 2 plus 3 equals to 15.
M: Show me that with the counters. [I empty the 15 counters in the bag onto the table in a heap.] [The confidence with which Sam says this further suggests that there is logic behind his thinking here, but it is still not clear to me. Asking him to model this with the counters is a mediating move with a dual purpose - first in the expectation that it will provide me with further insight into Sam's reasoning, but also that through modelling it practically Sam may himself come to realise that the answer is incorrect.]
S: 2, 4, 6, 8 [separates 8 counter from the pile] 8. [Sam can count a collection in twos!]
M: So that's like the 8 I put in the bag, isn't it?
S: Yes. [S points to the eight counters] 8. I'll take 2 from there and put it here. [S moves 2 counters from the seven remaining in the pile to add to the 8 he has already counted out.] Now we have 3. [Although, to the adult, it may seem obvious that there are more than three counters left when two are removed from the pile of seven, Sam seems not to notice this. This suggests to me that he is not seeing the counters as a complete representation of the situation, that when Sam says "now we have three" he is not referring to the pile of counters but to the answer, in his head, that he had previously arrived at. Although his reasoning is still not clear, it seems that his knowledge of five being made up of two and three and eight needing two to make it up to ten is behind the error here. My decision on a mediating move here is to direct Sam to working with the counters.]
M: Yeah, but we want 15. That's how many there are in the bag altogether. [M waving his hand over all the counters in the intention of pointing out that there are fifteen in total on the table.] So, there's the 8 that I put in the bag to start off with. [M pushes back into the pile of 5 the 2 counters S just added to leave the pile of 8]. So, how many did I put in the bag while your eyes were closed?
S: 5
M: Is it 5?
S: [S moves 2 counters back to the original 8. After about a 6 second pause.] 10 plus 5 ...
[Sam's thinking time and response here further confirms my suspicions that he has some image of 8 and 5 in his head, with the 5 partitioned into 2 and 3. When here he says, "ten plus five'" I am not convinced that he is actually referring to what is now on the table - a pile of ten counters and a pile of five - or whether this is still something to do with him getting the five that this is partitioned into two and three from his knowledge of partitioning fifteen into ten and five. My mediating move is to make this latter assumption.]
M: Yes, 10 plus 5 is 15, that's right. But there were 8 in the bag. [I again move the 2 counters away and back to the first group to leave the eight clearly separate.] And that's what I put in the bag to start off with I put 8 in, didn't I?
[This is a mediating move designed to take Sam back into thinking about how everything was initially set up. I am resisting telling him that his answer is wrong, but at the same time he is reluctant to give up on it.]
S: Yes.
M: That's what we put in to start with. So, how many did I put in while your eyes were closed?
S: 5
[This mediation that is focused on trying to get Sam to reconsider his answer is not working. My move now is to set up the situation again, working with Sam's answer of five.]
M: Okay, if I have 8 and I put in another 5. Let's do that. There's the 8. [M puts the 8 counters into the bag.] And you say I put in another 5? Is that right? (M moves five counters aside). Okay, so let's count. Eight (M taps the bag, looks at S to encourage him to count along. M picks up a counter, holds it over the bag and counts as S joins in.)
M & S: (together) 9.
S: 10, 11, 12, 13. [S continues the count while M drops the counters into the bag one at a time until the pile of five is used up.]
M: We didn't have 13 we had 15! [M empties the bag onto the desk.] So, there's the 8 ... There's the 8 that was in the bag. [M counts out 8 counters.] 9, 10, 11, 12, 13, 14, 15 [M moves counters over to add to the eight, counting, until there are 15 in the pile.] So, how many did I put in the bag while your eyes were closed?
S: 7
M: Okay, how do you know it's 7?
S: Okay, this time .
M: Yeah?
S: I'm telling you the real time.
[S will not actually admit to having been wrong!]
M: Okay, you're telling me the real time. Okay, go on, then.
S: 8 plus 7 equals to 15.
M: And how do you know that? You're right, but how do you know that?
S: I take 8 first. After 8 I take 3.
[This is a surprise to me, after the repeated adding of 2 to make the 8 up to ten, adding three does not look promising. Tempting though it was to remind S that adding two made sense, I resist and let him continue.]
M: Okay, so you want to take 3. What does that make if I add the 3 to the 8?
S: 3 to the 8. 11
[He is confident and says this quickly, so we move on.]
M: 11
S: I put 4 more left.
[Again confidently, and as we have spent quite some time on the problem, I wrap it up by getting S to correct the diagram.]
M: Okay, and that makes 15. So then change that to 7 for us. Let's correct that. Let's just put a line through there and write 7.
Discussion
Sam displayed knowledge of derived number facts that lead him to being over confident, in that once he had arrived at an answer using his logic, he was reluctant to let go of that answer, even using the physical artefacts in ways to support his conclusion.
The mediation is intentional and focused on meaning - my responses to Sam are not simply to point him in the direction of how to succeed (not scaffolding his accomplishment of the tasks) but are attempting to lead him to see the contradiction in his reasoning.
In terms of being relational, the dance towards this takes time - the danger in mediating to the extent that Sam's error is effectively pointed out to him could mean a loss of attention to meaning, with the learner succumbing to taking cues for success from the adult without the possibility of coming to understand why those steps needed to be taken. On the other hand, simply leaving Sam to be content with his interpretation misses the opportunity for mediation that could lead to him appropriating new approaches. It takes time in this interaction for this mutual relationship to be established, but towards the end of the transactions it is not possible to say which of the participants brings about the awareness of the correct approach. It emerges through the mutual, relational, nature of the conversation.
The episode has a transcendent quality in bringing into attention the fact that numerals represent quantities and thus have a logic to them that complements Sam's logic, although I make no claims that this would remain in Sam's awareness beyond this moment. Only through many experiences like this would I be confident that Sam would appropriate this awareness more securely.
Episode 2
Having established the nature of part-part-whole calculation in the presence of artefacts - counters in the bag - I decided to move to asking some calculations in a purely symbolic form. The second extract starts at this point in the interview, about two-thirds of the way through.
M: Some quick calculations for us to do.
M writes 7 + 1 = [ ].
S confidently writes 8 in the placeholder.
M: Too easy, isn't it?
[I am heavily influenced by Dweck's research (2000) into praise here and her recommendation that rather than say "well done" to a child that gets something easily correct to respond in a way that suggests you, the teacher, had made an error in setting something too easy to do.]
M writes 1 + 6 = [ ].
S immediately writes 7.
[This second example was chosen to see if S would count on 6 from 1 or whether he knew that 1 + 6 was the same as 6 + 1, which his rapid response suggests he did, leading me to conclude that he is reasonably confident about the n + 1 = 1 + n pattern.]
M writes 9 - 7 = [ ].
S writes 16.
M: Okay. Read that to me.
[I am confident that S has added here, but want to check that with him.]
S: 9 ... 9 minus 7 equals to 16.
M: Okay, and why are you shaking your head?
S: It's wrong.
M: It's wrong. Alright, can you put it right? Go on then.
S takes a little time (about 24 seconds) to work it out but then crosses out 16 and writes 2.
[On reflection, given how long he took to figure this out I might have asked him what he was thinking, but did not! The fact, however, that S self-corrected his mis-reading added to my confidence that he could engage in thinking about such calculations in ways that were not simply recall.]
M: Very nice. Thank you.
M writes 9 - [ ] = 7.
S writes 2 in the placeholder.
M: Lovely.
[The speed of answering here meant it was not worth asking S how he knew the answer as it was likely to have been a known fact, but it could also be demonstrating his understanding of the subtraction complement principle.]
M writes 5 + [ ] = 7.
S confidently fills in 2.
M: Super.
M writes [ ] + 3 = 7.
S writes 4.
M: Okay.
[This and the preceding calculations were to check that S was confident with his bonds to 10.]
M writes 9 + 11 = [ ].
After about 13 seconds, S writes 11.
M: Okay. Tell me how did you work this one out?
M puts down the sheet of paper with the sum: [4] + 3 = 7 (4 filled in by S).
[I went back to the previous correct calculation as I did not want to cue S into thinking that asking how he worked something out was only done when the answer is incorrect.]
S: 4 plus 3 equals to 7.
M: How do you know that?
S: Because like this. You see we have 4 and 3 (putting out fingers on each hand, without counting them)
M: Mmm.
S: It makes 7.
M: Okay. And this one? [Showing S: 5 + [2] = 7 (2 filled in by S)]
S: 5 plus 2 equals to 7.
M: Okay. How do you know that?
S: 5 plus 2 it makes 7.
M: And this one? [Showing: 9 + 11 = [11] (11 in the placeholder filled in by S).]
S: This one? [S pointing to the new question] 9 plus 11 equals to 11. 9 plus 1 equals to 10 plus 1 equals to 11.
M: So, 9 plus 1 is 10 and 1 more is 11.
[My repeating his explanation here is more for my benefit than his, to make sure I have followed what he said.]
This is the start of having to decide how to mediate - we have, I think, entered a zone of proximal development. Everything until now has shown that S has sound fluency of addition and subtraction of single digits, so I was confident that he had enough fluency in place to begin to work with larger numbers. My choices of how to mediate here boil down to (1) working on this particular calculation and supporting S in reaching a correct answer or, (2) try to help him come to the realisation that his answer is not sensible. The confidence with which he showed how he knew four plus three and five plus two, linking the numerals with quantities (fingers but without counting them), led me to think that he should be able to see why his answer of eleven could not be correct. I decided that we needed to re-introduce artifacts to represent the numbers as quantities and to start by exploring some other calculation, in this case five plus eleven. This was in part to see if he would make the same error again - how sedimented was this understanding - and in part to check if the answer 20 to nine plus eleven was causing a particular difficulty.
The next sequence started with me putting the paper with the incorrect answer aside, on the floor and returning to the bag and counters.
M: Okay. Let's just do one more of these? So 1, 2, 3, 4, 5 [M picks up another 5 counters and puts them in the bag.] How many are there?
S: Five.
M: Okay, quickly draw„. draw the box thing for us. Where are you going to put
the 5?
S writes 5 in the first small block - see Figure 4.
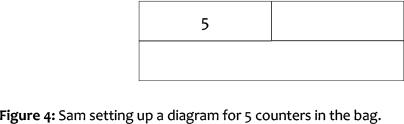
M: Lovely. And I'm going to put 2, 4, 6, 8, 10, 11 [M picks up the counters and puts them into the bag.] Can you write 11 here for us? [M points to the second small block.] So, how many altogether?
S writes 11 in the second small block and then immediately writes 16 in the large block - see Figure 5]

M: Lovely. How do you know that?
S: 5, 10 plus 5 equals to 15 plus 1 is 16.
M: Okay, so you said the 11 is 10 plus 1?
S: 10... 10 plus 5 plus 1.
[This is a key insight into Sam's thinking. He confidently can partition eleven into ten and one, add the five to the ten and then the one back on: that he does have some understanding of the 10 + n pattern and having answered 10 + 5 derived 11 + 5 from the answer. I restate "you said the 11 is 10 plus 1" in the hope that he will appropriate this and so reason similarly about nine plus eleven. I therefore now return to that calculation.]
M: Very nice. Okay. One more then. [M empties the counters out of the bag.] Can you draw me this diagram again?
S draws the rectangle.
M: This time I'm going to put 2, 4, 6, 8, 9 [M picks up the counters and puts them in the bag.]
S writes 9 in the first small block.
M: And 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11 [M puts these counters into the bag.]
S writes 11 in the second small block, glances at the papers on the floor and then writes 11 in the large block - see Figure 6]

[Having got an answer of 11, which I have previously accepted without comment and which is still visible, Sam saw no need to change anything here. I decided to return to the counters and the bag and work on the fact that Sam seems confident in adding a single digit to ten.]
M: Mmm. Okay. One more. One more. [M points to the box diagram.]
Sam draws another diagram.
M: 2, 4, 6, 8, 9 [M puts the counters in the bag two at a time.]
Sam writes 9 in the first small block, as before.
M: And 2, 4, 6, 8, 10 [M puts ten more counters in the bag, two at a time.]
In the second small block S writes 10 and then spends some time looking at this then writes 19 in the large block - see Figure 7.

M: Okay, let's check. How did you know it was 19? [M empties the counters out of the bag.]
[This checking is again to revisit the fact that Sam does seem to know how to add ten and I am still hoping that he will appropriate this in his calculation of 9 plus 11.]
S: 10 plus 9 is 19.
M: Okay. Let's check. 2, 4, 6, 8, 10, 12, 14, 16, 18, 19. [M empties the bag and counts the counters.] Now you said ... So, you've just said to me 9 ... If I had 9 in the bag and I put 10 in I get 19, but here you said [points to the sum 9 and 11 in the diagram] if I put 9 in the bag, 2, 4, 6, 8, 9 [M puts 9 counters in the bag] and I put 2, 4, 6, 8, 10, 11 [M counts out eleven counter but puts to the side of the bag], you tell me if I put 11 [M places hand over the eleven counters] in the bag there's going to be 11 in the bag?
[These mediating moves - the actions, the counting and the questioning - is intended to draw Sam's attention to the contradiction - that if nine plus ten is 19 that nine plus eleven cannot sensibly be eleven.]
S: Yes.
M: Is that right?
S: Yes.
[S is still not willing to let go of his first answer.]
M: So, how many are in here? (Tapping the bag.)
S: 9
M: And how many are here? [M points to the group of 11 counters.]
S: 11
M: So, if I put those 11 in the bag, how many are going to be in the bag?
S: (Slight pause) It's going to be 20.
M: It is going to be 20! How do you know it's going to be 20?
S: Okay. [Smiling broadly] 9 plus 1 equals to 10 plus 10 is 20.
M: Okay, so can you put that right for us please.
S: [S changes the 11 to 20.]
Discussion
Again Sam displays a mix of knowledge of known facts, derived facts and manipulation of symbols when the numbers are greater than ten. There is sense to Sam's thinking here: once the numbers become more than can be shown on fingers then it can be more efficient to work at the symbolic level. The difficulty is that Sam, in moving to the symbolic, ceases to think about whether his approaches make sense when considering the numerals to represent quantities.
My mediation is intentional and focused on meaning, attempting to bring Sam round to seeing how nine plus eleven could not be eleven, although, as in the previous extract, we see how Sam is reluctant to relinquish his solution. Once again, establishing a relational set of transactions - where each of us has appropriated some of the consciousness of the other - takes some time. And, as in the other episode, once this point is reached then the episode has a transcendent quality in that Sam may have appropriated the approach of thinking in terms of quantity as well as numerals, but again this would need to be revisited over time.
Conclusion
I have argued that mediation can only occur when the teacher is treating the content as scientific. For example, a card matching game based around selecting pairs of cards that marry up capital cities with countries may help learners commit these facts to memory, but the teacher, in introducing the game, is not mediating this learning if we take the definition of mediation as appropriation that includes meaning and transcendence: what is learned does not transcend the specific content of the game (if the capital of Nepal is not on the cards, playing the game cannot help the child determine what this capital is). Similarly a game based around matching up pairs of cards with a total of ten, is not mediated activity. Although some children through playing the game may begin to see some structure to the pairs of bonds to ten, but that is where the issue of intentionality comes in - a happy by-product is not the same as an intentionally mediated outcome.
In the data collected as part of the Wits Primary Connect project, many of the lessons show teachers treating number bonds as everyday concepts and consequently, in the interpretation of mediation that I have adopted here, their actions would not count as mediating learning. As the professional development work with these teachers progresses, the research team is looking for evidence of shifts towards treating number bonds as scientific concepts and the consequent types of mediation that would then be entailed.
Finally, working one-on-one with a learner to establish meaning, relations and transcendence is very different from establishing these with a class of learners and more research is needed into what mediation might look like in such circumstances.
References
Askew, M. 1998. Teaching primary mathematics: A guide for students and newly qualified teachers. London: Hodder and Stoughton. [ Links ]
Bliss, J., Askew, M. & Macrae, S. 1996. Scaffolding school knowledge through discourse: difficulties and issues. Paper presented at the annual meeting of the American Educational Research Association, New York.
Baroody, A.J. 2006. Why children have difficulty mastering the basic number combinations and how to help them. Teaching Children Mathematics, 13:22-31. [ Links ]
Bruner, J. 1985. Vygotsky: a historical and conceptual perspective. In Culture, Communication and Cognition: Vygotskian Perspectives;21-34. Cambridge: Cambridge University Press. [ Links ]
Cowan, R., Donlan, C., Shepherd, D.-L., Cole-Fletcher, R., Saxton, M. & Hurry, J. 2011. Basic calculation proficiency and mathematics achievement in elementary school children. Journal of Educational Psychology, 103:786-803. [ Links ]
Daniels, H. 2001. Vygotsky and Pedagogy. London and New York: Routledge/Falmer. [ Links ]
Dehaene, S. 1999. The number sense: How the mind creates mathematics. Oxford: Oxford University Press. [ Links ]
Dweck, C.S. 2000. Self-theories: Their role in motivation, personality, and development. Philadelphia: Psychology Press (Taylor and Francis Group). [ Links ]
Ensor, P., Hoadley, U., Jacklin, H., Kuhne, C., Schmitt, E., Lombard, A. & Van den Heuvel-Panhuizen, M. 2009. Specialising pedagogic text and time in Foundation Phase numeracy classrooms. Journal of Education, 47:5-30. [ Links ]
Feuerstein, R. 1990. The theory of structural cognitive modifiability. In B. Presseisen (Ed.), Learning and thinking styles: Classroom applications; 68-134. Washington, DC: National Education Association. [ Links ]
Goswami, U., & Bryant, P. 2010. Chapter 6: Children's cognitive development and learning. In R. Alexander (Ed.), The Cambridge Primary Review Research Surveys. London: Routledge. [ Links ]
Kozulin, A. 1996. Psychological tools and mediated learning. In A. Kozulin (Ed.), The Cambridge Companion to Vygotsky; 16-38. Cambridge: Cambridge University Press. [ Links ]
Lave, J. & Wenger, E. 1991. Situated learning: Legitimate peripheral participation. Cambridge: Cambridge University Press. [ Links ]
Lidz, C. 1987. Dynamic assessment. New York: Guilford Press. [ Links ]
Prawat, R.S. 1991. The value of ideas: the immersion approach to the development of thinking. Educational Researcher, 20:3-10. [ Links ]
Rogoff, B. 1995. Observing sociocultural activity on three planes. In J. Wertsch, P. Del Rio & A. Alvarez (Eds.), Sociocultural studies of mind; 139-164. New York: Cambridge University Press. [ Links ]
Schollar, E. 2008. Final Report: The primary mathematics research project 2004-2007 -Towards evidence-based educational development in South Africa. Johannesburg: Eric Schollar & Associates. [ Links ]
Venkat, H. & Askew, M. 2012. Mediating early number learning: specialising across teacher talk and tools? Journal of Education, 56:67-89. [ Links ]
Vygotsky, L.S. 1978. Mind in Society. Cambridge, MA: Harvard University Press. [ Links ]
Wood, D.J., Bruner, J.S. & Ross, G. 1976. The role of tutoring in problem solving. Journal of Child Psychology and Psychiatry, 17:89-100. [ Links ]














