Services on Demand
Article
Indicators
Related links
-
 Cited by Google
Cited by Google -
 Similars in Google
Similars in Google
Share
South African Journal of Childhood Education
On-line version ISSN 2223-7682
Print version ISSN 2223-7674
SAJCE vol.3 n.1 Johannesburg 2013
Development of numerical concepts
Sabine Peucker; Steffi Weißhaupt
Institute of Psychology, University of Education Freiburg, Germany. E-mail addresses: peucker@ph-freiburg.de, weisshaupt@ph-freiburg.de
ABSTRACT
The development of numerical concepts is described from infancy to preschool age. Infants a few days old exhibit an early sensitivity for numerosities. In the course of development, nonverbal mental models allow for the exact representation of small quantities as well as changes in these quantities. Subitising, as the accurate recognition of small numerosities (without counting), plays an important role. It can be assumed that numerical concepts and procedures start with insights about small numerosities. Protoquantitative schemata comprise fundamental knowledge about quantities. One-to-one-correspondence connects elements and numbers, and, for this reason, both quantitative and numerical knowledge. If children understand that they can determine the numerosity of a collection of elements by enumerating the elements, they have acquired the concept of cardinality. Protoquantitative knowledge becomes quantitative if it can be applied to numerosities and sequential numbers. The concepts of cardinality and part-part-whole are key to numerical development. Developmentally appropriate learning and teaching should focus on cardinality and part-part-whole concepts.
Keywords: Numerical development, numerical abilities, numerical cognition, numerical concept, cardinality, part-part-whole
Introduction
Research on numerical development has been conducted when children were only a few days old and revealed the remarkable capacity to work with numerosities (early sensitivity for numerosities) virtually from birth. Infants are able to compare quantities and they are able to identify changes in quantities. Infants are precise in small set sizes and estimate with increasing accuracy in larger set sizes. Young children use nonverbal mental models to represent collections of elements and changes in collections with precision. Children have fundamental knowledge about quantities. They know, in general, that quantities increase if elements are added, and quantities decrease if elements are removed (protoquantitative schemata). Children are predisposed to a store of pre-numerical knowledge when numbers eventually manifest themselves. Subitising allows for the exact recognition of 1 to 4 elements without counting. Subitising plays an important role in numerical development, because it can be assumed that numerical facts are initially discovered in small sets of elements. Concepts and procedures are likely to be developed and used in small sets. Subset-knowing leads to cardinal knowing. To arrive at the exact number of larger quantities, enumeration is needed. One-to-one-correspondence between the number and the element builds the connection between knowledge of quantities and knowledge of numbers. Children acquire the concept of cardinality if they understand that enumeration determines the numerosity of a collection of elements. Later on, numerals can be matched to representations of elements. Subsequently, numerals themselves can be enumerated. Protoquantitative part-whole-schema can be applied to concrete quantities, and, as a result, to numbers (part-part-whole-concept). More advanced counting strategies and arithmetical strategies are based on the part-part-whole conception of numbers.
The concepts of cardinality and part-part-whole turn out to be the key concepts in the developmental process through which children learn arithmetical concepts and skills. Alternatively stated, the concepts of cardinality and part-part-whole are essential to children's arithmetical knowledge. If children are not successful in acquiring these concepts, they persist in using ineffective calculation strategies (mostly counting), and can hardly understand the place-value system or fractions.
The development of numerical knowledge can be described as a hierarchical organisation of concepts, procedures, and the growing number of relations between them. The development of knowledge is coherent and it is necessary to trace the continuity of developmental pathways.
Notes on underlying developmental principles
As a theoretical framework for children's mathematical development, Sarama & Clements (2009) propose a hierarchic interactionalism, which is a constructivist approach, comprising principles based on general and domain specific developmental theories. Hierarchic interactionalism can provide the bedrock for the variety of theories of, and research conducted on, mathematical development presented in this article.
The key principle of hierarchic interactionalism is hierarchic development, in which later, more advanced concepts and processes, necessarily build on earlier concepts and processes. Consequently, developmental progression is more about the interaction between existing concepts and processes, than about the onset of completely new ones (Resnick, 1992; Fuson, 1988). The development of thinking is a coherent process. Siegler characterises later concepts and processes as increasingly sophisticated, more complex, abstract and powerful. A new strategy is chosen more often, once previous uses have been successful. However, the less mature, more primitive strategies do not disappear. They are applied, for example, in new or more complex situations, or if it is simply the best way of solving problems (Siegler & Alibali, 2005). This is described in Siegler's overlapping waves theory (Chen & Siegler, 2000; Siegler, 1996).
In the overlapping waves theory, Siegler (Chen & Siegler, 2000) also describes five components of strategy development: acquiring, mapping, strengthening, refining and executing. First a child acquires a new strategy, then the child generalises the strategy to other contexts (mapping). The child has to learn in which contexts the strategy is (or is not) applicable (appropriate mapping). Strengthening is the third component of strategy development and demands increased reliance on the new approaches and less reliance on older, past strategies. The fourth component is the refinement of choices; with increasing frequency children use the most efficient strategy for a certain type of task. Lastly, children's speed and accuracy in choosing appropriate strategies improve with practice (increasingly effective execution). The components are not to be seen in a strictly sequential order. The overlapping and interaction of the components is consequential. This is true of, for instance, mapping and strengthening new strategies.
Concepts and processes are often developed and used in a small area (for example small numbers), and later generalised (conveyed to larger numbers) (Fuson, 1988). Sarama & Clements (2009) refer to this as cyclic concretisation. Cyclic concretisation means also that concepts first refer to a perceptual or concrete level becoming more abstract and general. Internal mental representations can serve as mental models.
Concepts and processes influence each other in development (Baroody, 2004). To choose an appropriate strategy, or to execute procedures correctly, presupposes conceptual understanding (mutual dependency). Connections between concepts and procedures are gradually built, and a hierarchical cognitive structure is constructed (progressive hierarchisation, see also Resnick, 1992).
But how can the starting point of mathematical development be defined? This would be the place to lead the discussion about core concepts or core knowledge, which are explored later. Sarama & Clements (2009, referring to Karmiloff-Smith, 1992) describe the initial bootstraps as predispositions and pathways to guide the development of knowledge (not as built-in representations or knowledge). Predispositions support and constrain subsequent acquisition of mathematical knowledge, but they do not determine its development. Otherwise it would not be plausible to assume different developmental courses.
To trace hypothetical learning trajectories (Sarama & Clements, 2009) could serve as a starting point for developmentally appropriate teaching and learning.
Early knowledge about quantities
Early sensitivity for numerosities
Several studies reveal a remarkable sensitivity for numerosities in children who are a few months old. For one to three elements, infants realise whether two collections of elements are equal or different in number; the elements may be objects, movements or sounds. Infants are surprised, if they were shown impossible outcomes for simple additions, and/or subtractions, using one and two elements.
Early sensitivity has frequently been explored within a habituation paradigm. Infants are shown a sequence of pictures, differing in some ways, but always displaying the same number of objects, two for example. After a while infants get used to it and their attention wanes. But as soon as a picture of three objects appears it has their full attention (preference for novelty) (Starkey & Cooper, 1980; Antell & Keating, 1983).
Infants respond with enhanced attention when shown an impossible event (referred to as the violation of expectation). There is one doll and a second doll is added. If, as a result, only one doll can be seen, the infants look at this impossible outcome for longer than the possible outcome. Likewise, infants are surprised, if one of two dolls is taken away and two dolls still remain (Wynn, 1992a).
If three elements are a novelty for infants after getting used to two elements, in some way, infants must be able to compare these two collections of elements. Because the two collections of elements are displayed one after another, infants have to build up a mental representation of the habituated stimulus, i.e. the two elements. They have to compare their representation of the two elements with the novelty of three elements.
At the age of six months, infants are also able to discriminate three jumps of a doll from two jumps (Wynn, 1996). Infants, who are a few-days-old, perceive two sounds (syllables) as being substantively different from three sounds (syllables), even if there is no difference in duration (Bijeljac-Babic, Bertoncini & Mehler, 1993).
Subsequent research has failed to replicate the reported results reliably. Findings are inconsistent in this regard. For a replication of Wynn (1996), with different results, see Clearfield (2004); Clearfield & Westfahl (2006). But results could be re-affirmed if particular account is taken of perceptual cues, unrelated to number, that are known to influence looking preference experiments (Farzin, Charles & Rivera, 2009).
It can be argued that infants do not discriminate the numbers of elements, but only their spatial or temporal circumference (Feigenson, Carey & Spelke, 2002). This might be true, if dots are compared to dots, and sounds are compared to sounds. But what cannot be explained is the correspondence between elements of different perceptual modality, such as objects and sounds (Starkey, Spelke & Gelman, 1990). Seven-month-old infants preferred correspondence between number of women seen and voices heard (Jordan & Brannon, 2006). More likely, a representation of discrete elements or occurrences permits one-to-one correspondence, irrespective of their type. Recent research confirms infants' use of, or even preference for, the discrete and exact representation of a limited number of elements up to three (Cordes & Brannon, 2008a; 2008b).
To assess the changes in number (Wynn, 1992a), infants need representations of the discrete elements (see also nonverbal mental models), and they have to understand the sequence of actions. Infants have to retain the initial number of objects (one or two) mentally, because the objects are hidden. The representation has to be changed according the change occurring in the real situation (one object is added or one object is removed) (see also Feigenson, Carey & Spelke, 2002). This could explain the astonishment (i.e. increased attention) of the infant facing an impossible and unexpected outcome (Figure 1 shows the sequence of events).
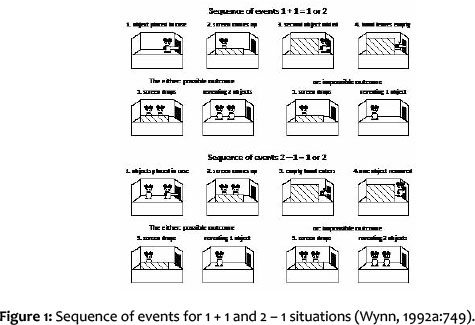
Infants can discriminate or match precisely collections of one to three elements. Their ability is limited, however, to those small numerosities (Starkey & Cooper, 1980; see also subitizing). Although early sensitivity for numerosities in infants can be taken as a certainty, it is not easy to grasp the underlying concepts and processes.
To learn more about infants' representations of number, Feigenson, Carey & Hauser (2002) worked with older infants (10 and 12 months old), and applied a choice task. Crackers were put one by one in two containers. The infants crawled to either one of the containers and were allowed to keep the crackers from the container they had chosen. The crackers were only visible when they were put in the containers. Infants were successful in choosing the larger quantity if they had to compare 1 with 2 crackers or 2 with 3 crackers. But they could not manage to compare 3 with 4, 2 with 4 and 3 with 6 crackers (what they could do easily if the crackers were visible). The infants spontaneously made more/less comparisons. Because the objects were hidden, they must have been able to represent more/less relationships, but were limited on set-sizes for up to three elements.
Feigenson et al. (2002), propose a representational system that allows for the tracking of one to three individual elements, and the parallel individuation of at least one other collection of one to three elements. One-to-one-correspondence is used for comparisons. It can be suggested that such a representational system underlies the performance of other numerical tasks.
But infants are also able to discriminate collections of four or more elements. The discriminability of the collections depends on the ratio and not on the absolute difference. Xu & Arriaga (2007) investigated development in large number discrimination and reported increasing precision. Whereas six-months-old infants could discriminate 4 elements from 8 elements, 8 elements from 16 elements (ratio 1 : 2), but not yet 4 from 6 or 8 from 12 elements (ratio 2 : 3), 10-months-old infants already managed the ratio 2 : 3, but could not discriminate collections of 8 elements from those with 10 (ratio 4 : 5). At about the age of 5, children come up to the speed and accuracy of adults (adults are able to discriminate numerosities by ratio 1 : 1,5) (Halberda & Feigenson, 2008; Huntley-Fenner, 2001).
Findings also reveal that infants perform similarly with intermodal numerical discrimination of larger collections: six-month-old infants can compare approximate numerical representations of auditory input with approximate numerical representation of later presented visual input (Feigenson, 2011). Xu & Arriaga (2007), in systematically varying continuous extent, and Feigenson (2011), in varying the perceptual modality, ensure that infants' representation of discrete numbers is captured.
These findings suggest that children dispose of two distinct systems for representing numbers, an object file system and an analogue magnitude system (or approximate number system). Infants successfully discriminate small sets from small sets and large sets from large sets; whereas, they fail at discriminating small sets from large sets (Feigenson & Carey, 2005; but see Wynn, Bloom & Chiang, 2002). These findings further support the idea of there being two distinct systems of numerical representation.
Cordes & Brannon (2009) found that the ratio determines when infants were able to discriminate small sets from large sets. Seven-month-old infants were able to differentiate between 1 and 4 elements and between 2 and 8 elements. A ratio of 1 : 4 was required, a ratio of 1 : 2 was not sufficient for successful discrimination. To explain their findings, Cordes & Brannon (2009) proposed a threshold hypothesis. Infants represent small sets with both object files and analogue magnitude representation (approximate number representation). Object files are the preferred representation for comparisons in which small sets are involved. But, if the two collections to be compared (a small and a large set) differ by a critical ratio (the threshold); the analogue magnitude representation is used. Threshold hypothesis undertakes not only continuity across the number line, but also across development (infants and adults using analogue magnitude representation for small sets).
Carey (2011) refers to the two distinct systems object file system and analogue magnitude system (or approximate number system) as constituting core cognition. In her understanding, mathematical core cognition comprises two systems of representation with numerical content: parallel individuation of small sets of entities in working memory (like the object file system), and analogue magnitude representation of number. Carey (2011) characterises the systems as being innate and conceptual. Carey (2011) assumes discontinuities in conceptual change from preverbal to verbal numerical concepts. Gopnik (2011), however, questions the innateness of the representational systems. The systems also could be acquired very early (for construction of number concepts, see also Sarnecka, Goldman & Slusser, in press). Gelman (2011), on the other hand, defends continuity in conceptual development and describes the continuity in concepts from preverbal to verbal and from infancy to adulthood.
Nonverbal mental models
Mental models and object files are both grounded in object individuation. Mental models may be seen as more abstract representations of collections of elements. Irrelevant characteristics (form, size, or colour) are left out, whereas, relevant numerical information is mapped with precision.
Huttenlocher, Jordan & Levine (1994) used matching and calculation tasks to explore mental models of children aged 2 years and 6 months to 3 years and 11 months. In the matching tasks, children were presented with collections of 1 to 5 objects (little disks) that were afterwards covered up. Children had to produce the matching number of disks. Of the youngest children (2;6 to 2;8 years), 90% could manage to match 2 objects, 37% matched 3 objects, 27% were successful with 4 objects and 20% even with 5 objects. Among the oldest children (3;9 to 3;11 years), 97% could manage to match 2 objects, 84% matched 3 objects, 57% matched 4 objects and 30% even 5. To solve this task the children had to create a mental representation of the objects.
In the calculation tasks, objects were again placed and hidden (for example 3 disks). After this, 1 or 2 objects were added to the hidden ones or removed from them. The children had to put down the resulting number of objects. To meet this demand, children had to represent the given quantity and to change the representation according to the changes of the real quantity. The generated representation can be used to produce the result.
Among the youngest children, 30% could determine the result for 2 - 1, 17% worked out the result for 1 + 3 and 13% could solve the task 3 - 2. Performance improved in the calculation tasks as well. Among the oldest children, already 70% could find the result for 2 - 1, 67% solved 3 - 2 and 43% produced the correct solution for 1 + 3.
Successful modelling depends on the number of objects that must be represented and the sort of transformation (addition or subtraction). Klein & Bisanz (2000) refer to the number of objects as the representational set size. For additions, it is the size of the sum, and for subtractions, it is the size of the minuend. In their study with four-year-old children, they could show a correlation between solution frequency and representational set size. This relationship provides further evidence of children's use of mental models in this context. Children could solve these nonverbal problems earlier than comparable verbal problems (Jordan, Huttenlocher & Levine, 1992).
Subitizing
Subitizing is the immediate apprehension of small numerosities (Fuson, 1992). Only one glance is needed to know the size of the set. Subitizing is rapid and accurate for set sizes from 1 to 4 (5). A typical test procedure will be to show an unstructured set of dots for a short time - too short to count them. Another possibility is to measure response time for enumeration.
Chi & Klahr (1975) found out that the time needed to recognise set sizes from 1 to 4 is always the same. Five-year-old children are as fast as adults. Larger set sizes require longer response time, with time increasing considerably with every additional element. As regards larger sets, adults are faster than children. This supports the conclusion that children are able to subitise collections for up to 4 elements. And that is exactly what adults are able to. Larger collections of elements have to be enumerated, and adults are faster at enumeration.
Starkey & Cooper (1995) explored the development of subitizing. The sets were shown for a very brief period of time (200 ms) to prevent counting. Two-year-old children subitised 1 to 3 elements, three-year-old children subitised 1 to 4 elements, four- to five-year-olds subitised 1 to 5 elements.
The maximum number of simultaneously recognisable elements alternates between 4 and 5 elements across various studies. The variations could be explained by marginally shorter presentation times that allow for a kind of quasi-simultaneous apprehension (parts of the collection of 5 are subitised, for example 2 elements and 3 elements, and then added up).
It can be assumed that numerical facts are discovered within collections of 1 to 4 elements, and then transferred to larger numbers. Sarnecka & Carey (2008) identify subset-knowing (1 to 4 elements known without counting) as prerequisite for cardinal knowing (see cardinality concept).
Spontaneous focusing on numerosity
Some children seem to perceive numerical features of objects and situations easily and often, thereby exercising early mathematical skills, such as subitizing and counting. Others pay more attention to other features of objects and situations and are much less involved in early mathematical activities. The extent to which children spontaneously focus on numerositiy and practise their numerical abilities could explain developmental differences in early mathematical skills (Hannula, Räsänen & Lehtinen, 2007).
In their study with three- to seven-year old children, Hannula & Lehtinen (2005) observed a steady tendency in spontaneous focusing on numerosity (SFON) over different tasks, and over time. They found a positive correlation between spontaneous focusing on numerosity and counting abilities. Children differ in their sensitivity for opportunities. In their study with four- to five-year old children, Hannula, Räsänen & Lehtinen (2007) found correlations between focusing on numerosities, subitizing and counting abilities.
Kindergarten children's individual differencesin spontaneous focusing on numerosity predict arithmetical skills in school two years later (Hannula, Lepola & Lehtinen, 2010). Hanula et al. (2010) conclude that the tendency of spontaneous focusing on numerosity is a domain specific predictor.
Protoquantitative schemata
Protoquantitative schemata comprise foundational knowledge about quantities. Children dispose of protoquantitative schemata before being able to determine numerosities precisely through counting (see also early sensitivity for numerosities, Hannula, Räsänen & Lehtinen, 2007). Resnick (1992; Resnick & Singer, 1993) characterises protoquantitative schemata as intuitive knowledge, which means that children construct this knowledge out of their everyday experience. Protoquantitative schemata allow for non-numerical reasoning about relations among amounts. Those relational schemata may be applied subsequently to numerosities and furthermore to numbers. Resnick (1992) specifies three different types of protoquantitative schemata: compare schema, increase/decrease schema, and part-whole schema.
• Compare schema: Children are able to compare two collections of objects and to decide which collection contains more or less elements. For small sets of items the comparison must be precise from the beginning. For large set sizes, estimations are sufficient. It can be assumed that on their way to exact numerical comparisons, children first discover the compare schema for small numerosities (1 to 4, by means of subitizing) and carry it over to larger quantities (quantities defined by means of counting).
• Increase schema and decrease schema: If elements are added to a certain amount of elements, the amount increases. If elements are taken away, the amount decreases (inversion of addition and subtraction). Imagine a bag of marbles: without seeing the marbles inside the bag, you know that if some marbles are added, there will be more marbles in the bag than before. If some marbles are removed, there will be less marbles in the bag than before (Resnick & Singer, 1993).
• Part-whole-schema: You also know that a constant amount of marbles is still the same amount, even if divided between two bags, and that this applies to all possible distributions of a constant amount of marbles. Furthermore, you know that transferring some marbles from one bag to the other leaves the whole amount unchanged (compensation). But if there are additional marbles put into one of the bags, the whole amount increases. If marbles are removed from one bag, the whole amount decreases (covariation) (Resnick & Singer, 1993).
By the age of two years, children have a protoquantitative comparison schema, and children dispose of a protoquantitative increase/decrease schema by the age of three years. The protoquantitative part-whole schema follows when children are about four years old.
Protoquantitative reasoning needs no numerical quantification. However, protoquantitative reasoning does not disappear with progressive numerical development. Protoquantitative reasoning is still useful, for example, in complex situations (Resnick & Singer, 1993).
Cardinality concept
Although correct enumeration is essential to find out the exact number of elements in a set, it is not enough to define cardinal understanding (Sarnecka & Carey, 2008). For correct enumeration, the three how-to-count-principles (Gelman & Gallistel, 1978) have to be followed: one-to-one-correspondence between items and numerals (number words), stable order of the numerals, and the cardinal principle (or last-word-rule).
One-to-one principle holds that one, and only one, numeral has to be assigned to each element of a collection. Stable order of the numerals holds that with every enumeration the numerals have to be used in the same order. Cardinal principle holds that the numeral assigned to the last element of the collection represents the number of elements in the collection (Gelman & Gallistel, 1978; Sarnecka & Carey, 2008).
Even two-year-old children, when starting to enumerate elements, are able to apply these principles. Beyond this there is hardly any evidence to confirm a principles-first view. Research results rather prove a principles-after view. The how-to-count principles are gradually learned, as can be seen from the mistakes that children make. At the beginning, children skip or double-count elements, they produce different numeral lists at different times (Fuson, 1988).
If children apply these principles properly and answer the how-many-question (how many marbles are there?) correctly, it can only be stated that they master the last-word-rule. Nothing can be known about their cardinal understanding.
To approximate an appropriate measure of cardinal understanding, the give-a-number task (give three marbles to the puppet) can be used (Sarnecka & Carey, 2008; Wynn, 1992b). Six performance levels in give-a-number tasks can be observed: prenumeral-knower-level, one-knower-level, two-knower-level, three-knower-level, four-knower-level, cardinal-principle-knower-level (Sarnecka & Carey, 2008; Wynn, 1992b).
Children at prenumeral-knower-level give always one or always a handful of marbles to the puppet. The number of given elements is not related to the number asked. One-knowers (children reach that level at about 2 years and 6 months to 3 years) give one marble when they are asked for one marble, but for all other numerals, the child gives two or more elements irrespective of the number asked. One-knowers know that one means one. Only a few months later, children become two-knowers; they give one marble if one marble is requested, and give two marbles if two marbles are requested. But again they cannot distinguish between numerals that are above their level. Asked to give 3, 4 or 5 marbles they simply grab some marbles. Two-knower-level is followed by three-knower-level. In some studies there is also a four-knower-level. Children on all knower-levels are called subset-knowers (Sarnecka & Carey, 2008). From 1 to 3 or 4 the meaning of each numeral is learned one after the other. But from that experience, children seem to discover the general principle and at once are able to give 5 or 6 or more requested marbles and are able to generate the cardinality for numerals 5, 6 and above.
Sarnecka & Carey (2008) describe differences between subset-knowers and cardinal-principle-knowers. Subset-knowers are not counting the elements they give while cardinal-principle-knowers count them. For this reason, Wynn (1992b) referred to the subset-knowers as grabbers and to the cardinal-principle-knowers as counters (see also the relation between subset-knowing and subitizing).
Sarnecka & Carey (2008) found that subset-knowers, as well as cardinal-principle-knowers were able to count up at least to 8 and to enumerate sets of elements (nearly all children were successful at a one-to-one correspondence task, most two-knowers had already learned the last-word-rule). But only cardinal-principle-knowers knew that adding elements to a collection means moving forward in the list of numerals, and that subtracting elements means moving backwards (direction task). Only cardinal-principle-knowers understood the unit of change task. Adding one object means moving one word, and adding two objects means moving two words (successor function). Only cardinal-principle-knowers understand equinumerosity (Sarnecka, Goldman, & Slusser, in press). At the same time, the findings reveal components of cardinality (Sarnecka & Carey, 2008).
As can be seen, children develop the number word sequence, enumeration and cardinal understanding by and by, while the relationships between these concepts and procedures become increasingly complex (Fuson, 1992).
First, the numeral list (number word sequence) is unbreakable (Fuson, 1992) and can be used to enumerate perceptible elements - perceptual counting scheme (Steffe, 1992). Counting (saying the number word sequence) and enumeration (counting elements), always start with one. Enumeration entails a one-to-one-correspondence.
Later on, the numeral list can be seen as a breakable chain (Fuson, 1992). Children at about four years are able to start counting from any numeral. If there are 2 sets, and the total number of both sets should be determined, children can count on from one addend: There are 3 marbles and there are another 2 - '3, 4, 5' - there are 5 marbles. The 3 marbles must be seen as cardinality and embedded in the whole (Fuson, 1992). Numerals can be related to representations of elements - figurative counting scheme (Steffe, 1992; see also non-verbal mental models, Huttenlocher, Jordan & Levine, 1994). Fingers can also be used to represent invisible elements.
When children are about five years old, they no longer need concrete elements to enumerate. Numerals themselves are enumerable - numerable chain level (Fuson, 1992). Steffe (1992) refers to the correspondent counting schema as the initial number sequence. It is followed by the tacitly nested number sequence. Numerals can be understood as units consisting of units. The unit 5 consists of 5 single units (Steffe, 1992).
As soon as children can see 5 as a part of 6, 5 is included in 6, the underlying counting scheme is the explicit nested number sequence (Steffe, 1992). Bidirectional-chain-level or truly numerical counting is the corresponding level of numeral sequence (Fuson, 1992). The numeral sequence is completely flexible in both directions. Numbers can be seen as composed of other numbers; 7 is composed of 3 and 4, but also 5 and 2 or 6 and 1.
Part-part-whole concept
Part-part-whole schema posits that numbers are composed of other numbers (Resnick, 1992). Remember the marbles in the bag? Now, imagine you can see them and you can enumerate them. Suppose there were 7 marbles in the bag. You divide them between 2 bags. You know that there are still 7 marbles together in the 2 bags and that this applies to all possible distributions of the 7 marbles (3 and 4, 5 and 2, 6 and 1). Imagine now in one bag there are 4 marbles, and in the other bag are 3. Put 2 of the 4 marbles in the bag with the 3 marbles. How many marbles are in your 2 bags combined? You know that moving 2 marbles from one bag into the other leaves the quantity of 7 unchanged (compensation). But, imagine 2 additional marbles are put in one of the bags, what will happen? Or, what will happen if 2 marbles are removed from one bag (covariation)?
Part-part-whole concept provides the foundation for flexible and understanding arithmetical strategies. Given the task 3 + 4: children know that 3 + 3 = 6 and they know 4 is 1 more than 3. So they know (covariation) that the result must be 1 more than 6, which means, 7. Or given the task 6 + 7: children know that 6 is composed of 5 and 1, 7 is composed of 5 and 2. Virtually, without calculating, children can see 5 and 5 makes 10, 1 and 2 makes 3, and the result is 13.
Inversion of addition and subtraction can be easily understood with knowledge of part-part-whole as a basis. If children know that 7 can be divided in 3 and 4 (7 is the whole, 3 and 4 are the parts), they can solve many addition and subtraction tasks:
3 + 4 = ?; 4 + 3 = ?; 7 - 4 = ?; 7 - 3 = ?; 3 + ? = 7; 4 + ? = 7; ? + 3 = 7; ? + 4 = 7; 7 - ? = 3; 7 -? = 4...
The part-part-whole concept is also helpful when solving word problems. It always seems to be difficult for children to choose the right operation (for example, addition or subtraction), in order to find the result. Here is an example:
Lisa had 3 cookies, Tom gave her some more, now she has 7 cookies. How many cookies has Tom given to Lisa?
The part-part-whole concept is an essential prerequisite for place-value system for the understanding of fractions and further arithmetical concepts where parts and wholes play an important role.
Conclusion
The development of early numerical skills has been described as an integration of knowledge about quantities and knowledge about numbers (Figure 2, on the following page, shows the development and interdependency of numerical concepts). The concepts of cardinality and part-part-whole have proven to be key concepts of numerical knowledge. Developmentally appropriate learning and teaching should focus on these concepts.

The concepts of cardinality and part-part-whole are the centre-pieces in the MARKO-T (Math and Arithmetic Training on Concepts in Pre-school and Primary School Age). Fritz, Ehlert & Balzer (in this volume), describe their empirically tested model of numerical development. It provides the foundation for MARKO-D (Math and Arithmetic Test for Assessing Concepts at Pre-school Age) and for MARKO-T.
References
Antell, S.E. & Keating, D.P. 1983. Perception of numerical invariance in neonates. Child Development 54:695-701. [ Links ]
Baroody, A.J. 2004. The developmental bases for early childhood number and operations standards. In D. H. Clements, J. Sarama & A.-M. DiBiase (Eds.), Engaging young children in mathematics: Standards for early childhood mathematics education, pp. 173-219. Mahwah, NJ: Erlbaum. [ Links ]
Bijeljac-Babic, R., Bertoncini, J. & Mehler, J. 1993. How do four-day-old infants categorize multisyllabic utterances. Developmental Psychology 29:711-721. [ Links ]
Carey, S. 2011. Precis of the origins of concepts. Behavioral and Brain Sciences 34:113-167. [ Links ]
Chi, M.T.H. & Klahr, D. 1975. Span and rate of apprehension in children and adults. Journal of Experimental Child Development 19:434-439. [ Links ]
Clearfield, M.W. 2004. Infants' enumeration of dynamic displays. Cognitive Development 19:309-324. [ Links ]
Clearfield, M.W. & Westfahl, S.M-C. 2006. Familiarization in infants' perception of addition problems. Journal of Cognition and Development 7:27-43. [ Links ]
Cordes, S. & Brannon, E.M. 2008a. Quantitative competencies in infancy. Developmental Science 11:803-808. [ Links ]
Cordes, S. & Brannon, E.M. 2008b. Representing continuous extent in infancy: Using number is just easier. Child Development 79:476-489. [ Links ]
Cordes, S. & Brannon, E.M. 2009. Crossing the divide: Infants discriminate small from large numerosities. Developmental Psychology 45:1583-1594. [ Links ]
Chen, Z. & Siegler, R. 2000. Overlapping waves theory. Monographs of the Society for Research in Child Development 65(2):7-12. [ Links ]
Farzin, F., Charles, E.P. & Rivera, S.M. 2009. Development of multimodal processing in infancy. Infancy 14:563-578. [ Links ]
Feigenson, L. 2011. Predicting sights from sounds: 6-month-olds' intermodal numerical abilities. Journal of Experimental Child Psychology 110:347-361. [ Links ]
Feigenson, L. & Carey, S. 2005. On the limits of infants' quantification of small object arrays. Cognition 97:295-313. [ Links ]
Feigenson, L., Carey, S. & Hauser, M. 2002. The representations underlying infants' choice of more: object file versus analog magnitudes. Psychological Science 13:150-156. [ Links ]
Feigenson, L., Carey, S. & Spelke, E. 2002. Infants' discrimination of number vs. continuous extent. Cognitive Psychology 44:33-66. [ Links ]
Fuson, K.C. 1988. Children's counting and concepts of number. New York: Springer. [ Links ]
Fuson, K. 1992. Relationships between counting and cardinality from age 2 to age 8. In J. Bideaud, C. Meljac & J.-P. Fischer (Eds.), Pathways to number, pp. 127-149. Hillsdale, NJ: Erlbaum. [ Links ]
Gelman, R. 2011. The case for continuity (open peer commentary to Carey). Behavioral and Brain Sciences, 34 127-128. [ Links ]
Gelman, R. & Gallistel, C.R. 1978. The child's understanding of number. Cambridge, MA: Harvard University Press. [ Links ]
Gopnik, A. 2011. A unified account of abstract structure and conceptual change: Probabilistic models and early learning mechanisms (open peer commentary to Carey). Behavioral and Brain Sciences 34:129-130. [ Links ]
Halberda, J. & Feigenson, L. 2008. Developmental change in the acuity of the 'number sense': The approximate number system in 3-, 4-, 5-, and 6-year-olds and adults. Developmental Psychology 44:1457-1465. [ Links ]
Hannula, M.M. & Lehtinen, E. 2005. Spontaneous focusing on numerosity and mathematical skills in young children. Learning and Instruction 15:237-256. [ Links ]
Hannula, M.M., Lepola, J. & Lehtinen, E. 2010. Spontaneous focusing on numerosity as a domain-specific predictor of arithmetical skills. Journal of Experimental Child Psychology 107:394-406. [ Links ]
Hannula, M.M., Räsänen, P. & Lehtinen, E. 2007. Development of counting skills: role of spontaneous focusing on numerosity and subitizing-based enumeration. Mathematical Thinking and Learning 9:51-57. [ Links ]
Huntley-Fenner, G. 2001. Children's understanding of number is similar to adults' and rats'. Numerical estimation by 5-7-year olds. Cognition 78:B27-B40. [ Links ]
Huttenlocher, J., Jordan, N.C. & Levine, S.C. 1994. A mental model for early arithmetic. Journal of Experimental Psychology: General 123:284-296. [ Links ]
Jordan, K.E. & Brannon, E.M. 2006. The multisensory representation of number in infancy. Proceedings of the National Academy of Sciences 103:3486-3489. [ Links ]
Jordan, N.C., Huttenlocher, J. & Levine, B.C. 1992. Differential calculation abilities in young children from middle- and low-income families. Developmental Psychology 28:644-653. [ Links ]
Karmiloff-Smith, A. 1992. Beyond modularity. A developmental perspective on cognitive science. Cambridge, MA: MIT Press. [ Links ]
Klein, J.S. & Bisanz, J. 2000. Preschoolers doing arithmetic: the concepts are willing but the working memory is weak. Canadian Journal of Experimental Psychology 54:105-115. [ Links ]
Resnick, L.B. 1992. From protoquantitaties to operators: Building mathematical competence on a foundation of everyday knowledge. In G. Leinhardt, R. Putnam & R. A. Hattrup (Eds.), Analysis of arithmetic for mathematics teaching, pp. 373-429. Hillsdale, NJ: Erlbaum. [ Links ]
Resnick, L.B. & Singer, J.A. 1993. Protoquantitative origins of ratio reasoning. In T.P. Carpenter, E. Fennema & T.A. Romberg (Eds.), Rational numbers: An integration of research, pp. 107-130. Mahwah, NJ. Erlbaum. [ Links ]
Sarama, J. & Clements, D.H. 2009. Early childhood mathematics education research. Learning trajectories for young children. New York, NY: Routledge. [ Links ]
Sarnecka, B.W. & Carey, S. 2008. How counting represents number: What children must learn and when they learn it. Cognition 108:662-674. [ Links ]
Sarnecka, B.W., Goldman, M.C. & Slusser, E.B. (in press). How counting leads to children's first representation of exact, large numbers. In R. Cohen Kadosh & A. Dowker (Eds.), Oxford Handbook of Numerical Cognition. Oxford: Oxford University Press.
Siegler, R.S. 1996. Emerging minds: The process of change in children's thinking. Oxford: Oxford University Press. [ Links ]
Siegler R.S. & Alibali, M.W. 2005. Children's thinking. Englewood Cliffs, NJ: Prentice Hall. [ Links ]
Starkey, P. & Cooper, R.G. 1980. Perception of numbers by human infants. Science 210:1033-1035. [ Links ]
Starkey, P. & Cooper, R.G. 1995. The development of subitizing in young children. British Journal of Developmental Psychology 13:399-420. [ Links ]
Starkey, P., Spelke, W.S. & Gelman, R. 1990. Numerical abstraction by human infants. Cognition 36:97-127. [ Links ]
Steffe, L.P. 1992. Learning stages in the construction of the number sequence. In J. Bideaud, C. Meljac & J.-P. Fischer (Eds.), Pathways to number, pp. 83-98. Hillsdale, NJ: Erlbaum. [ Links ]
Wynn, K. 1992a. Addition and subtraction by human infants. Nature 358:749-750. [ Links ]
Wynn, K. 1992b. Children's acquisition of number words and the counting system. Cognitive Psychology 24:220-251. [ Links ]
Wynn, K. 1996. Individuation and enumeration of actions. Psychological Science 7:164-169. [ Links ]
Wynn, K., Bloom, P. & Chiang, W. 2002. Enumeration of collective entities by 5-month-old infants. Cognition 83(3):B55-B62. [ Links ]
Xu, F. & Arriaga, R.I. 2007. Number discrimination in 10-month-old infants. British Journal of Developmental Psychology 25:103-108. [ Links ]














