Services on Demand
Article
Indicators
Related links
-
 Cited by Google
Cited by Google -
 Similars in Google
Similars in Google
Share
Water SA
On-line version ISSN 1816-7950
Print version ISSN 0378-4738
Water SA vol.45 n.3 Pretoria Jul. 2019
http://dx.doi.org/10.17159/wsa/2019.v45.i3.6698
RESEARCH PAPERS
Plantwide modelling - anaerobic digestion of waste sludge from parent nutrient (N and P) removal systems
DS Ikumi*; GA Ekama
Water Research Group, Department of Civil Engineering, University of Cape Town, Rondebosch, 7700, Cape Town, South Africa
ABSTRACT
Wastewater treatment plant (WWTP) mathematical models are based on the behavioural patterns of microorganisms involved in the treatment process. These microorganisms are assumed incapable of thinking or planning but simply act according to the capabilities afforded to them by their surrounding conditions - hence different microorganisms pre-dominate different WWTP zones according to how well the conditions suit them. When waste activated sludge (WAS) from biological nutrient removal (BNR) activated sludge (AS) systems, containing phosphorus-accumulating organisms (PAOs), is fed to an anaerobic digester, there is a release of high quantities of metals, phosphorus (P) and nitrogen (N). The manner in which we model the release of these metals and nutrients significantly affects the accuracy of predicted anaerobic digestion (AD) outcomes. Previous studies of PAOs show that in the anaerobic zone of the AS system, they can form energy-rich poly3-hydroxybutyrate (PHB) at the expense of their aerobically generated polyphosphate (PP). Thus, it is expected that the PAOs containing PP sent into an anaerobic digester with volatile fatty acids (VFAs) present, would utilize their PP reserves as they would in the anaerobic zone of an AS process ending up with formation and storage of some PHB. Ultimately, all the stored products of the PAO get released, since there is no alternating aerobic environment to cater for their growth. Since it has been established that the PP release in the AD occurs much faster than the PAO biomass hydrolysis rate, it is modelled as a separate process. Steps are presented in the development of this PP release mass-balanced stoichiometries that occur with AD of PAOs. By comparing outcomes from these proposed stoichiometries against measured experimental data, it is noticed that better predictions are obtained with acetate uptake for PHB formation than when modelling the AD PP release to occur with PAO death and hydrolysis.
Keywords: phosphorus removal, activated sludge, anaerobic digestion, plant-wide modelling
INTRODUCTION
The drive to recover phosphorus (P) and predict potential problems in wastewater treatment plant (WWTP) systems, such as struvite precipitation and pH changes in various unit processes, has led to extensive research into P removal in WWTPs. Phosphorus is removed from wastewater by transforming it from the dissolved liquid phase to the intracellular solid phase. Hence it was noted that for the AD of P-rich sludge, from biological excess P removal (EBPR) activated sludge (AS) systems, three-phase mixed weak acid/base chemistry is required because the release of biomass P or polyphosphate (PP) not only affects the system alkalinity but also can induce precipitation of minerals such as struvite (Van Rensburg et al., 2003; Harding et al., 2010). Batstone et al. (2012) outline the methods, available knowledge and the fundamental approaches towards the development of a physicochemical framework that supports the systematic development of such three-phase plant-wide models. This approach was applied by Lizarralde et al. (2015) in the development of a new general methodology for incorporating physicochemical and chemical transformations into multi-phase plant-wide models. Also, various research groups have worked both collaboratively and separately on related topics, towards the development of WWTP mathematical models that integrate bioprocess stoichiometry and physicochemical transformations, such that processes such as nutrient release and multiple mineral precipitations (MMP) can be included.
Related to this is the progress made on extending the Anaerobic Digestion Model No. 1 (ADM1; Batstone et al., 2002), for the inclusion of P removal and associated processes. Flores-Alsina et al. (2016) presents ADM1 with an improved physicochemical description, P and sulphur (S) biotransformation, iron (Fe) reduction and multiple mineral precipitation (MMP) to show the effect of including the interactions amongst P, S, Fe and observing their potential effect on predicted model outcomes, such as total biogas production (CO2, CH4, H2 and H2S). The MMP component of this model was included according to the semi-mechanistic modelling approach of Kazadi Mbamba et al. (2015). Further extensions to ADM1 to accommodate P include studies by Wang et al. (2016), for including phosphorus-accumulating organisms (PAOs) uptake of various volatile fatty acids (VFAs) for the formation of poly3-hydroxybutyrate (PHB), the effect of resultant PHB content on disintegration rate and the potential precipitation of the P (mostly released with the anaerobic PHB uptake). Bioprocess stoichiometry and physicochemical transformations are also included in the presentation of a new 'three-phase' (aqueous-gas- solid) plant-wide model that includes P, (PWM_SA) (Brouckaert et al., 2010; Ikumi et al., 2015) which includes compatible activated sludge (AS; ASM2-3P) and anaerobic digestion (AD; SDM3P) bioprocess model components and uses strict mass balance principles to track P through the unit processes of a WWTP (with recognition of its impact on the mutual interaction between the connected unit operations). The AD component of PWM_SA extends the AD model of Sötemann et al. (2006) by including multiple organic types (including WAS with PAOs), P release stoichiometry and multiple mineral precipitation. When including the behaviour of PAO biomass in AD, Ikumi (2011) concluded that most of the P release occurred with acetate uptake and PHB formation, which posed the question of whether there is any energy transfer between the AS and AD systems with PP release and, if so, how this energy transfer should be represented in AD. The significance of this energy representation in AD systems is highlighted by the impact of the PP release process towards the determination of system pH and carbon dioxide partial pressure (pCO2), which are required to be accurate for prediction of mineral precipitation potential and concentrations in the AD aqueous phase. This paper investigates the appropriate stoichiometry for this PP release process, using results from the investigation of an enhanced biological P removal (EBPR) AS system linked to an AD.
METHODS
In the study by Ikumi (2011), a University of Cape Town (UCT) NDEBPR system was operated and fed 600 mgCOD/L settled wastewater together with additives (i.e., 200 mgCOD/L acetate and 40 mgP/L from di-potassium hydrogen phosphate (KH2PO4)). This UCT AS system was operated at steady state, at a 10-day system sludge age (SRT) and its WAS was anaerobically digested in a separate flow-through anaerobic digestion (AD) system operated successively at different solids retention times (SRTs) (Table 2). The experimental set-up of Ikumi (2011) is given in Fig. 1. The results from this experimental set-up are used to examine the transfer of P to the AD and the release of poly-phosphate (PP) in the AD fed the NDEBPR WAS.
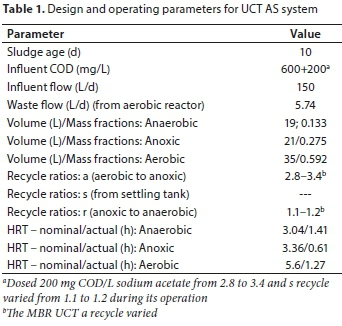
AD volume was always 16 L apart from 60 d AD which was operated using small 5 L AD volume reactors, The NDEBPR WAS taken from As system was 5.7 L/d at a concentration of about 9 gCOD/L. The required volume of this WAS was fed to the AD without thickening.
Model implementation
A three-phase plant-wide model (Ikumi et al., 2015) was developed for simulating the biological processes to track and predict the output of materials (chemical oxygen demand (COD), carbon (C), hydrogen (H), oxygen (O), nitrogen (N), phosphorus (P), magnesium (Mg), potassium (K) and calcium (Ca)) along the unit processes of a WWTP and tailored to conform to the laboratory AD and AD connected systems. The model comprises 3 sub-models, integrated for simulation of the entire WWTP comprising different unit operations (i.e., UCT NDEBPR AS system linked to an AD or anoxic-aerobic digestion (AAD) for WAS stabilisation). The sub-model components used to simulate the above experimental set-up include:
•The ionic speciation model (Brouckaert et al., 2010). This model includes the pairing of ionic components and inter-phase transfers of component species.
•The ASM2-3P model: This is the Activated Sludge Model No. 2 (ASM2, Henze et al., 1995), modified to include the ionic speciation model (Brouckaert et al., 2010), the inorganic settleable solids (ISS) model of Ekama and Wentzel (2004) and including multiple mineral precipitation according to Musvoto et al. (2000a,b).
•The SDM3P Model: This is the University of Cape Town Sludge Digestion Model 1 (UCTSDM1 (Sötemann et al., 2005)), modified to include the hydrolysis of multiple organic sludge types (primary sludge (PS), NDEBPR WAS and PS-WAS blends), the Ekama and Wentzel (2004) ISS model and the Brouckaert et al. (2010) speciation model which facilitates ionic speciation and multiple mineral precipitation.
The model evaluation procedure presented by Ikumi et al. (2015) was based on the BIOMATH protocol developed by Vanrolleghem et al. (2003). The complete description of the model (including components, processes and parameters) is reported in a previous publication of Ikumi et al. (2015). However, the new mass balanced stoichiometries of PP release (with the uptake of PHB and death of PAOs) that are used towards extension and modification of this model are evaluated below using the data from Ikumi (2011).
PAO behaviour in phosphorus removal unit processes
During the anaerobic phase of EBPR, the electrogenic release of PP occurs in conjunction with the production of protons to generate a proton motive force that energises the uptake of acetate to form poly3-hydroxybutyrate (PHB) (Van Veen et al., 1994). Noting that PHB is more of a reduced compound than acetate, it has been proposed that the reducing power for its synthesis is mainly from intracellularly stored glycogen (Mino et al., 1994; Smolders et al. 1995; Pereira et al., 1996) and also possibly from nicotinamide-adenine dinucleotide (NADH2) formed by the oxidation of acetate to carbon dioxide (CO2) (Comeau et al., 1985; Wentzel, 1988). However, during AD treatment of waste sludge (containing PAOs) from the aerobic reactor of the AS system the question arises: are the PAOs capable of carrying out the same P-release mechanisms in the AD as in the anaerobic reactor of the NDEBPR AS system? Essentially, microorganisms are assumed incapable of planning their actions based on a WWTP system configuration, and are expected to act according to the capabilities afforded to them by their surrounding environmental conditions. Thus, PAOs containing polyphosphate (PP) sent into an anaerobic digester with volatile fatty acids (VFAs) present, would utilize their PP reserves as they would in the anaerobic zone of an AS process. The PAOs are also expected to behave similarly in the absence of electron acceptors as regulated by the intracellular adenosine triphosphate (ATP), NADH2 and acetyl-CoA levels (or related ratios). This suggests that there is some potential for energy transfer from the AS to the AD via PP when WAS is treated in AD systems. However, the quantity of PP used as an energy source is known to vary according to the balance between energy generation and consumption in the cell (Smolders et al., 1995; Mino et al., 1994), a mechanism that is linked to the system pH - Smolders et al. (1995) observed changing P/acetate ratio (0.25 to 0.75) for varying pH ranges (5.5 to 8.5). However, the effect of pH on the EBPR stoichiometry is yet to be included in the current models.
After anaerobic P release and PHB uptake, the PAOs require a terminal electron acceptor (oxygen, usually supplied in the aerobic reactor of AS system) to utilise this PHB for growth and energy generation metabolism. However, oxygen is not available in AD and, in this case, the PAOs continue to release over time all remaining stored PP and PHB. From anaerobic batch tests on the NDEBPR WAS, Harding et al. (2010) noted that it took 5 to 8 days to release practically all the PP under anaerobic conditions. Whether this is faster than the death rate of the PAOs in the AD is uncertain, but it is significantly faster than AS biomass hydrolysis rate in the AD. Therefore, in the AD, both the PP release with PHB formation (as in the anaerobic reactor of the NDEBPR system) and PP release with the eventual death of PAOs takes place.
Ikumi (2011) explains the steps for the development of a generalised stoichiometry to model the release of PP, with the uptake of acetate (HAc), in the AD system. This includes:
(i) The use of enzymes to degrade PP (via hydrolysis), in the presence of adenosine diphosphate (ADP), for the release of phosphate and production of adenosine triphosphate (ATP, formed as an intermediate for utilization in energy-consuming processes and to drive some enzyme-controlled reactions) (Cole and Hughes, 1964; Smolders et al., 1995).(polyphosphate)n + ADP → ATP + (polyphosphate)n−1
Then ATP + H2O → ADP + H2PO4, ultimately:
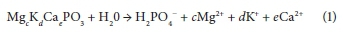
(ii) Anaerobic uptake of acetate (using the produced ATP, with energy) and its conversion to acetyl-CoA, which is subsequently converted to PHB with the use of NADH2 (formed as NADH-H+, when NAD+ molecules take up two electrons and two H+ atoms), as reported by Smolders et al. (1995) and also confirmed by Mino et al. (1994). Equation 2a provides a general reaction for anaerobic PP release:
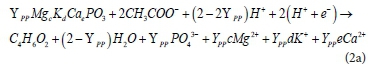
Where YPP is the mols of P released per mol of PHB formed. The uptake of H+ ions together with the electrons, during PHB formation, allows the PP release process to moderate anaerobic pH changes that occur with the release of orthophosphate (OP). The working AD pH is usually close to 7; hence mostly dissociated acetate is used in the AD PHB formation process. According to Wentzel et al. (1990), at a pH maintained at ≥ 7.25, 2 mg COD acetate is taken up per 1 mg P of PP released. Therefore, 2/64 mol of acetate is taken up per 1/31 mol of PP (hence for 1 mol of acetate taken up 64/(2 × 31) mol of P in PP is released). Since, in the tricarboxylic acid (TCA) cycle, 2 mol of acetate are used to form 1 mol PHB, 2 × (64/62) mol of P are released in the process. This is close to the observation of Smolders et al. (1995), where at a pH of 7 about 0.5 mol of P in PP are used to provide 0.5 mol ATP to form 1 mol of C in PHB. Hence, for 4 C mol of PHB (i.e., 1 mol PHB), 2 mol ATP are provided by the PP released (which is 2 mol P of PP). However, apart from the possibility of the acetate (anaerobically available) being used as the source of some of the H+ and e- for the PHB formation, Smolders et al. (1995) report that the main source of these electrons (and H+) is the aerobically generated glycogen (i.e., to act as a reducing agent). Since acetate uptake is linked to P release (for energy generation) and glycogen consumption (which maintains the redox balance), the quantity of glycogen available in PAOs becomes an important factor during the anaerobic PP release. With the PP release process taken as the reverse of its aerobic manufacture process, the energy content of PP could be determined to quantify the equivalent quantity of glycogen that is available for use in place of this energy. Aerobically, the PHB is used as the electron donor towards (i) anabolism of new PAO biomass, (ii) manufacture of glycogen, (iii) catabolism for the generation of energy, and (iv) uptake of P to form polyphosphate. Because both the PAO biomass and the glycogen stored in their cells are essentially organic components of PAO, we have to account for the quantity of glycogen that makes up part of the PAO organic mass in our models. This is such that Ybiomass + Yglycogen = YPAO = 0.66, where Yglycogen and Ybiomass are the yield coefficients for PHB converted to glycogen and biomass of PAOs, respectively. Smolders et al. (1995) estimated the yield value of PAO biomass (without the glycogen) to be about 13% lower than that of usual heterotrophic biomass. However, for the equivalent donating capacity of 2e- and 2H+, an extra 1/8 mol of glycogen (C4H7O3.5) is utilised in the process and less alkalinity gets generated.
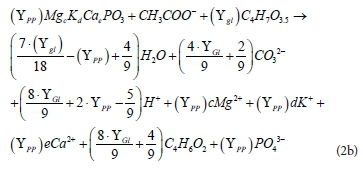
Where Ygl is the mols of glycogen used per mol of PHB formed, noting that the electrons of glycogen represent energy in PP transferred from the AS to AD. Since the molar electron donating capacity for glycogen, acetate and PHB is 16, 8 and 18, respectively, and 1 mol of acetate uptake releases 64/62 mol PP, then (1−Ygl)/8 mol acetate uptake results in (1−Ygl)∙(64/62) mol PP release. This is used in formulating the PP release and PHB formation stoichiometry below:
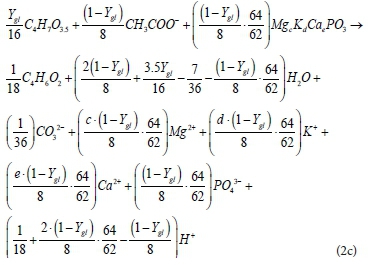
(iii) In the AD environment, the PAOs are not modelled to compete with the AD biomass for the acetate, but after an initial rapid uptake of acetate and release of PP, leave the remaining acetate to be degraded by AD biomass and give up the PHB with their hydrolysis. Thus, the PHB produced from the reaction of Eq. 2 is later broken down in AD, to form carbon dioxide together with some H+ and e-, as shown (Eq. 3). The products of this breakdown contribute (as with other biodegradable organics) towards AD biomass growth and energy (methane) generation:
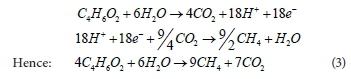
(iv) In the AD, the remaining PP that doesn't get released initially with PHB formation also eventually gets hydrolysed (as shown in Eq. 1a), since the dead PAO biomass cannot hold on to it further. In the aerobic digester, Vogts et al. (2014) observed the PP release with the death/endogenous respiration of PAO biomass to be slow (at a similar rate to the endogenous respiration process). However, in the AD the remaining PP is degraded faster than in the aerobic digester. Harding et al. (2010) provide a steady-state stoichiometric equation for this process:
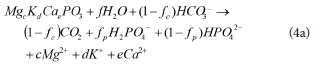
Where the f value fractionates the total phosphates to HPO42- and H2PO4-, according to pH, as shown by Harding et al. (2010), i.e.:
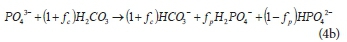
It was decided that both the PP release mechanisms in AD, i.e., with PHB formation (Eq. 2a, b and c) and with PAO death (Eq. 1), together with PHB disintegration (Eq. 3), be entered into the dynamic model because all have a possibility of occurrence. However, this may result in complexities for the calibration of their kinetics.
Ikumi (2011) accounts that the final outcome, for an AD system operated at steady state, is equivalent to the PP hydrolysis occurring with the death of PAOs, as was reported by Harding et al. (2010) (see Eqs 4a and 4b). This is because in the AD model, the PHB formed eventually gets completely degraded, hence all the COD removed in AD is ultimately destined for conversion to an inevitable quantity of AD biomass and biogas (some electrons go towards CH4), irrespective of the defined form of the biomass (i.e., the active PAOs are modelled with or without inclusion of glycogen). The discrepancies observed during the calibration raised a few questions regarding the current stoichiometric model: Mainly (i) why is less glycogen produced than consumed? And (ii) is the energy in PP all represented by glycogen (organic material that is measurable as COD) or is there a possibility of it not being measurable as COD in the anaerobic reactor (i.e., NADH2 formed aerobically from PHB degradation)? The breakdown of an organic component such as glycogen or acetate, to act as the reducing agent in the anaerobic phase, results in CO2 production. With the partial pressure of CO2 maintained according to Henry's law expression (Loewenthal et al., 1994), CO2 gets dissolved into the aqueous phase resulting in a lower pH prediction (see results section below). Is there a possibility of lower CO2 solubility in the AD to stabilise the pH accordingly? This may be important to explore as the pH prediction is significant for AD of P-rich sludge, due to the potential for the occurrence of further processes such as mineral precipitation.
The flow diagram (Fig. 2) provides tracking of PP in the aerobic and anaerobic environments. In aerobic P uptake, ATP is required to make the PP, indicating that PP contains energy, which came from the electron transport chain in aerobic conditions. This 'energy' in PP is equivalent to electrons donated from PHB to O2 in the catabolic PP uptake process - instead of being lost as heat this energy is used in linking orthophosphate to the PP chain (Kortstee et al., 2000). The electrons used here are accounted for by the oxygen used in the aerobic process. The fraction of COD in PHB used to store PP is defined by a parameter YPHA. For a plant-wide model, the COD of PHB used to form PP (that is released in the anaerobic digester) is accounted for by deducting from the flux of oxygen used aerobically (FOc), the YPHA fraction of the PHB used for aerobic storage of PP. In the anaerobic reactor (or digester) this energy (released with PP hydrolysis) is used to reclaim electrons from the oxidation of water during the PP release process (Eq. 1), to HAc to make 1 PHB (C4H6O2 = 8e-).
EXPERIMENTAL AND MODELLING RESULTS
Figure 3 shows the graphs resulting from the progressive validation of the three-phase (aqueous-gas-solid) ASM2-3P model, through a comparison of model simulation results to data generated from the experimental investigation by Mebrahtu et al. (2007), Ikumi (2011) and Vogts et al. (2014), who operated the UCT AS system from which WAS was abstracted and fed to the AD of the experimental set-up (see Fig. 1).
As exhibited in Figs 3a and b, the ASM2-3P models the behaviour of the parent UCT NDEBPR system well by predicting values close to experimental observation for: mixed liquor COD (includes all biomass and other waste organics), ISS (includes PP and reactor-accumulated influent ISS; mineral precipitation was not observed here, hence the model correctly excludes precipitates from the ISS, which, incidentally, also validates the ISS model of Ekama and Wentzel (2004) that is included in the extension of the ASM2-3P model), OP (for organics and P removal shown in Fig. 3a), total Kjeldahl nitrogen (TKN) and FSA (for N removal shown in Fig. 3b). This is important for plant-wide modelling because the PP is the main source of orthophosphate (OP) and metals (Mg, Ca and K) in the subsequent WAS treatment systems (AD or aerobic digestion), and hence determines the extent to which precipitation and other weak acid/base processes take place (Ikumi et al., 2015).
Figure 4 shows a comparison between the results simulated by the three-phase AD dynamic model (SDM3P) and AD data measured with changing AD sludge age (10, 18, 25, 40 and 60d) for the AD of the NDEBPR WAS. Also, two different modelling approaches are compared here (i.e. using simulated predictions of Model 1, where P release occurs with PHB formation by PAOs as shown in Eq. 2c and Model 2, where P release occurs without PHB formation, as shown in Eq. 1).
Apart from COD removal (where all simulated results match measured ones well), the results predicted by the SDM3P simulation model (Fig. 4a to f) do not yet have a good correspondence to those measured (as mentioned earlier, this has resulted in various questions regarding the approach to be taken in modelling the process). In all cases, the FSA release shows a similar trend to COD removal, indicating its steady release as the biodegradable organics of the sludge is hydrolysed (the kinetics of hydrolysis initially having been calibrated by Ikumi et al., 2014). The biodegradable organic (i.e., the active ordinary heterotrophic organism (OHO) and phosphorus accumulating organism (PAO) biomass) sludge hydrolysis, results in the release of organically bound N, which is in the non-ionic NH3 form and is in the form of non-reference species for the ammonia weak acid/base system. On release, the NH3 picks up an H+ ion from the bulk liquid which is supplied (in part) by the dissolved CO2 (H2CO3*) forming HCO3- i.e.:
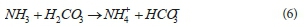
However, for AD of EBPR WAS the total alkalinity generation not only depends on the release of organically bound N but also on how PP is released.
The initial PP release in the AD occurs with the uptake of acetate and results in increased alkalinity by the addition of H2PO4- (see Eq. 2c). The eventual PAO death and hydrolysis in the AD (due to the lack of terminal electron acceptor, oxygen, to allow for their growth) results in the subsequent release of the remaining PP and other stored PAO components such as PHB. This release of stored PHB and PP is also modelled to occur independently at the PAO hydrolysis because there is not enough information to know the manner by which disintegration of the stored PP and PHB occurs after exposure of the biomass cytoplasm.
The PP release upon PAO hydrolysis (see Eq. 3a) results in, depending on the charge/proton balance requirements, some of the H2PO4- species becoming HPO42- species while keeping the H3PO4 alkalinity constant. This release of a proton from H2PO4- results in increased ρCO2 and decreased pH - a proton is added to HCO3- producing H2CO3, which then gets released to the atmosphere as CO2 when the Henrys' equilibrium constant is exceeded.
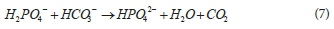
Since with PP release, there is lowered pH and no net alkalinity change, the reaction releases 2H+ with each PO4-3; however the PO4-3 is approximately equally divided between HPO4-2 and H2PO4- at pH 7, so it effectively releases 1H+ per PO4-3 to the solution.
Connected to (and hence at the same rate as) hydrolysis of 'dead' PAO (and OHO) biomass, organic P is released as H3PO4. Because this is the reference species for the OP weak acid/base system, this organic P release does not cause a change in total alkalinity. However, in the AD pH range 7 to 8, the H3PO4 species lose 1 or 2 H+ to become H2PO4- and HPO42- species. As shown in Eq. 7 this H+ reacts with HCO3- to become H2O and CO2. So while the total alkalinity does not change, the species that represent it do, and the CO2 that would have been retained in the aqueous phase as HCO3- now exits the AD as gas, which increases the ρCO2 (the CH4 gas production remains unchanged because it is fixed by the COD of the biodegraded organics).
Therefore, accurate prediction of total alkalinity, ρCO2 and pH requires accurate estimation of the N and P content of the PAO (and OHO) biomass, the PP quantity stored and the correct rates of PP release (both with PHB uptake and after PAO death) and biomass organics hydrolysis. If a limited quantity of acetate is generated from partial hydrolysis (e.g., due to low AD sludge age) and subsequent fermentation of biodegradable organics, the PP release with PHA uptake may be interrupted, allowing more PP hydrolysis with PAO death.
The rapid breakdown of PP yields high concentrations of P, Mg2+, K+ and C2+ species in the AD mixed liquor already at short sludge ages (< 10 d; see Fig. 4c). This, together with the increased release of ammonia with sludge age, results in struvite (MgNH4PO4∙6H2O) precipitation potential. This struvite precipitation removes PO43- species from the aqueous phase, hence decreasing the total alkalinity and resulting in re-speciation of the inorganic carbon and other weak acid/base systems to cause a further decrease in ρCO2 and AD pH (Loewenthal et al., 1994).
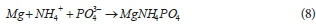
The measurements of total and soluble K in the influent and effluent suggest the complete (or almost complete) release of PP for all residence times; in that the effluent dissolved K is very close to the effluent total K. Although the K from the released PP does not precipitate, the Mg has the potential for precipitate formation - this is not accounted for well in the model simulations of Fig. 4. The influent soluble Mg concentrations are around 80 mg/L, while the effluent concentrations are remarkably constant at around 25 mg/L - this in spite of the Mg released from PP. The constancy suggests equilibrium with a precipitate, but none of the Mg precipitates in the model appears to be able to reduce the Mg to that level, due to low pH prediction.
As can be noticed in Figs 4a-f, both the simulation of PP release without considering PHB formation (i.e., using stoichiometry from Eq. 1) and with PHB formation, where an organic compound is utilised as a reducing agent (see Eq. 2c), results in underprediction of the system pH, and consequent miscalculation of precipitation potential (low pH does not support the formation of struvite and other mineral precipitates). This miscalculation of precipitation potential (due to low pH prediction) results in the poorly predicted AD effluent FSA, OP and metals (Mg, K and Ca) in the aqueous phase. If the PP release is modelled according to Eq. 2a (with the e- and H+ sourced from NADH2 formed aerobically with PHB degradation in the parent NDEBPR AS system), better predictions for pH are obtained due to improved accuracy of calculated precipitation, AD FSA, OP and metals (see Fig. 7a-d). This suggests the uptake of H+ ions is an aspect requiring further investigation. It has been observed that the quantity of PP released to energise PHB formation is dependent on the system pH, rather than vice versa. With PHB formation maintained constant, an increased reactor pH would result in more PP degraded (Smolders, 1995). Moreover, greater certainty of predicted ionic strength would be a valuable addition to the calibration exercise (lacking in this dataset) - due to the increased concentration of metals and phosphates released. Ionic strength and ion pairing affect mineral precipitation calculation much more strongly than pH calculation (Tait et al., 2012) especially for a trivalent ion like PO43-.
Apart from the polyphosphate release stoichiometry, other possible contributions to explore for the under-predicted pH from Fig. 4d include:
i. Formation of precipitates in the feed: All the precipitation reactions in the models are favoured by higher pH values, but lower the pH when they occur in the anaerobic reactor. Conversely, if precipitates are present in the feed, they will tend to dissolve if the pH drops, and act as a pH buffer. They would provide a reservoir of alkalinity which is not detected by a 5-point titration (Moosbrugger et al., 1993) performed on liquid separated from particulates. This has implications for the ISS in the feed. From the experimental data, it is evident that the influent ISS varies considerably, whereas the effluent ISS is much more consistent. In the model, the ISS is the sum of the PP and the model ISS component. To properly calibrate PP release would require experimental data that characterises the ISS towards the location of the particulate phosphorus (i.e., whether the ISS is PP or a mineral precipitate such as struvite). Hence it is recommended in the future project to test for conditions (pH and temperature) that would allow for a diluted sample that achieves re-dissolution of mineral precipitates (change from solid to aqueous phase) without altering polyphosphates (stay in solid phase).
ii. Potential for lower CO2 solubility in the AD (e.g., reduction in the CO2 partial pressure due to the presence of inert gas such as nitrogen; N2) resulting in further CO2 transfer from aqueous to the gaseous phase. The evolution of CO2 would cause a decrease in acidity and an increase in the system pH.
The AD of WAS-containing enhanced cultures of PAO
The findings from this investigation have resulted in a few research questions, regarding the extent to which existing mathematical models virtually represent the AD of WAS from EBPR WWTP systems. To allow for more precise tracking of material components (i.e., C, H, O, N, P, metals, ISS, charge and energy) through the WWTP, in research or practical applications, necessitates having a definitive description of the biochemical mechanisms of PAO enhanced cultures. Towards this end, further investigation is required into (i) the extent to which PP is released with PHB formation (resulting in energy generation, alkalinity increase and ρCO2 decrease) rather than PAO death (results in total alkalinity decrease and ρCO2 increase), and (ii) the endogenous mass fraction of PAOs. The parent activated sludge experimental set-up is similar to that of Wentzel (1988), i.e., a continuous anaerobic-aerobic system fed volatile fatty acids, sufficient orthophosphate (to avoid P limitation) with the required quantity of mineral supplements (including Mg, K and Ca for synthesis of PP) and ammonia (just enough for biomass growth, hence avoiding anoxic conditions that would result from nitrification). Further, the waste sludge from this EBPR system will be fed to anaerobic digestion units and batch tests to generate data towards calibrating the maximum specific rate of anaerobic PHB uptake. These AD batch tests on enhanced cultures of PAO biomass may also be useful to investigate further the process of PAO death in AD for validating the kinetics of PAO hydrolysis and the endogenous residue faction (the AD effluent is essentially PAO endogenous residue). In the AS system, the PAOs' endogenous respiration rate is known to be at about 0.04/d, with the endogenous residue being about 0.25 of the PAO biomass (Wentzel et al., 1990). In the AD system, it is likely that the PAOs die at a faster rate, since the AD biomass is acting on them as a substrate source. However, rather than their death rate, of importance is the rate at which their biodegradable particulate organics hydrolyse. Ikumi et al. (2014) report the biodegradability and kinetics of hydrolysis of WAS from NDEBPR AS system, as similar to that of primary sludge, but this was obtained from the AD of WAS containing mixed cultures of OHO and PAO biomass.
Within this same experimental set-up, the PHB formation rate can also be calibrated by testing the quantity of PHB formed (with PP release) at changing anaerobic reactor retention times. It is expected that if this PHB formation rate can also be used in the AD (since PAOs are again exposed to anaerobic conditions with the presence of acetate) to provide reasonable outputs for pH and ρCO2, this would validate the concept of PP contributing to some 'energy' carry over from the AS to AD system.
CONCLUSIONS
The behaviour of PAOs in anaerobic digestion was investigated but inadequacy in the experimental data prevented making definitive conclusions. However, the results do indicate that PAO behaviour may influence AD pH in ways that OHO do not.
i. In AS systems the stoichiometry of PP-accumulating organisms includes the processes of aerobic P uptake to form PP and anaerobic P release from this PP. The energy for aerobic PP formation is sourced from poly3-hydroxybutyrate (PHB) breakdown, with oxygen used as the terminal electron acceptor. Hence this oxygen used represents electrons transferred from the PHB and allows for the COD balance to be made over the activated sludge (AS) system. However, the 'energy' acquired in this PHB breakdown and PP uptake process is available in the PP when it is reused in the anaerobic reactor of the EBPR system. The energy within this PP allows for the anaerobic formation and storage of PHB using acetate in AS systems.
ii. When the WAS from parent EBPR AS systems is fed to an anaerobic digestion (AD) system, it is expected that a similar process of PHB formation with PP release occurs (due to the combination of anaerobic conditions and presence of acetate), as for the AS system. However, this PHB eventually gets broken down to methane and carbon dioxide, since there is no alternating aerobic reactor for its utilisation in PAO growth.
iii. When modelling the AD system in this way (as defined in (ii), we notice that better predictions are obtained (with acetate uptake and PHB formation included) than when modelling the AD PP release to occur with PAO death and hydrolysis. However, to validate this model, and explore the energy transfer between aerobic reactor of AS system and AD systems, requires further investigation on the AD of enhanced cultures of PAOs to amplify the PAO behaviour in the AD.
ACKNOWLEDGEMENTS
This research was supported by the Water Research Commission, the National Research Foundation and the University of Cape Town, and is published with their permission.
REFERENCES
BATSTONE DJ, KELLER J, ANGELIDAKI I, KALYUZHNYI SV, PAVLOSTATHIS SG, ROZZI A, SANDERS WTM, SIEGRIST H and VAVILIN VA (2002) Anaerobic digestion model No 1 (ADM1), Scientific and Technical Report No 9. International Water Association (IWA), London, UK. https://doi.org/10.2166/wst.2002.0292 [ Links ]
BATSTONE DJ, AMERLINCK Y, EKAMA G, GOEL R, GRAU P, JOHNSON B, KAYA I, STEYER J-P, TAIT S, TAKÁCS I, VANROLLEGHEM PA, BROUCKAERT CJ and VOLCKE E (2012) Towards a generalized physicochemical framework. Water Sci. Technol. 66 (6) 1147-1161. https://doi.org/10.2166/wst.2012.300 [ Links ]
BROUCKAERT CJ, IKUMI DS and EKAMA GA (2010) A 3-phase anaerobic digestion model. Proc. 12th IWA Anaerobic Digestion Conference (AD12), 1-4 November 2010, Guadalajara, Mexico. [ Links ]
BRUN R, KÜHNI M, SIEGRIST H, GUJER W and REICHERT P (2002) Practical identifiability of ASM2d parameters-systematic selection and tuning of parameter subsets. Water Res. 36 4113-4127. https://doi.org/10.1016/s0043-1354(02)00104-5 [ Links ]
COLE JA and HUGHES DE (1965) The metabolism of polyphosphates in chlorobiumthiosulfatophilum. J. Gen. Microbiol. 38 (1) 65-72. [ Links ]
COMEAU Y, HALL KJ, HANCOCK REW and OLDHAM WK (1985) Biochemical model for enhanced biological phosphorus removal. Proc. UBC Conference on New Directions and Research in Water Treatment and Residuals Management, June 1985, Vancouver, Canada. https://doi.org/10.1016/0043-1354(86)90115-6 [ Links ]
DAIGGER TD, HENRY CL and LESLIE CP (1999) Biological Wastewater Treatment. (2nd edn.) Marcel Dekker Inc., New York, USA. [ Links ]
EKAMA GA and WENTZEL MC (2004) A predictive model for the reactor inorganic suspended solids concentration in activated sludge systems. Water Res. 38 (19) 4093-4106. https://doi.org/10.1016/j.watres.2004.08.005 [ Links ]
EKAMA GA, WENTZEL MC and SÖTEMANN SW (2006) Mass balanced-based plant-wide wastewater treatment plant models - Part 2: Tracking the influent inorganic suspended solids. Water SA 32 (3) 277-285. https://doi.org/10.4314/wsa.v32i3.5272 [ Links ]
EKAMA GA (2009) Using bio-process stoichiometry to build a steady state plant-wide wastewater treatment plant model. Water Res. 43 (8) 2101-2120. https://doi.org/10.1016/j.watres.2009.01.036 [ Links ]
FLORES-ALSINA X, SOLON K, KAZADI MBAMBA C, TAIT S, JEPPSSON U, GERNAEY KV and BATSTONE DJ (2016). Modelling phosphorus, sulphur and iron interactions during the dynamic simulation of anaerobic digestion processes. Water Res. 95 370-382. https://doi.org/10.1016/j.watres.2016.03.012 [ Links ]
HARDING TH, IKUMI DS and EKAMA GA (2010) A steady state stoichiometric model describing the anaerobic digestion of biological excess phosphorus removal waste activated sludge. Research Report W132 (MSc thesis), Department of Civil Engineering, University of Cape Town, South Africa. [ Links ]
HAUDUC H, RIEGER L, TAKÁCS I, HÉDUIT A, VANROLLEGHEM PA and GILLOT S (2010) A systematic approach for model verification: Application on seven published activated sludge models. Water Sci. Technol. 61 (4) 825-839. https://doi.org/10.2166/wst.2010.898 [ Links ]
HENZE M, GUJER W, MINO T, MATSUO T, WENTZEL MC and MARAIS GVR (1995) Activated sludge model No.2 (ASM2). IWA Scientific and Technical Report No. 3. IWA Publishing, London. https://doi.org/10.2166/wst.1995.0061 [ Links ]
HENZE M, VAN LOOSDRECHT MCM, EKAMA GA and BRDJANOVIC D (2008) Biological Wastewater Treatment: Principles, Design and Modelling. IWA Publishing, London. 620 pp. https://doi.org/10.2166/9781780408613 [ Links ]
IKUMI DS (2011) The Development of a Three-phase Plant-Wide Mathematical Model for Sewage Treatment. PhD thesis, Water Research Group (WRG). Department of Civil Engineering. University of Cape Town, South Africa. [ Links ]
IKUMI D, VANROLLEGHEM PA, BOUCKAERT V, NEUMANN M and EKAMA G (2014) Towards calibration of phosphorus (P) removal plant-wide models. Proc. 4th IWA/WEF Wastewater Treatment Modelling Seminar (WWTmod2014), 30 March-2 April 2014, Spa, Belgium. 197-206. [ Links ]
IKUMI DS, HARDING TH, VOGTS M, LAKAY MT, MAFUNGWA HZ, BROUCKAERT CJ and EKAMA GA (2015) Mass balances modelling over wastewater treatment plants III. WRC Report No. 1822/1/14. Water Research Commission, Pretoria. https://doi.org/10.1016/j.watres.2014.02.008 [ Links ]
IKUMI DS, HARDING TH and EKAMA GA (2014) Plant-wide wastewater treatment modelling (1) - Biodegradability of wastewater and activated sludge organics in anaerobic digestion. Water Res. 56 (1) 267-279. [ Links ]
JARDIN N and PÖPEL HJ (1994) Phosphate release of sludge from enhanced biological P-removal during digestion. Water Sci. Technol. 30 (6) 281-292. https://doi.org/10.2166/wst.1994.0279 [ Links ]
JEPPSSON U, PONS M-N, NOPENS I, ALEX J, COPP JB, GERNAEY KV, ROSEN C, STEYER J-P and VANROLLEGHEM PA (2007) Benchmark simulation model No2: General protocol and exploratory case studies. Water Sci. Technol. 56 (8) 67-78. https://doi.org/10.2166/wst.2007.604 [ Links ]
KAZADI MBAMABA C, FLORES-ALSINA X, BATSTONE DJ and TAIT S (2015) A systematic study of multiple minerals precipitation modelling in wastewater treatment. Water Res. 85 359-370. https://doi.org/10.1016/j.watres.2015.08.041 [ Links ]
LIZARRALDE I, FERNÁNDEZ-ARÉVALOA T, BROUCKAERT CJ, VANROLLEGHEM PA, IKUMI DS, EKAMA GA, AYESA E and GRAU P (2015) A new general methodology for incorporating physicochemical transformations into multi-phase wastewater treatment process models. Water Res. 74 (5) 239 -256. https://doi.org/10.1016/j.watres.2015.01.031 [ Links ]
LOEWENTHAL RE, KORNMULLER URC and VAN HEERDEN EP (1994) Modelling struvite precipitation in anaerobic treatment systems. Water Sci. Technol. 30 (12) 107-116. https://doi.org/10.2166/wst.1994.0592 [ Links ]
MEBRAHTU MK, WENTZEL MC and EKAMA GA (2007) Aerobic digestion of waste activated sludge from biological N and P removal systems. Research Report No. W126, Department of Civil Engineering, University of Cape Town, South Africa. [ Links ]
MOOSBRUGGER RE, WENTZEL MC, EKAMA GA and MARAIS GVR (1993b) A five - pH point titration method for determining the carbonate and SCFA weak acid/bases in anaerobic systems. Water Sci. Technol. 28 (2) 237-246. https://doi.org/10.2166/wst.1993.0112 [ Links ]
McCARTY PL (1975) Stoichiometry of biological reactions. Prog. Water Technol. 7 (1) 157-172. [ Links ]
MINO T, SATOH H and MATSUO T (1994) Metabolism of different bacterial populations in enhanced biological phosphate removal processes. Water Sci. Technol. 29 (7) 67-70. https://doi.org/10.2166/wst.1994.0309 [ Links ]
MUSVOTO EV, WENTZEL MC and EKAMA GA (2000) Integrated chemical- physical processes modelling I. Development of a kinetic based model for weak acid/base systems. Water Res. 34 (6) 1857-1867. https://doi.org/10.1016/s0043-1354(99)00334-6 [ Links ]
NEUMANN MB (2012) Comparison of sensitivity analysis methods for pollutant degradation modelling: A case study from drinking water treatment. Sci. Total Environ. 433 530-537. https://doi.org/10.1016/j.scitotenv.2012.06.026 [ Links ]
PEREIRA H, LEMOS PC, REIS MAM, CRESPO JPSG, CARRONDO MJT and SANTOS H (1996) Model for carbon metabolism in biological phosphorus removal processes based on in vivo C-NMR labeling experiments. Water Res. 30 (9) 2128-2138. https://doi.org/10.1016/0043-1354(96)00035-8 [ Links ]
SMOLDERS GJF (1995) A metabolic model of the biological phosphorus removal (stoichiometry, kinetics and dynamic behavior). PhD thesis, Department of Biochemical Engineering, Tech. University Delft, The Netherlands. [ Links ]
SMOLDERS GJF, VAN DER MEIJ J, VAN LOOSDRECHT MCM and HEIJNEN JJ (1995) A structured metabolic model for the anaerobic and aerobic stoichiometry and kinetics of the biological phosphorus removal process. Biotechnol. Bioeng. 47 277-287. https://doi.org/10.1002/bit.260470302 [ Links ]
SOLON K, FLORES-ALSINA X, KAZADI MBAMBA C, IKUMI D, VOLCKE EIP, VANEECKHAUTE C, EKAMA GA, VANROLLEGHEM PA, BATSTONE DJ, GERNAEY KV and JEPPSSON U (2017) Plant-wide modelling of phosphorus transformations in wastewater treatment systems: Impacts of control and operational strategies. Water Res. 113 97-110. https://doi.org/10.1016/j.watres.2017.02.007 [ Links ]
SÖTEMANN SW, RISTOW NE, WENTZEL MC and EKAMA GA (2005) A steady-state model for anaerobic digestion of sewage sludges. Water SA 31 (4) 511-527. https://doi.org/10.4314/wsa.v31i4.5143 [ Links ]
SÖTEMANN SW, WENTZEL MC and EKAMA GA (2006). Mass balance based plant-wide wastewater treatment plant models - Part 4: Aerobic digestion of primary and waste activated sludges. Water SA 32 (3) 297-306. https://doi.org/10.4314/wsa.v32i3.5274 [ Links ]
TAIT S, SOLON K, VOLCKE EIP and BATSTONE DJ (2012) A unified approach to modelling wastewater chemistry: model corrections. Proc. 3rd Wastewater Treatment Modelling Seminar (WWTmod2012), 26-28 February 2012, Mont-Sainte-Anne, Quebec, Canada. [ Links ]
VANHOOREN H, MEIRLAEN J, AMERLINCK Y, CLAEYS F, VANGHELUWE H and VANROLLEGHEM PA (2003) WEST: modelling biological wastewater treatment. J. Hydroinf. 5 27-50. https://doi.org/10.2166/hydro.2003.0003 [ Links ]
VAN RENSBURG P, MUSVOTO EV, WENTZEL MC and EKAMA GA (2003) Modelling multiple mineral precipitation in anaerobic digester liquor. Water Res. 37 (13) 3087-3097. https://doi.org/10.1016/s0043-1354(03)00173-8 [ Links ]
VANROLLEGHEM PA, INSEL G, PETERSEN B, SIN G, DE PAUW D, NOPENS I, WEIJERS S and GERNAEY K (2003) A comprehensive model calibration procedure for activated sludge models. In: Proceedings WEF 26th Annual Technical Exhibition and Conference (WEFTEC, 2003), Los Angeles, CA, USA, 11-15 October 2003. https://doi.org/10.2175/193864703784639615 [ Links ]
VAN VEEN HW, ABEE T, KORTSTEE GJJ, PEREIRA H, KONINGS WN and ZEHNDER AJB (1994) Generation of a proton motive force by the excretion of metal phosphate in the polyphosphate-accumulating Acinetobacter johnsonii 210A. J. Biol. Chem. 269 29509-29514. https://doi.org/10.1128/jb.175.1.200-206.1993 [ Links ]
VOGTS M, IKUMI DS and EKAMA GA (2014) The removal of N and P in aerobic and anoxic-aerobic digestion of waste activated sludge from biological nutrient removal systems. Proc. WISA Conference 2014, 25-29 May 2014, Mbombela. https://doi.org/10.4314/wsa.v41i2.05 [ Links ]
WANG R, YONGMEI L, CHEN W, ZOU J and CHEN Y (2016) Phosphate release involving PAOs activity during anaerobic fermentation of EBPR sludge and the extension of ADM1. Chem. Eng. J. 297 (1) 436-447. https://doi.org/10.1016/j.cej.2015.10.110 [ Links ]
WENTZEL MC (1988) Biological excess phosphorus removal in activated sludge systems. PhD thesis, Water Research Group, Department of Civil Engineering, University of Cape Town, South Africa. [ Links ]
WENTZEL MC, EKAMA GA, DOLD PL and MARAIS GVR (1990) Biological excess phosphorous removal - steady state process design. Water SA 16 (1) 29-48. [ Links ]
Received 11 April 2018
Accepted in revised form 26 June 2019
* Corresponding author, email: david.ikumi@uct.ac.za














