Servicios Personalizados
Articulo
Indicadores
Links relacionados
-
 Citado por Google
Citado por Google -
 Similares en Google
Similares en Google
Compartir
Journal of Energy in Southern Africa
versión On-line ISSN 2413-3051
versión impresa ISSN 1021-447X
J. energy South. Afr. vol.24 no.3 Cape Town ene. 2013
RESEARCH ARTICLE
Exergy analysis of a flat plate solar collector
Sunil Chamoli
GRDIMT Dehradun, Uttarakhand, India
ABSTRACT
In this study, exergetic performance analysis of flat plate solar collector has been carried out analytically. A comprehensive mathematical modelling of thermal performance is simulated using MATLAB simulink and optimal geometrical and thermody-namic parameters are predicted pertaining to optimum performance of the system. The optimization procedure was applied to a typical collector and the optimum design points were extracted. The optimum values of collector inlet temperature, mass flow rate, absorber plate area, and fluid outlet temperature for maximum exergy inflow from the system have been obtained.
Keywords: exergy, optimization, solar collector, solar energy
1. Introduction
The role of energy becomes increasingly important to fulfil needs of modern societies and to sustain fast economic and industrial growth worldwide. In view of the world's depleting fossil fuel reserves and environmental threats, development of renewable energy sources receives importance. Of many alternatives, solar energy stands out as a conspicuous energy source for meeting the demand. Though it is located and time dependent, it requires efficient collection and storage systems for economic utilization. The easiest way to utilize solar energy for heating applications is to convert it into thermal energy by using solar collectors.
The performance of solar collectors can be examined from the standpoint of exergy, which is a useful method to complement, not to replace the energy analysis. The amount of useful energy (exergy) delivered by solar collectors is found to be affected by heat transfer irreversibility between the sun and the collector, between the collector and the ambient air and inside the collector. Exergy analysis quantifies the collection and useful consumption of exergy and pinpoints the unrecoverable losses, leading the way to improve the system. The rate at which the exergy is collected by a solar collector can be increased by increasing the mass flow rate of the fluid through the collector and/or the collector area. Since the collector is an expensive component of any solar thermal system which needs advanced material technology to build it and hence, requires large investment in the form of capital cost. We have to optimize the collector area in order to reduce the capital cost of the system, as the fuel (sunlight) is free. Again, for large mass flow rates the fluid outlet temperature is very low and requires more power to pump the fluid through the collector. At the other limit low flow rates result in high fluid outlet temperatures with high specific work potential but the energy losses increase due to the temperature differences, therefore, the optimum mass flow rate is required.
Farahat et al. (2005) have done exergetic optimization of a flat plate solar collector, which is developed to determine the optimal performance and design parameters of the solar to a thermal energy conversion system. Exergetic optimization has been carried out under design considerations and optimum values of mass flow rate, absorber plate area and the maximum exergy efficiency have been found.
Ucar (2006) has done an experimental investigation carried out for the shape and arrangement of absorber surfaces of the collectors that were reorganized to provide better heat transfer surfaces suitable for the passive heat transfer augmentation techniques. The performance of such solar air collectors with staggered absorber sheets and attached fins on absorber surface were tested. The exergy relations are delivered for different solar air collectors. It is seen that the largest irreversibility is occurring at the conventional solar collector in which collector efficiency is smallest.
Hikmet (2008) does an experimental energy and exergy analysis for a novel flat plate solar air heater (SAH) with several obstacles and without obstacles. For increasing the available heat-transfer area, this may be achieved if air is flowing simulta- neously and separately over and under the different obstacle absorbing plates, instead of only flowing either over or under the different obstacle absorbing plates, leading to improved collector efficiency. The measured parameters were the inlet and outlet temperatures, the absorbing plate temperatures, the ambient temperature, and the solar radiation. Further, the measurements were performed at different values of mass flow rate of air and different levels of absorbing plates in the flow channel duct. After the analysis of the results, the optimal value of efficiency is the middle level of the absorbing plate in the flow channel duct for all operating conditions and the double-flow collector supplied with obstacles appears significantly better than that without obstacles. At the end of this study, the exergy relations are delivered for different SAHs. The results show that the largest irreversibility is occurring at the flat plate (without obstacles) collector in which collector efficiency is smallest.
Kahrobaian (2008) proposed a new method of optimization on linear parabolic solar collectors using exergy analysis that is presented. A comprehensive mathematical modelling of thermal and optical performance is simulated and geometrical and thermodynamic parameters were assumed as optimization variables. By applying a derived expression for exergy efficiency, exergy losses were generated and the optimum design and operating conditions were investigated. The objective function (exergy efficiency) along with constraint equations constitutes a four-degree freedom optimization problem. Using the Lagrange multipliers method, the optimization procedure was applied to a typical collector and the optimum design point was extracted.
To study the effect of changes in optimization variables on the collected exergy, the sensitivity of optimization to changes in the collector parameters and operating conditions is evaluated and variation of exergy fractions at this point are studied and discussed in the present study.
2. Energy analysis
The energy analysis of a flat plate solar collector is given by Sukhatme (1993). The useful heat gain from working fluid is:

Where  , Cp, T0, Ti, is the mass flow rate, specific heat, Outlet and Inlet temperature of working fluid. The Hottel-Whiller equation for the useful heat gain from solar collector is:
, Cp, T0, Ti, is the mass flow rate, specific heat, Outlet and Inlet temperature of working fluid. The Hottel-Whiller equation for the useful heat gain from solar collector is:
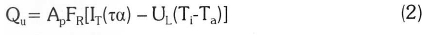
Where Ap, FR, Ta, UI, IT, (τα) are the area of the absorber plate, Heat removal factor, ambient tem- perature, overall heat loss coefficient, incident solar radiation and the transmittance absorptance product.
Heat removal factor is defined as:
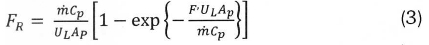
Where F' is the collector efficiency factor.
Thermal efficiency of solar collector is defined as

3. Exergy analysis
Application of exergy analysis to a solar collector helps designers to achieve an optimum design and gives direction to decrease exergy losses. The Exergy concept is one of the two ways to the second law analysis, and entropy generation from irre-versibility's is the other method. However, both techniques fundamentally give identical results. Decreasing exergy destructed during thermody-namic processes can be achieved by the view point of maximizing exergy efficiency. By applying exergy balance on a solar collector (shown in Figure 1a), exergy efficiency can be derived and the shares of irreversible factors are defined as well. Exergy balance on a flat plate solar collector, as depicted in Figure 1a, can be generally expressed as Suzuki (1987):
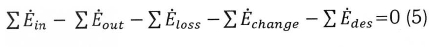
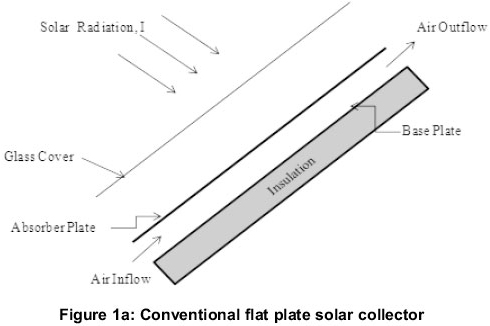
The associated exergy losses of a system shown in Figure 1a are given in Figure 1b.
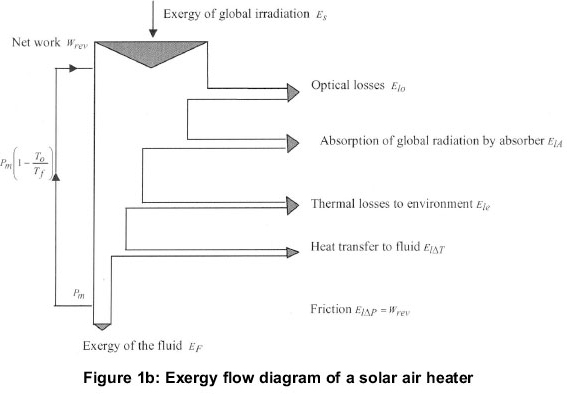
The exergy input rate σ Ėin includes the exergy accompanying mass flow rate and the exergy of solar radiation and exergy out rate includes exergy mass flow. The Ėgain is the exergy accumulated by the fluid flow:

Exergy efficiency is the ratio of gain exergy to solar radiation exergy.

A dimensional less parameter is defined as 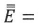
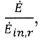 is the ratio of exergy to the incoming radiation sxergy from the sun.
is the ratio of exergy to the incoming radiation sxergy from the sun.
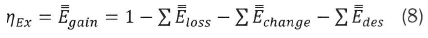
The inlet exergy rate includes the inlet exergy rate with fluid flow and the absorbed solar radiation.
The inlet exergy rate includes the inlet exergy rate with fluid flow and the absorbed solar radiation exergy rate. The inlet exergy rate with fluid flow is given by:
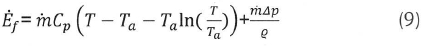
According to Petela's theory, the exact exergy income by solar radiation for a typical collector with surface area of Ap becomes:

ηρ is Petela's efficiency of converting radiation energy (i.e.ITAp ) into work as Bejan (1988):

Where Ts is the sun temperature.
Considering the exergy at inlet and outlet then exergy efficiency is expressed as
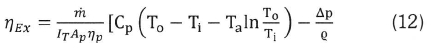
the exergy efficiency should be expressed in terms of lost and destructed exergy to illustrate which exergy fractions are major, and consequently take direction to decrease them. Exergy leakage rate out to the surroundings due to optical errors and heat transfer to ambient in a solar collector and is undesirable:

Due to optical error and surface properties of the absorber tube in collector parts of solar radiation do not reach absorber tube. The fraction of optical exergy rate is:
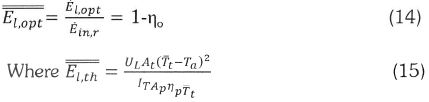
Studying the collector in steady state conditions is thus 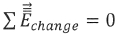 however, exergy change rate of the tube and fluid can be approximately neglected in every condition due to low values.
however, exergy change rate of the tube and fluid can be approximately neglected in every condition due to low values.
Exergy destruction is caused by irreversibility's in the system and there are two ways of exergy destruction in solar collectors; exergy destructed due to friction of viscous fluid and exergy destructed due to heat transfer processes. Since the viscous fluid causes pressure drop between inlet and outlet of the tube, considering correspondent entropy generation, exergy destructed during this process can be stated as

Exergy is also destructed while heat is transferred from hot to cold temperatures. There are two heat transfer processes in the collector; 1) heat transfer of solar energy absorbed by the surface of the tube, 2) heat transfer conduction from outer tube surface to fluid flow. The exergy destructed rate due to absorption and conduction process is defined as:
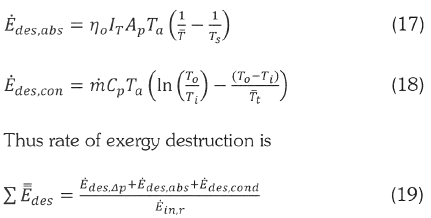
Substituting (16-19) in equation (8), the exergy efficiency can be depicted as:
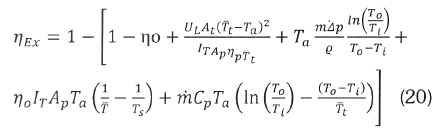
The right term of equation (20) indicates the exergy losses.
4. Results and discussion
Thermal analysis of a solar collector has been carried out using a mathematical simulation technique. The effect of operating parameters on exergy efficiency has been investigated. An optimization of exergy efficiency has been carried out to evaluate the optimum values of operating parameters by using MATLAB computational program.
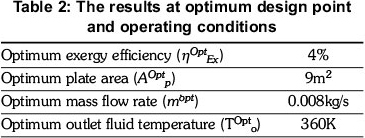
Figure 2 shows the behaviour of the exergy efficiency as a function of the mass flow rate of fluid and the absorber plate area. It is presented to a range of operational and design conditions where the exergy efficiency takes a global maximum value. The incremented quantities are the absorber plate area from 1 to 9 m2 and the mass flow rate of fluid from 0.001 to 0.009 kg/s. The calculated values for the global maximum point are A p= 9 m2, m= 0:008 kg/s, ηEx=4%. Increasing the mass flow rate above the value of m=0.008kg/s shows the no change of exergy efficiency for A p=9 m2. This subject allows the designer to optimize the solar collector regarding other conditions such as design limitations and thermal applications. Whereas, decreasing the mass flow rate below the value of m=0.008kg/s determines the sensible decrease of exergy efficiency for A p=9m2 and this subject shows the danger range.
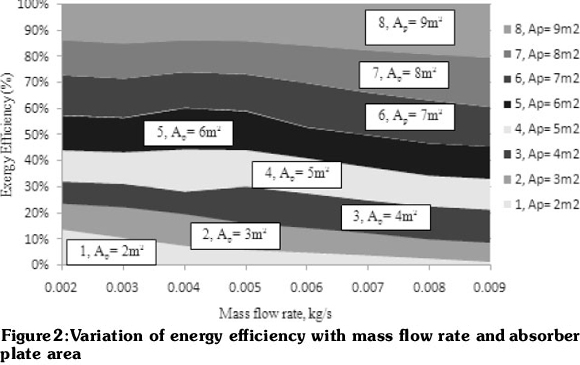
Figure 3 shows the effect of fluid inlet temperature on the exergy efficiency. By increasing the fluid inlet temperature until the value of Ti=300 K, the exergy efficiency increases and then decreases sharply.
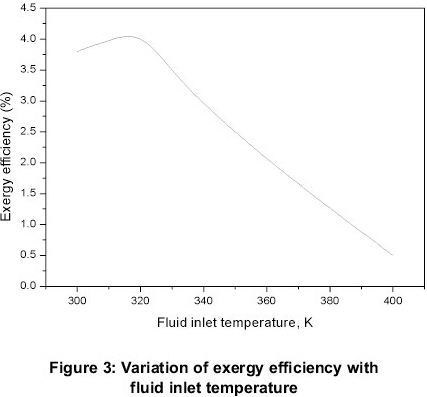
Figure 4 shows the ambient temperature effect on the exergy efficiency. Increasing the ambient temperature from 300 to 313 K determines the sensible decrease of the exergy efficiency. Since the ambient temperature changes during the day, for having the maximum exergy efficiency, other parameters and the solar collector operating conditions should change during the day.
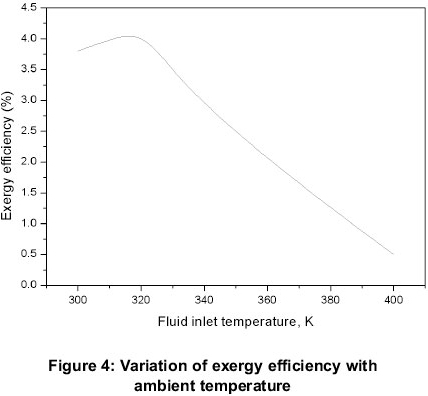
Figure 5 shows the variations of the exergy efficiency versus the wind speed. Increasing the wind speed from 0.001 to 50 m/s determines the sensible decrease of the exergy efficiency from 5 to 4%.
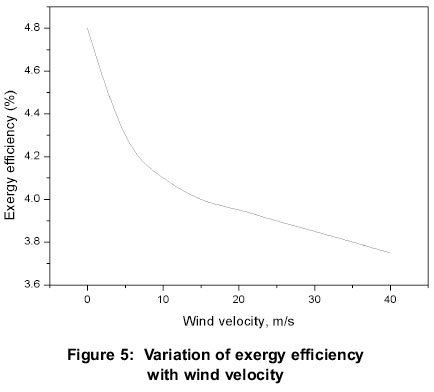
Figure 6 shows the variations of the exergy efficiency with respect to the incident solar energy per unit area of the absorber plate. By increasing this parameter from 100 to 1000 W/m2, the exergy efficiency increases.
5. Conclusion
In this paper, a new and comprehensive method for defining design and operating conditions for a flat plate solar collector was presented. The proposed method consists of an objective design approach, and simulation of thermal performance of the collector. Exergy efficiency was introduced as an objective function and regarding thermal aspects, mathematical modelling for real performance of the collector implemented.
In the optimization procedure, optimum values of collector inlet temperature, mass flow rate, fluid outlet temperature as main variables, were extracted in the presence of environment conditions, material selected and design constraints. The optimum design plots are prepared for operating parameters so that the maximum value of exergy efficiency of the collector was gained.
Nomenclature
A area (m2)
Cp heat capacity of the fluid (kJ/kgK)
D diameter (m)
E exergy rate (J/s)
F' collector effciency factor
FR heat removal factor
IT solar radiation intensity (W/m2) k conductivity (W/mK) m mass flow rate (kg/s)
Q heat transfer rate (W)
S radiation absorbed flux (W/m2)
T temperature (K)
U collector loss coefficient (W/m2K)
V speed, velocity (m/s)
Greek symbols
a absorptance
Δ difference in pressure or temperature
η efficiency (%)
τ transmittance
ϱ density (kg/m3)
(τα) effective product transmittance-absorptance
Subscripts
a ambient
en energy
ex exergy
exp exponential
f fluid
i inlet
l overall
o outlet
p absorber plate, Petela
u useful
References
Bejan, A., (1988). Advanced Engineering Thermodynamics, John Wiley & Sons, 488-491. [ Links ]
Farahat, S., Sarhaddi S., & Ajam H., (2009). Exergetic Optimization of Flat Plate Solar Collector, Renewable Energy, 34, 1169-1174. [ Links ]
Ucar, A., & Inalli L., (2006), Thermal and exergy analysis of solar air collectors with passive augmentation techniques, International Communication in Heat and Mass Transfer, 33, 1281-1290. [ Links ]
Hikmet, E., (2008). Experimental energy and exergy analysis of a double-flow solar air Heater having different obstacles on absorber plates, Building and Environment, 43, 1046-1054. [ Links ]
Kahrobaian, A., & Malekmohammadi H., (2008). Exergy Optimization Applied to Linear Parabolic Solar Collectors, Journal of Faculty of Engineering, 2008, 42, 131-144. [ Links ]
Sukhatme, S.P, (1993). Solar Energy, New York TMH, 1993, 83-139. [ Links ]
Suzuki, A., (1987). General Theory of Exergy Balance and Application to Solar Collectors, 123-128. [ Links ]
Received 22 February 2011
Revised 20 June 2013














