Services on Demand
Article
Indicators
Related links
-
 Cited by Google
Cited by Google -
 Similars in Google
Similars in Google
Share
South African Journal of Education
On-line version ISSN 2076-3433
Print version ISSN 0256-0100
S. Afr. j. educ. vol.28 n.2 Pretoria May. 2008
CONTENTS
Mathematics for teaching: observations from two case studies
Mercy KazimaI; Vasen PillayII; Jill AdlerIII
ISenior Lecturer in mathematics education at the University of Malawi. Her research interests include mathematics for teaching, teaching and learning mathematics in multilingual classrooms, and mathematical modelling. E-mail: mkazima@chanco.unima.mw
IIHead of the District Management Information Systems Unit for the Gauteng Department of Education in the Ekurhuleni North District and has taught mathematics for 11 years. His research interest is mathematics for teaching. E-mail: Vasen.Pillay@gauteng.gov.za
IIIDirector of the Marang Centre for Mathematics and Science Education and Chair of Mathematics Education at the University of the Witwatersrand and at King's College, London. Her international research interests include mathematics teacher education and mathematics for teaching. E-mail: adlerj@educ.wits.ac.za
ABSTRACT
We report on two case studies in which we investigated mathematics for teaching. We were interested in the mathematical knowledge teachers need to know, and know how to use, in order to teach mathematics well. The two case studies focused on the teaching of probability in Grade 8 and the teaching of functions in Grade 10. We discuss the mathematics for teaching probability and functions in terms of the mathematical 'problem solving' or 'mathematical work' demanded of the teachers as they taught the two topics. Among the findings are the interesting differences between the demands on the teaching of functions and the demands on the teaching of probability in these two cases. We argue that mathematics for teaching needs to be understood as shaped by the particular topic being taught, as well as by how teachers select to introduce and approach the ideas and concepts they are teaching. We conclude with a discussion of questions raised for mathematics teacher education, together with implications for practice.
Keywords: mathematical knowledge; mathematics for teaching; mathematical work; problem solving; teacher education
Introduction
The teaching of mathematics does not only require the teacher to be knowledgeable about the topic that is to be taught in the sense that the teacher is proficient in solving any problem within the topic. The teacher needs to know and be able to do more than doing the mathematics for him or herself. The teacher needs mathematical knowledge that is useful and usable for teaching. For example, a teacher must be able to select and clarify appropriate mathematical goals for any lesson taught, and link these with the approach used to teach an idea or concept; a teacher must be able to sequence mathematical tasks into appropriate lesson designs; the teacher must be able to 'evaluate' on the spot the mathematical worth of a learner's explanation or argument; and a teacher needs to be capable of interpreting curriculum materials and also be in a position to explain these to learners and parents if necessary; a teacher must be able to listen to what learners are saying and also be able to pose questions that enable a learner to advance their mathematical thinking. This is by no means an exhaustive list but suffices to show a specificity to the mathematical work of teaching, work that is different from, for example, the mathematical work of a mathematician.
Ball, Bass and Hill (2004) refer to this work as mathematical problem-solving done in and for teaching. They argue that it is productive to think about the kind of mathematical work that teachers do as a special kind of mathematical problem-solving enacted in the practice of teaching. More recently, Hill, Rowan and Ball (2005) have referred to this as the mathematical work of teaching. In this article we report on two similar studies that explored the mathematical work of the teaching two teachers engaged with as they went about their work of teaching mathematics in secondary classrooms in two different Gauteng schools in South Africa. As explained in Adler and Pillay (2007) the notion 'mathematical work' is used to describe the mathematical entailments of the work the teacher does to provide learners with opportunities for mathematical reflection with a focus on the mathematics the teacher draws on to accomplish these tasks. The two studies in question formed part of the QUANTUM1 research project, specifically its interest in mathematics for teaching (MfT).
As will be seen, the studies reflect two different approaches to teaching mathematics — one that is well recognised, especially across the further education and training band (FET)2 in present day South Africa, whilst the other reflects an attempt to embrace new curriculum goals in South Africa, particularly activity-based learning.3 These contrasting approaches were not part of the design of the study. Rather they emerged through the study. Together, however, they provided an important window into the mathematical work of teaching. By observing these two teachers in practice we saw that how they chose to introduce concepts, ideas or procedures to their learners, which was in turn a function of their teaching approach, led them to have to deal with different mathematical work. At face value it is obvious that different approaches will demand different mathematical work. We will argue that the specificity of these differences and how they manifest in classroom practice raises significant questions about descriptions of mathematics for teaching on the one hand, with implications for mathematics teacher education on the other.
Mathematics for Teaching
We are using the term 'mathematics for teaching' (MfT) to refer to 'specialised mathematical knowledge that teachers (need to) know and know how to use in their teaching'. Various studies have delved into the dichotomy of 'content versus pedagogy' in an attempt to define the professional knowledge base of teaching. Some of the foundational work that informs and enlightens this includes, inter alia: Shulman's (1986; 1987) notion of Pedagogic Content Knowledge; Ma's (1999) notion of Profound Understanding of Fundamental Mathematics and the notion of Specialised Knowledge for Teaching Mathematics (Ball & Bass, 2000).
Various researchers (Ball and Bass, 2000; Ball, Lubienski & Mewborn, 2001; Ball et al., 2004; Even, 1990; McNamara, 1991) allude to the notion that knowing mathematics for teaching requires knowing in detail the topics and ideas that are fundamental to the school curriculum and beyond. Many seem to support the ideas of Ball and her co-researchers that in mathematics, knowing in detail entails being able to unpack or decompress mathematical ideas so that they can be accessible to learners. In other words, teachers need not only know how to do mathematics but should also know how to use mathematics in the practice of teaching (Adler, 2004). The unpacking that Ball et al. (2004) are referring to is unpacking from mathematics in relation to learner thinking. Unpacking requires understanding the mathematics of a concept, how this might develop in learning, and then the relationship between these i.e. between what others have referred to as the epistemic and the cognitive. Tall, for example, argues that, in teaching, it is important to start from cognitive roots, rather than mathematical foundations. He defines a cognitive root as 'an anchoring concept which the learner finds easy to comprehend, yet forms a basis on which a theory may be built' (Tall, 1989:40). He offers the function machine, for example, as a cognitive root for the development of the function concept; and argues that this connects more effectively with, and lays foundations for, learners' conceptual thinking, than, for example, beginning with a version of the formal definition of a function.
Others argue similarly that the way mathematical concepts or ideas are introduced to learners is critical. Researchers working with the French theory of didactic situations, for example, emphasise the importance of what they call the 'first encounter' — the first moment of the didactic process or process of study. They argue that in any pedagogy practice, the first encounter with a mathematical idea or concept needs to be purposefully designed (see Barbé, Bosch, Espinoza, & Gascón, 2005).
Being able to 'unpack' mathematical ideas, design first mathematical encounters, discern appropriate cognitive roots all elaborate Shulman's notion of pedagogic content knowledge (PCK). Shulman described PCK as a 'blending' of subject matter knowledge and pedagogic knowledge' and he distinguishes this knowledge from what he calls 'subject matter knowledge'. Elsewhere, we have argued that the boundary between these categories is not clear in the practice of teaching, and hence refer to the more inclusive notion of mathematics for teaching. We have argued further that knowledge-in-use is always towards some purpose and so never outside of social activity (Adler & Huillet, forthcoming). In relation then to mathematics used in teaching, be it unpacking or introducing concepts, it is inevitably institutionalised,4 constrained by the context of schooling, i.e. particular curricular, particular social practices and so forth (Pillay, 2006a).
The question for us is what is it that teachers need to know and be able to do as they unpack mathematics, design first encounters, or navigate between the cognitive and the epistemic and their inter-relation? Does it matter, and research suggests it does, how mathematical ideas are 'rooted'? Does this differ across mathematical topics? And if these are important, then where and how are teachers to learn these mathematical dimensions of their work? Our studies with teachers on their classroom practices began to illuminate these questions. We focused on two specific content areas in mathematics: Functions and Probability. Following Shulman, there have been a number of topic-focused studies in mathematics (e.g. Marks, 1992 — fractions; Sanchez & Llinares, 2003 — functions; Even, 1990 — functions; Stacey, Helme, Steinle, Baturo, Irwin & Bana, 2001 — decimals). Our case studies therefore also add to this growing literature and research.
The case studies
Teaching linear functions in Grade 10 and teaching probability in Grade 8 formed the backdrop against which the two studies were conducted.5 One of the critical questions asked in both studies was what mathematical work of teaching does a teacher engage in as he/she goes about his/her work (i.e. how do they use mathematics in order to teach linear functions or probability)? The two studies were set up in similar ways. The identification of an appropriate case began with finding a reputably successful, qualified and experienced secondary school mathematics teacher who was willing to participate in the research, and who worked in a well-functioning school. These criteria were to ensure that, on the one hand, the teacher had a reasonable foundation of mathematical knowledge and, on the other, that we would capture teaching in situations where not only was the teacher qualified and the school functional in the technical sense, but also where teaching was professional: the teacher was recognized by the school hierarchy and or/the wider staff and field as competent and committed. The second step was to negotiate with the teacher the observation and discussion of a particular topic and set of lessons over at least one week. In both studies the identification of these teachers was also opportunistic in nature since in the case of the functions study, one of us (VP) knew the principal of the school and this gave him access to the school. Whilst in the case of the probability study, he knew the teacher and the teacher in this instance was the principal of the school. Both teachers observed taught at public schools in Gauteng. Data were collected in 2005, prior to the introduction of the new FET curriculum in Grade 10.
With the functions study, Nash6 had had previous experience teaching the section of linear functions to Grade 10 learners and observation revealed his teaching strategy as quite typical of secondary mathematics teaching. He presented his learners with mathematical ideas or concepts, provided examples of how to carry out procedures to solve related tasks, whereafter learners practised these through textbook set exercises. This kind of pedagogic practice is often referred to as traditional.7 With the probability study, although Vuyo8 was an experienced mathematics teacher, he was teaching the topic for the first time. He used an activity-based approach which was in line with the principles governing the new curriculum currently being phased in to South African schools and already implemented in Grade 8.
It is important to take cognisance of the fact that in both studies the intention was not to evaluate the teachers. Neither was our intention in contrasting the work of these two teachers to judge one or the other as 'better' in any way. Our purpose was to learn from them the mathematical demands of teaching these different topics, viz. linear functions and probability, in the ways that they had chosen, and through this to further our understanding of the mathematical work of teaching.
Methodology, data collection and analysis
To engage in this type of investigation of the mathematical work of teaching that Nash and Vuyo grappled with as they went about their work, we had to observe the teachers in practice. Structured observations were done while the teachers were teaching and we were non-participant observers in the class. An observation schedule was designed based on the categories of teaching discussed below. We also video-recorded all lessons so as to keep a more 'permanent' track of the lessons. The video data made it possible for us to focus in more closely and in greater depth, and also to elaborate categories and expand our analytic frame where needed. All video recordings of the lessons were transcribed. Interviews with the teacher augmented the data-collection strategy. However, it is the video data that we focus here.
In order to describe the teachers' work, we needed to analyse each and all of the lessons in full. We therefore needed to divide up the video transcripts in a way that enabled us to analyse the teachers' work over time. In the wider studies, from which this study was drawn, we describe the methodology and data analysis in more detail (Adler & Pillay, 2007), in particular how and why our unit of analysis was what we call an 'evaluative event'. In Bernstein's (1990; 1996) terms any pedagogy transmits evaluation rules, this is to say, in any pedagogic practice, teachers transmit criteria to learners of what it is they are to come to know. In other words, at various points in time the teacher needs to legitimate aspects of the pedagogic discourse (in relation to what it is he wants learners to know), and in order for the teacher to do this he or she must exercise some form of judgement. We were able to chunk all transcripts into a series of evaluative events, the beginnings of which were marked by the teacher introducing an idea or concept, and the ends of which were marked by a move to legitimate or to fix meaning.
In each of these episodes, we were able to focus on what and how the teacher introduced the concepts or ideas he or she was teaching, the mathematical work of teaching the teachers were faced with and enacted, as well as the resources the teacher called on in this work. Our focus was on the tasks of teaching, elaborated in the next section. We also discuss how the two teachers introduced new ideas, concepts or procedures in their class. We do not focus on the resources called on, what we have described elsewhere as legitimating appeals. The extracts that follow in the Findings section each illustrate an event, as well as present our analysis of how mathematical ideas were introduced, and the teachers' mathematical work that ensued.
The framework used to analyse the mathematical work of teaching in the two case studies was drawn, in part, from Ball et al. (2004). Ball and her co-researchers suggest eight aspects of mathematical work of teaching that teachers could engage with as they go about their work which they describe as:
• Design mathematically accurate explanations that are comprehensible and useful for learners;
• Use mathematically appropriate and comprehensible definitions;
• Represent ideas carefully, mapping between a physical or graphical model, the symbolic notation, and the operation or process;
• Interpret and make mathematical and pedagogical judgements about learners' questions, solutions, problems, and insights (both predictable and unusual);
• Be able to respond productively to learners' mathematical questions and curiosities;
• Make judgements about the mathematical quality of instructional materials and modify as necessary;
• Be able to pose good mathematical questions and problems that are productive for learners' learning; and
• Assess learners' mathematics learning and take the next steps
(Ball et al., 2004:59)
Ball and her co-researchers developed these through a detailed study of teachers' classroom practices, arguing that these tasks of teaching repeatedly appear in teachers' work. This is a practice-based notion of MfT which we found useful in our study of classroom teaching of functions and probability. However, we condensed Ball et al.'s eight categories into six because we found some of the eight aspects overlapping. Specifically, responding to learners questions, interpreting and making pedagogical judgements about learners' productions, and assessing learners' mathematical learning and taking next steps, are all about working with students' ideas. Our six categories were as follows:
• Defining — attempts to provide a definition
• Explanations — teachers explain an idea or procedure
• Representations — teachers represent ideas and in various ways
• Working with learners' ideas — teachers engage with both expected and unexpected learners' mathematical ideas
• Restructuring tasks — teachers change set tasks by scaling them either up or down
• Questioning — teacher asks questions to move the lesson on
There is a shift in how we describe these categories. Our interest was in capturing what the teachers did irrespective of whether this was correct, appropriate, or productive. In the extract below, which illustrates an event, we point to how we recognised these teaching tasks in our data. Across all lessons observed, we recorded all the instances where the teacher responded to a demand for, or judged the need for, a definition, an explanation, a representation, or any of the other aspects above, as the lesson progressed.
For example, in Extract 1, taken from Nash's lessons on functions, a learner asks a question to which he responds with an explanation. Therefore our analysis recorded this as an explanation.
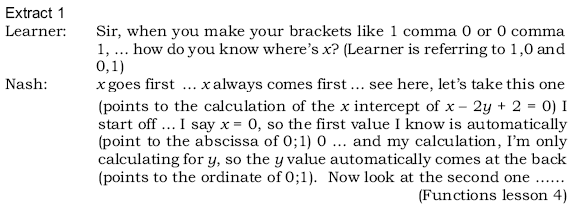
Extract 2 is from Vuyo's lessons on probability. It also illustrates the teacher providing an explanation. This explanation did not follow a question from a learner as above, but rather followed interactions with learners on possible outcomes from a head/tail coin-spinning activity, where it was evident that learners did not distinguish between HT and TH as two different outcomes. This extract is thus at the same time an illustration of the teacher working with learner ideas. The full event (in the methodological sense) from which this extract is drawn is elaborated later and includes discussion of how the activity was introduced, the interactions that followed, and all the teaching tasks that were evident

In the two extracts above we have provided illustrations of how we recognised one of the tasks of teaching (particularly providing explanations), as these appeared in both sets of data. In each example, meaning is legitimated through an explanation by the teacher. The nature of the explanations are clearly different and while this aspect of the two studies (the nature of legitimating appeals) is not in focus in any detail in this article, we will discuss this further later. Our recognition of the other tasks of teaching followed a similar process.
Findings
In order to gain some insight into the mathematical work of teaching in each of the two studies, it was necessary for us to exhaust all the data as this provided us with a comprehensive grasp of the teachers' work. This enabled us to see the mathematics for teaching as it was demonstrated by each of the teachers. This therefore led to tallying occurrences so that we could obtain a picture of presence or absence and frequency. In this sense quantification was used to structure an overview of the data analysis. Tables 1 and 2 succinctly capture the mathematical work of teaching that each of the teachers engaged with.


From the two tables we see the different emphases in the mathematical work of teaching that the two teachers enacted. Firstly, we can see that while Vuyo was mostly working with learners' ideas and restructuring tasks, Nash mostly engaged in explanations and representations. It is also intriguing to note that, while Vuyo worked across all these tasks of teaching (though in greater and lesser degrees), we recorded no occurrences when Nash was either engaged with learners' ideas or restructured tasks.
In the next section we provide extracts from each of the teacher's lessons. We focus first on the most predominant work of teaching that each of the two teachers engaged in: thus working with learners' ideas for Vuyo and explanations for Nash. The extracts we selected illustrate these. They also illuminate the way in which each teacher introduced ideas in their lessons. It was in the inter-relationship between these that we were able to interpret and explain the different mathematical work done by these two teachers.
Probability
In one of the lessons observed, Vuyo gave his class an activity that required the learners to investigate all possible outcomes from tossing two coins. This marked the beginning of an evaluative event for analysis. The learners were asked to practically toss the coins and record all the possible outcomes. The introduction to this aspect of probability was then categorised as through an activity. Learners worked in groups and recorded their work on charts. Later groups presented their solutions to the rest of the class. Extract 3a shows part of the discussion that followed the activity where one group was presenting to the class. In the extract, H represents 'heads' and T represents 'tails' on a coin.

Whole-class discussion followed where learners demonstrated that they considered HT and TH the same. Order did not seem to matter to them. The discussion made reference to a coin game called kapukapu which the learners were familiar with. Kapukapu is a game where two players chose either heads or tails, then a number of coins are thrown in the air and allowed to land on the ground. The number of heads and the number of tails are counted and the winner is one with the highest number. In the kapukapu game, order does not matter, only the total number of heads and tails. Similarly, learners thought that in the class activity what mattered was the total number of heads and tails and not the order. Some learners did not even seem to understand that there was an order. This is understandable because in a kapukapu game that the learners were familiar with, HT or TH would be reported as one head and one tail, order is not part of the game. At this moment during the lesson, Vuyo had to work with the learners' ideas (that order did not matter) to effectively get the learners to understand the concept of 'all possible outcomes' where order does matter. Consequently, the mathematical work of teaching that was demanded of Vuyo was to work with learners' ideas, in particularly here their intuitive and everyday notions of head/tail options.
Of course as Vuyo dealt with the learners' ideas, he also engaged in other mathematical tasks of teaching such as explaining or questioning. Across the lessons, there were many instances where there were additional demands on the teacher while he was working with learners' ideas. Hence the spread of mathematical work evident in Table 1.
As an illustration, further on during the discussion in Vuyo's class, learners still seemed not to understand that order mattered; they still thought that HT and TH were the same thing. In an attempt to demonstrate to his learners that HT and TH were different outcomes, Vuyo explained as shown in Extract 3b:

We see here that Vuyo offers an example of animals to explain the difference between HT and TH in a coin toss. It is important to note that Vuyo's explanation as shown in the above example followed the discussion with learners which revealed their ideas. In observing the probability lessons, there were many of such instances where learners' ideas were evident. This could be because probabilities are visibly experienced in everyday life. Learners thus bring their everyday knowledge and experiences to the classroom. In particular, the activity here of coin tossing brings into the mathematics class objects used in everyday life, be these as money or within a game as discussed.
More significant than the fact of the coins was the nature of the activity. Vuyo's activity-based tasks provided opportunities for the learners to talk about their everyday experiences. Consequently, learners' ideas derived from their everyday experiences were exposed, and the teacher needed to work with such ideas in order to move the lesson on. One of the demands in the teaching of probability then is the conflict between learners' experiences and the counter-intuitive concepts or mathematical notions related to probability.9 The unpacking Vuyo needed to do in relation to a probability event and all possible outcomes required that he work between learners' intuitions and mathematical foundations. This opens the question as to whether coin tossing activity (seen in many textbooks) is appropriate for establishing, in Tall's terms, a cognitive root for the notion of an event and all possible outcomes in probability theory. It is our contention that this question points to one of the key aspects of mathematics for teaching, and illuminates that this kind of knowledge for teaching has topic specificity.
Functions
During lesson 3 on functions, Nash demonstrated, on the board, how to draw the graph of the function: 3x - 2y = 6. He used the dual intercept method of substituting 0 for x and y in the function to get the y-intercept and the x-intercept. For this example he got the points (0,-3) and (2,0), respectively. Extract 4 presents the discussion that followed.

Extract 4 is an example of how Nash conducted his lessons. His explanations dominated the lessons. Learners participated mostly by responding to some of his questions, asking questions of clarification on what to do, and doing some class exercises. As can be seen from the extract, learners were asking questions to which Nash responded with explanations. We did not classify these as working with learners' ideas, as the questions were about what to do. Looking at Nash's explanations, we see that the most common explanations were procedural, following questions from learners about what to do (for example, Learner 1's question of whether they didn't need other points to draw the straight line graph). Procedural explanations were also observed when Nash was commenting on learners' written work. This work of explaining mathematics stands in contrast to the dominant work Vuyo did in his class.10
Discussion
So, how are these differences observed in the mathematical work done by Vuyo and Nash to be interpreted and explained? What work did they (need to) do? What insights into the notion of mathematics for teaching follow?
In order to provide an answer to these questions we first consider the manner in which the two teachers chose to introduce the concepts in question. In the case of probability, Vuyo introduced concepts through activity- based tasks which brought out learner productions either anticipated or un- anticipated. The teacher therefore needed to work with the learners' ideas in order to proceed with the lesson and move learners from their intuitive notions to mathematical notions. Vuyo's learners had their first encounter with aspects of probability in a form of activities, which often brought out ideas from learners' everyday experiences. Vuyo then had to work from the learners' everyday knowledge or prior experiences to the mathematical idea he wanted them to grasp.
In comparison, in Nash's lessons on linear functions, first encounters were with a range of representations of a linear function. In the extract above, Nash (re)presents a linear equation in a particular form, from which the line is best drawn using the dual intercept method. Nash's work was then on exemplifying the important components of this procedure. This followed an initial representation of a linear function as a set of ordered pairs in a table of values, and then as an equation in standard form where a line could be drawn using the gradient-intercept method. In extract 4, Nash's students asked questions. However, these questions did not lead Nash to engage with their thinking. He was able to move his lessons on with explanations of what to do. The spread of explanations prevalent across Nash's lessons were either solicited by students through questions or errors, or a function of Nash's own judgement that an explanation was needed.
So what did we learn from these two teachers, and their work as they taught probability and functions in their respective classrooms? In the first instance, we suggest that their work differed. Both teachers responded to learners and engaged in explanations. The emphases, however, differed considerably. For Vuyo to move from the activity he set to the probability ideas he wanted his learners to understand, he was confronted with intuitive reasoning on the one hand, and everyday knowledge on the other. We have illustrated that these demands were a function both of the nature of probability and the way in which he chose to introduce learners to these ideas. Probability invokes everyday knowledges and these are often counter to mathematical orientations to the same 'notion'. Moreover, task-based activity provides the space for these intuitions to become visible in the lessons.
Of course, probability lessons do not need to proceed in this way. A teacher could present formal definitions of probability concepts, together with a set of rules or formulae to solve typically problems, and some examples of how to do such calculations (e.g. the number of possible outcomes in an event). In this case, it is less likely that the kinds of demands Vuyo faced would materialise. He would be able to proceed with explanations, similar to how Nash proceeded in his class. And it is possible, that as in Nash's class, many learners would be successful in executing these.
In the same way, lessons on linear functions could proceed through task- based activity. Nash would likely then have to work with how learners interpret the tasks, the meanings they bring, and those he wishes them to grasp. Depending on the kinds of activities used to introduce functions, learners' everyday knowledge and experience might too enter the class and require engagement by the teacher.
Our point is that the mathematical work of teaching is complex, and not adequately captured in categories of tasks of teaching in and of themselves. The work of these teachers reveals that the topic being taught matters, as does they way in which teachers approach the topic and how they introduce mathematical ideas in class. Activity-based teaching is likely to illicit learners' ideas. Mathematics for teaching with activities thus entails working with learners' ideas. In the case of probability, there are significant demands on the teacher here as intuitive notions, structured as they are by everyday experience, run counter to related mathematical notions. They cannot be easily ignored or passed over. Elsewhere (Kazima & Adler, 2006) we have shown that Vuyo indeed faces significant challenges in moving between learners' intuitions, his visual examples that attempt to move these notions towards mathematical ones, and then the formal mathematical notions themselves. In contrast, if linear functions are taught through tightly sequenced representations coupled with ongoing explanations, then learners' thinking does not need to be engaged. This is evident in Nash's lesson when he responds procedurally to a question of whether "you don't need all the other parts". This was an opportunity to probe the learner's thinking, and to have the class puzzle over whether and why two points define a line, so illuminating his assertion of the efficiency of the dual intercept method for drawing a line. Hence our argument that MfT is a function of the topic being taught together with the teachers' approach to teaching, which includes the way ideas are introduced.
It is in this sense then that the categories of teaching tasks (the mathematical work that teachers do) offered by Ball et al. (2004) are useful only to a point. Each needs to be understood more specifically in relation to particular topics in mathematics, and to particular approaches to teaching. In addition, one task not identified, yet suggested by our study, is what others have called 'first encounters'.
Conclusion and implications
There are a number of important questions that arise particularly in relation to mathematics teacher education? Our studies confirmed that the tasks identified by Ball et al. (2004) are not only mathematical, but they take on specific meanings across topics, and across different approaches to teaching. And so the question we posed at the beginning of the article: where do prospective teachers get to know about and be able to engage with, for example, learner thinking; with the range and rationales of possible first encounters; with ways of unpacking notions like the linear functions? What opportunities are there for practising teachers to come to understand that a different approach to teaching requires that they engage in new ways with mathematical thinking and mathematical tasks. And where might these be learned? The main rationale for mathematics teacher education is to prepare and support teachers to teach mathematics well. How then do mathematics teacher education programmes include these mathematical foci that are specific to teaching? How do these programmes provide sufficient engagement with all the relevant topics in the school curriculum? Typically, teacher education includes courses in the subjects that do not extend to include related tasks of teaching. These are dealt with at a general level in methodology courses. Topic-specific tasks of teaching and their relation to approaches to teaching mathematics are rarely addressed. This is a significant challenge for mathematics teacher education, which needs to be embraced if our goals for improving the preparation and support of mathematics teachers, and so the quality of mathematical teaching, are to be realised.
Acknowledgements
This work formed part of the QUANTUM research project on Mathematics for Teaching, directed by one of us (JA) at the University of the Witwatersrand. The material is based upon work supported by the National Research Foundation under Grant number FA2006031800003.
Notes
1. QUANTUM is the name given to an R & D project on quality mathematical education for teachers in South Africa. See Adler and Davis (2006a) for elaboration of the first phases of the project.
2. The further education and training band is the term used to describe the grouping of Grades 10, 11 and 12 in secondary schools across South Africa.
3. Reform in education in South Africa led to the implementation and phasing in of a new curriculum which is still in the process of being phased in.
4. Barbé et al. (2005:240) describe the notion of institutionalised mathematics as "the teacher has some 'given data', such as curricular documentation, textbooks, assessment tasks, national tests, etc. where some components of a mathematical organisation, as well as some pedagogic elements and indications on how to conduct the study, can be found. This is how the educational institution 'informs' the teacher about what mathematics to teach and how to do so". We would add that classroom interactions — didactic practice — also shape what comes to be mathematics in school.
5. For detailed reports on these studies see Pillay (2006a ; 2006b) and Kazima and Adler (2006), respectively.
6. Pseudonym used to refer to the teacher in the functions study.
7. 'Traditional' is typically used to capture and refer to an approach to mathematics that relies on a textbook where the pattern of mathematical presentation is a description of a rule, convention or procedure, followed by a few examples and then an exercise. Investigative tasks, following a more inductive approach to a concept, thus building on learner activity is typically absent from these kinds of textbooks. However, these textbooks have served the curriculum demands of the day more than adequately.
8. Pseudonym used to refer to the teacher in the probability study.
9. See Kazima and Adler (2006) for further discussion of this aspect of teaching probability.
10. There is much that is interesting in this extract, both in relation to the learners' question and Nash's responses. The way in which these were handled focuses on what to do. The questions about other points can be interpreted as profoundly mathematical — how many points define a line? The way this was dealt with reflected the resources drawn on by the teacher in his explanations, and these repeatedly turned on procedures and what to do. This aspect of MfT lies in legitimating appeals in use, and is therefore beyond the scope of this paper.
References
Adler J 2004. Researching inside teacher education: the QUANTUM project, its context, some results and implications. Paper presented at the AERA, San Diego. [ Links ]
Adler J & Davis Z 2006a. Opening another black box: Researching mathematics for teaching in mathematics teacher education. Journal for Research in Mathematics Education, 37:270-296. [ Links ]
Adler J & Davis Z 2006b. Studying mathematics for teaching inside teacher education. Paper presented at the 14th Annual Meeting of the Southern African Association for Research in Mathematics, Science and Technology Education, University of Pretoria, South Africa. [ Links ]
Adler J & Pillay V 2007. An Investigation into Mathematics for Teaching: Insights from a case. African Journal of Research in Mathematics, Science and Technology Education, 11:87-108. [ Links ]
Adler J & Huillet D (forthcoming). The social production of mathematics for teaching. In: Wood T (series ed.) & Sullivan P (volume ed.). International handbook of mathematics teacher education: Vol.1. Knowledge and beliefs in mathematics teaching and teaching development. Rotterdam, The Netherlands: Sense Publishers. [ Links ]
Ball DL & Bass H 2000. Interweaving content and pedagogy in teaching and learning to teach: knowing and using mathematics. In: Boaler J (ed.). Multiple Perspectives on Mathematics Teaching and Learning. Wesport: Ablex Publishing. [ Links ]
Ball DL, Bass H & Hill H 2004. Knowing and using mathematical knowledge in teaching: Learning what matters. Paper presented at the 12th Annual Conference of the Southern African Association for Research in Mathematics, Science and Technology Education, Durban, South Africa. [ Links ]
Ball DL, Lubienski S & Mewborn D 2001. Research on teaching mathematics: The unsolved problems of teachers' mathematical knowledge. New York: Macmillan. [ Links ]
Barbé J, Bosch M, Espinoza L & Gascón J 2005. Didactic restrictions on the teacher's practice: The case of limits of functions in Spanish high schools. Educational Studies in Mathematics, 59:235-268. [ Links ]
Bernstein B 1990. Class codes and control: the structuring of pedagogic discourse. London: Routledge. [ Links ]
Bernstein B 1996. Pedagogy, symbolic control and identity: theory research, critique. London: Taylor & Francis. [ Links ]
Even R 1990. Subject matter knowledge for teaching and the case of functions. Educational Studies in Mathematics, 21:521-544. [ Links ]
Hill HC, Rowan B & Ball DL 2005. Effects of Teachers' Mathematical Knowledge for Teaching on Learner Achievement American Educational Research Journal, 42:371-406. [ Links ]
Kazima M & Adler J 2006. Mathematical knowledge for teaching: Adding to the description through a study of probability in practice. Pythagoras, 63:46-59. [ Links ]
Marks R 1992. Pedagogical content knowledge: from a mathematical case to a modified conception. Journal of Teacher Education, 41:3-11. [ Links ]
Ma L 1999. Knowing and teaching elementary mathematics: teachers' understanding of fundamental mathematics in China and the United States. New Jersey: Lawrence Erlbaum. [ Links ]
McNamara D 1991. Subject knowledge and its application: Problems and possibilities for teacher educators. Journal of Education for Teaching, 17:113-128. [ Links ]
Pillay V 2006a. An investigation into mathematics for teaching; the kind of mathematical problem-solving a teacher does as he/she goes about his/her work. Research Report. Johannesburg: University of the Witwatersrand. [ Links ]
Pillay V 2006. Mathematical knowledge for teaching functions. Paper presented at the 14th Annual Meeting of the Southern African Association for Research in Mathematics, Science and Technology Education, University of Pretoria, South Africa. [ Links ]
Sanchez V & Llinares S 2003. Four learner teachers' pedagogical reasoning on functions. Journal of Mathematics Teacher Education, 6:5-25. [ Links ]
Shulman LS 1986. Those who understand knowledge growth in teaching. Educational Researcher, 15:4-14. [ Links ]
Shulman LS 1987. Knowledge and teaching: foundations of a new reform. Harvard Educational Review, 57:1-22. [ Links ]
Skemp R 1987. Relational Understanding and Instrumental Understanding. Mathematics Teaching, 77:20-26. [ Links ]
Stacey K, Helme S, Steinle V, Baturo A, Irwin K & Bana J 2001. Preservice teachers' knowledge of difficulties in decimal numeration. Journal of Mathematics Teacher Education, 4:205-225. [ Links ]
Tall D 1989. Concept Images, Generic Organizers, Computers & Curriculum Change. For the Learning of Mathematics, 9:37-42. [ Links ]














