Serviços Personalizados
Artigo
Indicadores
Links relacionados
-
 Citado por Google
Citado por Google -
 Similares em Google
Similares em Google
Compartilhar
South African Journal of Science
versão On-line ISSN 1996-7489
versão impressa ISSN 0038-2353
S. Afr. j. sci. vol.116 no.5-6 Pretoria Mai./Jun. 2020
http://dx.doi.org/10.17159/sajs.2020/6871
RESEARCH ARTICLE
Updated lower limb stature estimation equations for a South African population group
Mubarak A. BidmosI; Desire BritsII
ICollege of Medicine, QU Health, Qatar University, Doha, Qatar
IIHuman Variation and Identification Research Unit, School of Anatomical Sciences, University of the Witwatersrand, Johannesburg, South Africa
ABSTRACT
One of the main steps in the identification of an unknown person, from their skeletal remains, is the estimation of stature. Measurements of intact long bones of the upper and lower extremities are widely used for this purpose because of the high correlation that exists between these bones and stature. In 1987, Lundy and Feldesman presented regression equations for stature estimation for the black South African population group based on measurements of bones from the Raymond A. Dart Collection of Human Skeletons. Local anthropologists have questioned the validity of these equations. Living stature measurement and magnetic resonance imaging scanograms of 58 adult volunteers (28 males and 30 females) representing the modern black South African population group were obtained. Physiological length of the femur (FEPL) and physiological length of the tibia (TPL) were measured on each scanogram and substituted into appropriate equations of Lundy and Feldesman (S Afr J Sci. 1987;83:54-55) to obtain total skeletal height (TSHL&F). Measured total skeletal height (TSHMeas) for each subject from scanograms was compared with TSHL&F Both FEPL and TPL presented with significantly high positive correlations with TSHMeas. A comparison between TSHL&F and TSHMeas using a paired t-test, showed a statistically significant difference - an indication of non-validity of Lundy and Feldesman's equations. New regression equations for estimation of living stature were formulated separately for male and female subjects. The standard error of estimate was low, which compared well with those reported for other studies that used long limb bones.
SIGNIFICANCE:
• Statistically significant differences were observed between measured and estimated skeletal height, thus confirming non-validity of Lundy and Fieldsman's (1987) equations for lower limb bones.
• New regression equations for living stature estimation were formulated for femur and tibia lengths, and the low standard error of estimates of equations compared well to results from other studies.
Keywords: living stature, estimation, forensic anthropology, MRI scanogram
Introduction
Estimation of stature from complete skeletons (anatomical method) or from individual/combination measurements of bones (mathematical method) forms a necessary part of the process of establishing the biological profile of an individual from recovered or discovered skeletons. The former method has been reported to produce accurate estimates of stature and is neither population nor sex-specific.1-3 However, it has the disadvantages of being time consuming and very tedious.2 In addition, the anatomical method can be used for estimation of stature only if an intact and complete skeleton is available, which is considered a luxury in forensic cases. Consequently, the latter method, i.e. the mathematical method, is the most often used method in the absence of a complete skeleton or when bones are recovered in fragmentary states.
The mathematical method is based mainly on a statistical theorem known as regression analysis. This involves the formulation of regression equations from individual measurements or combinations of measurements of intact and fragmentary bones of the skeleton and percutaneous bones. This method is less time-consuming and tedious than the anatomical method and is considered more applicable in most forensic cases. However, the mathematical method is both population and sex specific. It therefore requires that equations for the estimation of stature need to be formulated for different population groups and at appropriate intervals in order to account for temporal changes.4 There has been a plethora of studies on stature reconstruction using measurements of long bones of upper and lower limbs in different parts of the world following the publication of arguably the largest study on stature reconstruction by Trotter and Gleser4 in 1958. Regression equations have been formulated for populations including, but not limited to, the Portuguese5, Germans6, Bulgarian7, Polish8, Turks9, Croatians10, Mexicans11, Spaniards12, Koreans13 and Japanese14. Regression equations have also been formulated from measurements of fragments of long bones for stature reconstruction15 and other bony elements (e.g. clavicle16, skull17, scapulae18, metacarpals,19 vertebrae20, sacrum21, calcaneus22 and metatarsals23) as long limb bones are often recovered in forensic and archaeological practice in fragmentary states.
In South Africa, a country with a high crime rate, similar regression equations have been formulated from intact long bones24,25, fragments of long bones26, the skull27, sacrum28, metatarsals29 and calcaneus30. In 1983, Lundy31 conducted the first ever study on stature reconstruction in South Africa. Lundy31 used Fully's1 method in calculating total skeletal height (TSH) which was later regressed on maximum lengths of humeri, radii, ulnae, femora, tibiae and fibulae. Regression equations were derived separately for male and female black South Africans.31 Lundy and Feldesman24 revised the regression equations due to some errors in the computer program handling some data. The regression equations developed by Lundy and Feldesman24 are the most frequently used stature estimation equations when dealing with black South African skeletal remains; however, results from an unpublished study by Arendse32 highlighted the need to re-examine these equations, specifically in modern black South Africans. The validity of these equations on a contemporary black South African population has been questioned, as these equations were derived more than three decades ago, using skeletal remains housed in the Raymond A. Dart Collection of Human Skeletons. Regrettably, many skeletal collections do not represent the populations from which they were derived as collections often have an over-representation of the elderly and individuals from lower socio-economic strata.33,34 Additionally, the effects of secular trends on populations also often render skeletal collections unrepresentative of their modern counterparts.35,36 As such, many studies are using modern image modalities of living individuals to study skeletonised remains.12,18,20,37,38 Because there has not been any attempt to test the validity of these equations on living individuals, the aim of this study was to investigate the validity of some of Lundy and Feldesman's equations24 on a sample of living black South Africans using data collected from magnetic resonance imaging (MRI) scanograms and to calculate new equations, if necessary.
Subjects and methods
Participants
Prior to the commencement of the study, ethics approval was obtained from the Human Research Ethics Committee of the University of the Witwatersrand, Johannesburg, South Africa (clearance certificate number M180788) to access data collected in two previous studies by Bidmos and Manger37 and Brits et al.38 Data used in the current study and how they were obtained have been described in previous studies.37,38 Participants in these studies37,38 were individuals from diverse South African black ethnological groups. As previous studies31,39 have shown little intertribal variations amongst black South Africans, they were considered a single homologous group. Furthermore, Franklin et al.40 reported the disappearance of tribal subdivisions, possibly due to inter-marriage between individuals of different groups. More than 88 individuals were approached to participate in both studies.37,38 However, only data from a final sample of 58 participants (28 males and 30 females) were analysed. The individual measurements of each participant are provided in Supplementary table 1.
Measurements
Living stature of participants was measured, and thereafter, full body MRI scans were collected. Measurement of the living stature (LSM) of each of the participants was taken with a stadiometer on the morning of the MRI scan. This procedure became necessary because of the documented loss of stature during the day.41 Full body MRI scans were carried out at the Wits Donald Gordon Medical Centre in Johannesburg, South Africa. Each participant was scanned in a supine position as documented in previous studies37,38 and the scanned images were then transferred to a DVD. A suite of measurements as described in previous studies37,38 was taken on each scanogram using OsiriX42. These measurements are height of cranium, height of axis (C2), height of vertebrae (C3 to L5), height of first sacral vertebra, physiological (bicondylar) length of the femur, physiological length of tibia and talus-calcaneal height. The sum total of these measurements gave the measured total skeletal height (TSHMeas). Two of these measurements defined and illustrated below were used in the assessment of the validity of Lundy and Feldesman equations24:
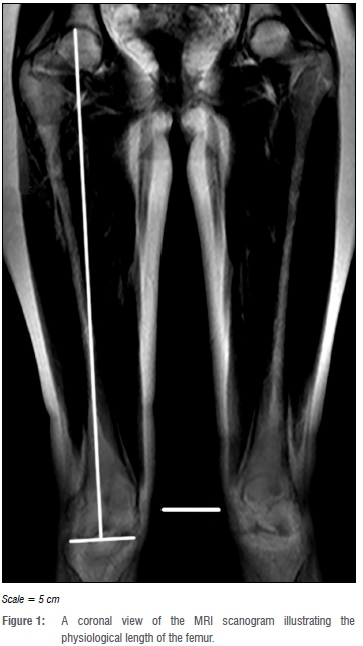
1. Physiological (bicondylar) length of the femur (FEPL): The linear measurement between the most superior projecting point of the head of the femur and a line connecting the most inferior aspects of the femoral condyles38 (Figure 1). This measurement was taken on coronal images.
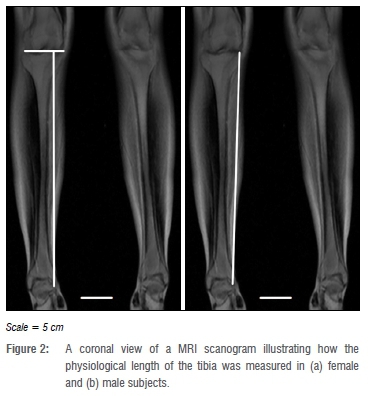
2. Physiological length of the tibia (TPL): The physiological length of the tibia as described by Lundy43 was measured by excluding the intercondylar eminence of the tibia while including the medial malleolus. In the female sample, the physiological length of the tibia was measured between the tip of the medial malleolus and a line drawn parallel to the superior aspect of the lateral tibial condyle38 (Figure 2a). For the male sample, the physiological length of the tibia was measured from the tip of the medial malleolus to the superior aspect of the medial condyle37 (Figure 2b). This measurement was taken on coronal images. As no guidelines are available for osteometric data collection from MRI scans, the two studies explored various ways to collect the tibial length as reliably and accurately as possible.
The FEPL and TPL measurements were summed to produce an additional skeletal measurement. These measurements were used in conjunction with the stature estimation equations for the femur and tibia developed by Lundy and Feldesman24 to estimate total skeletal height (TSHL&F), as per the equations below:
Males
Total skeletal height = 45.721 x 2.403(femur - physiol) ±2.777
Total skeletal height = 60.789 x 2.427(tibia - physiol) ±2.78
Total skeletal height = 46.543 x 1.288(femur + tibia) ±2.371
Females
Total skeletal height = 27.424 x 2.769(femur - physiol) ±2.789
Total skeletal height = 55.968 x 2.485(tibia - physiol) ±3.056
Total skeletal height = 34.617 x 1.41(femur + tibia) ±2.497
Data analysis
Prior to data collection for the current study, a test of intra-observer repeatability was performed using Lin's concordance co-efficient of reproducibility.44 A total of 20 individuals were measured for this purpose and after confirming that the measuring technique was satisfactory (Lin's concordance correlation coefficients for all measurements were between 0.95 and 0.99), data were collected separately for males and females and captured into MS Excel sheets. Thereafter, descriptive statistics were obtained separately for male and female samples using IBM SPSS (version 24). In addition, normality of data was tested and verified for both sexes.
The accuracy of regression equations derived by Lundy and Feldesman24 for estimation of stature of male and female black South Africans using FEPL, TPL and a combination of both measurements was assessed. For each subject, total skeletal heights (TSHL&F) were calculated from (1) FEPL, (2) TPL and (3) a combination of FEPL and TPL using the appropriate regression equation of Lundy and Feldesman24. The estimated total skeletal height using Lundy and Feldesman's24 equations (TSHL&F) was compared with the measured total skeletal height on the MRI scanograms (TSHMeas) published by Bidmos and Manger37 and Brits et al.38, using a paired t-test. Regression analyses were subsequently performed. Firstly, living stature was regressed on FEPL and TPL. Secondly, a regression equation for a combination of both measurements was obtained for both sexes separately. From these analyses, the unstandardised coefficients and constants were obtained in addition to the correlation coefficient (r) and standard error of estimate (SEE).
Results
The ages of female subjects ranged between 19 and 60 years, with a mean of 38 years (s.d. = 11.2). Male subjects were of a similar age -between 18 and 56 years with a mean age of 35 years (s.d.=10.5). The majority of male and female subjects (70%) fell within the 21-45-year age bracket. There is no statistically significant difference between the mean ages of both sexes (Table 1). The means and standard deviations for LSM, TSHMeas, FEPL and TPL are also shown in Table 1. Mean values of all measurements of male subjects were statistically significantly higher than those for female subjects (Table 1).
Measured values of FEPL, TPL and the combined measurement of FEPL and TPL were substituted into the appropriate sex-specific regression equations of Lundy and Feldesman24 to estimate total skeletal height (TSHL&F). TSHL&F was compared with TSHMeas using a paired f-test. Table 2 shows that a statistically significant difference exists between TSHMeas and calculated TSHL&F using Lundy and Feldesman's24 equations for FEML, TPL and the sum of FEML and TPL. These results indicate that regression equations previously derived for skeletal height estimation by Lundy and Feldesman24 using FEPL, TPL and a combination of these measurements are no longer valid for male and female black South Africans (Table 2).
Therefore, new regression equations specific for the direct estimation of living stature were calculated from FEPL, TPL and the sum thereof for black South Africans. The correlations between LSM and each of the measured variables - namely FEPL, TPL and a combination of FEPL and TPL - were strong and statistically significant (p<0.0001; Table 3).
In the female sample, FEPL displayed the strongest correlation with LSM (r=0.879, r2=0.773) while the lowest correlation was obtained for the regression equation generated for TPL (r=0.792, r2=0.627). The SEE for the equations ranged between 2.56 and 3.28 cm (Table 3). In the male sample, a combination of FEPL and TPL was most strongly correlated (r=0.921, r2=0.848) with LSM. FEPL and TPL each presented similar correlations (r=0.878, r2=0.771) with LSM. The lowest SEE was obtained for the regression equation derived using a combination of FEPL and TPL (2.10 cm). The SEE for regression equations formulated separately for FEPL and TPL was 2.58 cm (Table 3).
Discussion
In the current study, MRI was used to study the components of the skeletons that constitute stature of living individuals. MRI was selected as the imaging modality as it does not expose participants to high doses of harmful ionising radiation as is the case with X-ray and computed tomography (CT).45 Although MRI is not usually used to examine skeletal remains, it has been found that measurements obtained from these scans are comparable to those obtained from CT and dry bones.45 Furthermore, as is evident from the intra-observer repeatability scores, MRI measurements are easily reproducible. By studying living individuals, the researchers were able to measure living stature as opposed to relying on often over-reported statures46 or questionable cadaveric lengths reported in skeletal collections31. It has been shown that cadaveric length is greater than living stature47 and therefore stature estimation methods using cadaveric length tends to overestimate living stature48. To adjust for this, a correction factor of 25 mm was proposed by Trotter and Gleser49. However, a recent study by Cardoso et al.47 showed that the difference between cadaveric length and living stature is greater than initially proposed with an average difference of about 40 mm, and, as such, there is no consensus yet on the adjustment factor required.
By using measured living stature, researchers also did not have to make use of estimates of living stature produced using the anatomical (Fully1) method. This method is considered to be an accurate method for the estimation of skeletal height because it takes into account all the skeletal elements that constitute stature.2 It remains the most extensively used method in the formulation of regression equations for stature estimation in South Africa.24,25,30,31 Recently, a number of studies3,37,38 have challenged the accuracy of the anatomical method because of uncertainties regarding applicability of the correction factors for soft tissue that were recommended by Fully1. The stature estimation equations derived by Lundy and Feldesman24 were calculated using the anatomical method and as such the validity and accuracy of these equations need to be assessed in a modern living black South African population. In this study, measurements of living stature ranged between 161 cm and 180 cm (mean = 170.79 cm) for males and between 146 cm and 171 cm (mean = 159.1 cm) for females. These measured living statures are similar to living statures recorded for black South African military personnel50 and are therefore considered representative of the modern black South African population group. The members of the South African military50 represent a sample of living adult population. Consequently, their mean height was compared with the mean height of the individuals in the current study. On average, black South Africans are shorter than black and white North Americans51, white South Africans50 and Spanish males12. However, they are slightly taller than the Portuguese5 and Japanese14 based on cadaveric heights which have been converted to living stature. Direct comparisons of stature are often limited as most stature estimation research relies on either cadaveric height or heights measured during autopsies.8,10,13
The mean femoral and tibial length measurements from scanograms were 45.2 cm (±2.3 cm) and 38.2 cm (±2.1 cm) for males and 43.3 cm (±2.0 cm) and 36.5 cm (±2.1 cm) for females. The mean femoral measurement from the current study for males was smaller compared to those of black and white North Americans49, Spanish12 and white South Africans25 but slightly larger than that reported for Japanese14. The mean femoral measurement for the current sample was comparable to that of black South Africans reported by Lundy43. The mean femoral measurement for females was larger than those reported for Japanese14 but smaller than the average recorded by Lundy43. The measurement is comparable to that of black North Americans49, white South Africans25 and Spaniards12. In addition, mean tibial measurement for females was longer than those reported for black43 and white South Africans25 while the mean tibial measurement for males was comparable to that reported for black24,43 and white South Africans25. A direct comparison of bone lengths with other studies were difficult as some studies report cutaneous bone measurements11, measurements with cartilage7 or maximum measurements as opposed to physiological/bicondylar measurements10,11. Comparisons of tibial measurements were also limited due to variations in the way in which the tibiae were measured.52,53 Furthermore, tibial differences or the lack thereof can also be contributed to the MRI techniques used to measure the bone. No standards for the measurement of skeletal remains from MRI scanograms or other image modalities are currently available or are yet to be validated. However, a pilot study has found no significant difference between the tibial lengths measured from MRI scans and the corresponding dry bone measurement.54 As such, efforts were made to collect data in line with current standard osteometric practices. The differences highlighted above between the various population groups support the need for population-specific equations. All measurements for male subjects were significantly greater than those for female subjects, thus confirming the need for sex-specific regression equations.
Of importance are the differences noted between the femoral and tibial measurements of female black South Africans in the current study compared to those presented by Lundy43. These differences hint at secular trends. Secular trends are often associated with changes in environmental conditions such as nutrition, health and medical care55 and in South Africa could also be related to the abolishment of apartheid. Previously, a lack of secular change in stature and measurements of the femur and tibia were noted in black South African individuals from the early 20th century.56 However, more recent results have found a positive secular increase in stature in black South Africans along with an increase in lower limb lengths in relation to stature.57 The reason for the lack of secular change in males is not fully understood and warrants further research; however, it could in part be related to the small sample sizes in the study. Further supporting secular changes are the statistically significant differences observed between measured total skeletal height and all estimates of total skeletal height using Lundy and Feldesman's24 stature estimation equations. Therefore, as suggested by Meadows and Jantz35 and Myburgh57, new stature estimation equations from lower limb bone measurements of modern black South Africans were calculated in the current study.
All measured variables along with associated regression equations had very strong statistically significant correlations with measured living stature (Table 3). The correlation between stature and the bicondylar length of the femur was similar between males and females; however, the correlation between the physiological length of the tibia and stature was stronger in males. The association between the femoral and tibial measurements, and stature had an equivalent correlation in males; however, the femur had a stronger association with stature in females. The association between the femur and stature in males in the current study was stronger than that reported for black and white Americans49, Spaniards12 and Koreans13, but weaker than that noted for white South Africans25 and black South Africans24 (Table 4). The relationship between the femur and stature in females was stronger than that reported for White Americans49 and Spaniards12, but weaker than associations reported for black South Africans24, white South Africans25 and Koreans13 (Table 4).
The association between the tibia and stature in the current male sample was comparable to that reported for Spaniards12 and white South Africans25 but stronger than that previously noted by Lundy and Feldesman24 for black South Africans (Table 4). The correlation between stature and the tibia in females was weaker than that documented for Spaniards12 and white South Africans25 and that of black South Africans noted by Lundy and Feldesman24 (Table 4). Interestingly, the correlation of the combined femur and tibia measurement and stature in females was not stronger than that of the femur alone, while the combined measurement in males showed the strongest correlation to stature. Many studies have reported very strong associations between lower limb long bones and stature (Table 4), because these bones directly contribute to the overall height of a person.58
The SEE of equations are considered as a measure of accuracy of regression equations.49 The SEE for stature estimation equations derived from the femoral and tibial measurements in the current male sample was smaller than that reported by various authors for different populations, including that reported for black South Africans by Lundy and Feldesman24 (Table 4). This was also true for female femoral measurements with the exception of the SEE reported for white South Africans.25 Interestingly, SEEs from other populations were smaller than the SEE noted for the stature estimation regression equations derived from the tibia in the current female sample (Table 4). The higher SEE related to the female tibial regression equation is not fully understood and could in part be related to secular trends that have been observed in the distal limb of female black South Africans57 or could be associated with the slightly larger standard deviation observed for the female tibial measurement, which might hint at greater variation in this measurement in females.
Presented in Table 3 are equations for the estimation of living stature as opposed to the estimation of total skeletal height which is often the case in South Africa.24,25,27,29 Lundy and Feldesman24 derived their total skeletal height estimation equations using the anatomical method in conjunction with soft tissue correction factors proposed by Fully1 to provide an estimate of stature. A number of researchers3,37,38 have questioned the accuracy and applicability of Fully's1 soft tissue correction factors. Consequently, alternative soft tissue correction factors have been proposed by various authors3,37,38 but there is no consensus on the validity of these factors.
In conclusion, we provide regression equations for the estimation of living stature of black South Africans from measurements of the femur and tibia. These equations, with reasonably low SEEs, do not require the addition of soft tissue correction factors. Regrettably, the sample size of this study was very small due to expenses associated with the collection of full body MRI scans as well as difficulties related to the recruitment of willing participants. As the regression equations proposed here were derived from a small sample size, future studies are encouraged to explore larger sample sizes to validate these equations and also to generate additional stature estimation equations from various skeletal elements, as research has shown that secular trends affect all limbs, especially in black South African populations.57
Acknowledgements
We thank all those who participated in the study. We also acknowledge the invaluable assistance of staff members from the Department of Radiology, Wits Donald Gordon Medical Centre, especially Dr Haagensen, Ms Bussy, Ms Gibbs and Ms Benade. The assistance of Mr Du Plessis from the School of Anatomical Sciences, University of the Witwatersrand, is also acknowledged. This publication was in part made possible by a grant from the Carnegie Corporation of New York. 'The statements made and views expressed are, however, solely the responsibility of the authors.' Additional funding was received from the National Research Foundation (NRF) of South Africa (grant 80655). 'Any opinion, findings and conclusions or recommendations expressed in this material are those of the authors and therefore the NRF does not accept any liability in regard thereto.'
Authors' contributions
Both M.B. and D.B. were responsible for the conception and design of the study; data acquisition, analysis and interpretation; drafting and critically reviewing the manuscript and editing the final version for publication.
References
1. Fully G. Une nouvelle methode de determination de la taille [A new method for determining size]. Ann Med Leg. 1956;35:266-273. French.
2. Lundy JK. A report on the use of Fully's anatomical method to estimate stature in military skeletal remains. J Forensic Sci. 1988;33(2):534-539. https://doi.org/10.1520/JFS11969J [ Links ]
3. Raxter MH, Auerbach BM, Ruff CB. Revision of the Fully technique for estimating statures. Am J Phys Anthropol. 2006;130(3):374-384. https://doi.org/10.1002/ajpa.20361 [ Links ]
4. Trotter M, Gleser GC. A re-evaluation of estimation of stature based on measurements of stature taken during life and of bones after death. Am J Phys Anthropol. 1958;16(1):79-124. https://doi.org/10.1002/ajpa.1330160106 [ Links ]
5. De Mendonça MC. Estimation of height from the length of long bones in a Portuguese adult population. Am J Phys Anthropol. 2000;112(1):39-48. https://doi.org/10.1002/(SICI)1096-8644(200005)112:1<39::AID-AJPA5>3.0.CO;2-%23 [ Links ]
6. Mall G, Hubig M, Buttner A, Kuznik J, Penning R, Graw M. Sex determination and estimation of stature from the long bones of the arm. Forensic Sci Int. 2001;117(1-2):23-30. https://doi.org/10.1016/S0379-0738(00)00445-X [ Links ]
7. Radoinova D, Tenekedjiev K, Yordanov Y Stature estimation from long bone lengths in Bulgarians. Homo. 2002;52(3):221-232. https://doi.org/10.1078/0018-442X-00030 [ Links ]
8. Hauser R, Smolinski J, Gos T. The estimation of stature on the basis of measurements of the femur. Forensic Sci Int. 2005;147(2-3):185-190. https://doi.org/10.1016/j.forsciint.2004.09.070 [ Links ]
9. Sargin OO, Duyar I, Demirçin S. Estimation of stature from the lengths of ulna and tibia: A cadaveric study based on group-specific regression equations. Euras J Anthropol. 2012;3(1):1-9. [ Links ]
10. Petrovecki V Mayer D, Slaus M, Strinovic D, Skavic J. Prediction of stature based on radiographic measurements of cadaver long bones: A study of the Croatian population. J Forensic Sci. 2007;52(3):547-552. https://doi.org/10.1111/j.1556-4029.2007.00419.x [ Links ]
11. Garmendia AM, Sánchez-Mejorada G, Gómez-Valdés JA. Stature estimation formulae for Mexican contemporary population: A sample-based study of long bones. J Forensic Leg Med. 2018;54:87-90. https://doi.org/10.1016/j.jflm.2017.12.019 [ Links ]
12. Munoz JI, Linares-Iglesias M, Suarez-Penaranda JM, Mayo M, Miguens X, Rodriguez-Calvo MS, et al. Stature estimation from radiographically determined long bone length in a Spanish population sample. J Forensic Sci. 2001;46(2):363-366. https://doi.org/10.1520/JFS14973J [ Links ]
13. Lee JH, Kim YS, Lee UY Park DK, Jeong YK, Lee NS, et al. Stature estimation from partial measurements and maximum length of lower limb bones in Koreans. Aust J Forensic Sci. 2014;46(2):330-338. https://doi.org/10.1080/00450618.2013.877078 [ Links ]
14. Chiba F, Makino Y Torimitsu S, Motomura A, Inokuchi G, Ishii N, et al. Stature estimation based on femoral measurements in the modern Japanese population: A cadaveric study using multidetector computed tomography. Int J Legal Med. 2018;132(5):1485-1491. https://doi.org/10.1007/s00414-018-1834-4 [ Links ]
15. Simmons T, Jantz RL, Bass WM. Stature estimation from fragmentary femora: A revision of the Steele method. J Forensic Sci. 1990;35(3):628-636. https://doi.org/10.1520/JFS12868J [ Links ]
16. Torimitsu S, Makino Y, Saitoh H, Sakuma A, Ishii N, Yajima D, et al. Stature estimation in a contemporary Japanese population based on clavicular measurements using multidetector computed tomography. Forensic Sci Int. 2017;275:316.e1-316.e6. https://doi.org/10.1016/j.forsciint.2017.02.037 [ Links ]
17. Kyllonen KM, Simmons-Ehrhardt T, Monson L. Stature estimation using measurements of the cranium for populations in the United States. Forensic Sci Int. 2017;281:184.e1-184.e9. https://doi.org/10.1016/j.forsciint.2017.10.01 [ Links ]
18. Zhang K, Cui JH, Luo YZ, Fan F, Yang M, Li XH, et al. Estimation of stature and sex from scapular measurements by three-dimensional volume-rendering technique using in Chinese. Leg Med. 2016;21:58-63. https://doi.org/10.1016/j.legalmed.2016.06.004 [ Links ]
19. Zaher JK, Al-Ameen NFM, Seedhom AE. Stature estimation using anthropometric measurements from computed tomography of metacarpal bones among Egyptian population. Egypt J Forensic Sci. 2011;1(2):103-108. https://doi.org/10.1016/j.ejfs.2011.03.002 [ Links ]
20. Milani C, Di Stefano M, Isaia G, Panattoni GL. Stature estimation based on vertebral morphometry by dual energy X-rays absorptiometry imaging in Italian females. J Biol Res. 2017;90, Art. #6430. https://doi.org/10.4081/jbr.2017.6430 [ Links ]
21. Pelin C, Duyar I, Kayahan EM, Zagyapan R, Agildere AM, Erar A. Body height estimation based on dimensions of sacral and coccygeal vertebrae. J Forensic Sci. 2005;50(2):294-297. https://doi.org/10.1520/JFS2004010 [ Links ]
22. Zhang K, Fan F, Tu M, Wang YH, Deng ZH. Estimation of stature and sex from calcaneal measurements in Chinese. Aust J Forensic Sci. 2017;49(1):69-77. https://doi.org/10.1080/00450618.2015.1128967 [ Links ]
23. Ibrahim MA, Elelemi AH, Ibrahim MS, Bandy AH. Adult stature estimation from radiographically determined metatarsal length in Egyptian population. J Forensic Radiol Imaging. 2017;11:28-32. https://doi.org/10.1016/j.jofri.2017.10.002 [ Links ]
24. Lundy JK, Feldesman MR. Revised equations for estimating living stature from the long bones of the South African Negro. S Afr J Sci. 1987;83:54-55. [ Links ]
25. Dayal MR, Steyn M, Kuykendall KL. Stature estimation from bones of South African whites. S Afr J Sci. 2008;104(3-4):124-128. [ Links ]
26. Bidmos MA. Estimation of stature using fragmentary femora in indigenous South Africans. Int J Legal Med. 2008;122(4):293-299. https://doi.org/10.1007/s00414-007-0206-2 [ Links ]
27. Ryan I, Bidmos MA. Skeletal height reconstruction from measurements of the skull in indigenous South Africans. Forensic Sci Int. 2007;167(1):16-21. https://doi.org/10.1016/j.forsciint.2006.06.003 [ Links ]
28. Pininski M, Brits D. Estimating stature in South African populations using various measures of the sacrum. Forensic Sci Int. 2014;234:182.e1-182.e7. https://doi.org/10.1016/j.forsciint.2013.08.030 [ Links ]
29. Bidmos MA. Metatarsals in the estimation of stature in South Africans. J Forensic Leg Med. 2008;15(8):505-509. https://doi.org/10.1016/j.jflm.2008.05.007 [ Links ]
30. Bidmos MA. Adult stature reconstruction from the calcaneus of South Africans of European descent. J Clin Forensic Med. 2006;13(5):247-252. https://doi.org/10.1016/j.jcfm.2005.11.010 [ Links ]
31. Lundy JK. Regression equations for estimating living stature from long limb bones in the South African Negro. S Afr J Sci. 1983;79:337-338. [ Links ]
32. Arendse LM. Stature estimation: Evaluating regression equations for different population groups in South Africa [MSc dissertation]. Cape Town: University of Cape Town; 2018. [ Links ]
33. L'Abbé EN, Loots M, Meiring J. The Pretoria Bone Collection: A modern South African skeletal sample. HOMO. 2005;56(2):197-205. https://doi.org/10.1016/j.jchb.2004.10.004 [ Links ]
34. Dayal MR, Kegley AD, Strkalj G, Bidmos MA, Kuykendall KL. The history and composition of the Raymond A. Dart Collection of human skeletons at the University of the Witwatersrand, Johannesburg, South Africa. Am J Phys Anthropol. 2009;140(2):324-335. https://doi.org/10.1002/ajpa.21072. [ Links ]
35. Meadows L, Jantz RL. Allometric secular change in the long bones from the 1800 to the present. J Forensic Sci. 1995;40(5):762-767. https://doi.org/10.1520/JFS15380J [ Links ]
36. Wilson RJ, Herrmann NP, Jantz LM. Evaluation of stature estimation from the database for forensic anthropology. J Forensic Sci. 2010;55(3):684-689. https://doi.org/10.1111/j.1556-4029.2010.01343 [ Links ]
37. Bidmos MA, Manger PR. New soft tissue correction factors for stature estimation: Results from magnetic resonance imaging. Forensic Sci Int. 2012;214(1- 3):212.e1-212.e7. https://doi.org/10.1016/j.forsciint.2011.08.020 [ Links ]
38. Brits D, Manger PR, Bidmos MA. The accuracy of the anatomical method for stature estimation in black South African females. Forensic Sci Int. 2017;278:409.e1-409.e10. https://doi.org/10.1016/j.forsciint.2017.06.004 [ Links ]
39. De Villiers H. Sexual dimorphism of the skull of the South African Bantu speaking Negro. S Afr J Sci. 1968;64(2):118-124. [ Links ]
40. Franklin D, O'Higgins P, Oxnard CE, Dadour I. Discriminant function sexing of the mandible of indigenous South Africans. Forensic Sci Int. 2008;179(1):84. e1-84.e5. https://doi.org/10.1016/j.forsciint.2008.03.014 [ Links ]
41. Sjovold T. Stature estimation from the skeleton. In: Siegel JA, Saukko PJ, Knupfer GC, editors. Encyclopaedia of forensic sciences. London: Academic Press; 2000. p. 276-284. [ Links ]
42. Rosset A, Spadola L, Ratib O. OsiriX: An open-source software for navigating in multidimensional DICOM images. J Digit Imaging. 2004;17(3):205-216. https://doi.org/10.1007/s10278-004-1014-6 [ Links ]
43. Lundy JK. Selected aspects of metrical and morphological infracranial skeletal variations in the South African Negro [PhD thesis]. Johannesburg: University of the Witwatersrand; 1984. [ Links ]
44. Lin LI. A concordance correlation coefficient to evaluate reproducibility. Biometrics. 1989;45:255-268. https://doi.org/10.2307/2532051 [ Links ]
45. Rathnayaka K, Momot KI, Noser H, Volp A, Schuetz MA, Sahama T, et al. Quantification of the accuracy of MRI generated 3D models of long bones compared to CT generated 3D models. Med Eng Phys. 2012;34(3):357-363. http://dx.doi.org/10.1016/j.medengphy.2011.07.027 [ Links ]
46. Maijanen H, Jeong Y Discrepancies between reported and cadaveric body size measurements associated with modern donated skeletal collections. HOMO. 2018;69(3):86-97. https://doi.org/10.1016/j.jchb.2018.06.005 [ Links ]
47. Cardoso HF, Marinho L, Albanese J. The relationship between cadaver, living and forensic stature: A review of current knowledge and a test using a sample of adult Portuguese males. Forensic Sci Int. 2016;258:55-63. https://doi.org/10.1016/j.forsciint.2015.10.012 [ Links ]
48. Cardoso HF. A test of three methods for estimating stature from immature skeletal remains using long bone lengths. J Forensic Sci. 2009;54(1):13-19. https://doi.org/10.1111/j.1556-4029.2008.00916.x [ Links ]
49. Trotter M, Gleser GC. Estimation of stature from long bones of American whites and negroes. Am J Phys Anthropol. 1952;10(4):463-514. https://doi.org/10.1002/ajpa.1330100407 [ Links ]
50. Steyn M, Smith JR. Interpretation of ante-mortem stature estimates in South Africans. Forensic Sci Int. 2007;171(2-3):97-102. http://dx.doi.org/10.1016/j.forsciint.2006.10.006 [ Links ]
51. Fryar CD, Gu Q, Ogden CL. Anthropometric reference data for children and adults: United States, 2007-2010. Vital and Health Statistics Series 11 no. 252. Hyattsville, MD: National Centre for Health Statistics, 2012. [ Links ]
52. Jantz RL, Hunt DR, Meadows L. The measure and mismeasure of the tibia: Implications for stature estimation. J Forensic Sci. 1995;40(5):758-761. https://doi.org/10.1520/JFS15379J [ Links ]
53. Lynch JJ, Brown C, Palmiotto A, Maijanen H, Damann F. Reanalysis of the Trotter tibia quandary and its continued effect on stature estimation of past-conflict service members. J Forensic Sci. 2019;64(1):171-174. https://doi.org/10.1111/1556-4029.13806 [ Links ]
54. Brits DM. Stature estimation in South African juveniles and adult females [PhD thesis]. Johannesburg: University of the Witwatersrand; 2016. [ Links ]
55. Jantz LM, Jantz RL. Secular change in long bone length and proportion in the United States, 1800-1970. Am J Phys Anthropol. 1999;110(1):57-67. https://doi.org/10.1002/(SICI)1096-8644(199909)110:1<57::AID-AJPA5>3.0.CO;2-1 [ Links ]
56. Tobias PV. Adult stature in Southern African Negroes - further evidence on the absence of a positive secular trend. S Afr Med J. 1990;78(7):97-101. [ Links ]
57. Myburgh J. Limb proportions in South Africans: Secular changes, population differences and implications for stature estimation [PhD thesis]. Pretoria: University of Pretoria; 2016. [ Links ]
58. Ruff C. Body size prediction from juvenile skeletal remains. Am J Phys Anthropol. 2007;133(1):698-716. https://doi.org/10.1002/ajpa.20568 [ Links ]
 Correspondence:
Correspondence:
Mubarak Bidmos
mbidmos@qu.edu.qa
Received: 30 Aug. 2019
Revised: 24 Jan. 2020
Accepted: 12 Feb. 2020
Published: 27 May 2020
Editors: Margaret Avery, Maryna Steyn
Funding: Carnegie Corporation (NY USA); National Research Foundation (South Africa)
Supplementary Data
The supplementary data is available in pdf:[Supplementary Data]














