RESEARCH LETTER

 

Student throughput variables and properties: Varying cohort sizes

 

 

Lucas C.A. Stoop

Independent Researcher, Johannesburg, South Africa

Correspondence

 

 

---

ABSTRACT

A recent research paper described how student throughput variables and properties combine to explain the behaviour of stationary or simplified throughput systems. Such behaviour can be understood in terms of the locus of a point in the triangular admissible region of the H-S plane, where H represents headcounts and S successful credits, each depending on the system properties at that point. The efficiency of the student throughput process is given by the ratio S/H. Simplified throughput systems are characterised by stationary graduation and dropout patterns of students as well as by annual intakes of student cohorts of equal size. The effect of varying the size of the annual intakes of student cohorts is reported on here. The observations made lead to the establishment of a more generalised student throughput theory which includes the simplified theory as a special case. The generalised theory still retains the notion of a triangular admissible region in the H-S plane but with the size and shape of the triangle depending on the size of the student cohorts. The ratio S/H again emerges as the process efficiency measure for throughput systems in general with unchanged roles assigned to important system properties. This theory provides for a more fundamental understanding of student throughput systems encountered in real life.
SIGNIFICANCE:
•A generalised stationary student throughput theory through varying cohort sizes allows for a far better understanding of real student throughput systems.

Keywords: throughput efficiency; student success rates; analytical process model; graduates; dropouts

---

 

 

Introduction

A recent research paper¹ (hereafter referred to as the main paper) described how throughput variables combined with throughput properties determine the behaviour of stationary student throughput systems. Throughput variables refer to headcounts (H) and successful credits (S), and throughput properties relate to the percentages of student intakes graduating (G) or dropping out (D) from the degree annually. These percentages also determine on average the number of years for students to graduate (J) or to drop out of a degree (K). Simplified throughput system behaviour can be described by the locus of a point of H and S values in a two-dimensional triangular region, with each admissible point associated with specific system properties. Throughput process efficiency is defined by the ratio S/H. Simplified throughput systems are characterised by stationary graduation and dropout patterns of students - the consequence of annual intakes of student cohorts of equal size.

The effect of varying the size of the annual intakes of student cohorts in student throughput systems for 4-year degrees is reported on here. The assumption of annual intakes of student cohorts of equal size is important in the development of a simplified theory on student throughput, but unfortunately also a constraint when applying the simplified theory to real throughput systems in which annual student intake cohorts vary in size from year to year. A more generalised student throughput theory is therefore required to gain a more fundamental understanding of throughput systems encountered in real life. The development of such a generalised theory is reported on here and it is demonstrated that the simplified theory is indeed a special case of a more generalised theory. The focus will again be on 4-year degrees but the theory as presented can easily be extended to apply to 2- and 3-year degrees.

 

Generalised cohort survival model calculations

In the case of a 4-year degree with 8 years assumed to be the maximum time for the completion of the degree, cohort studies would require a set of eight consecutive annual intakes Nⁱ (i=1,…,8) of different student cohort sizes. According to the convention followed in the main paper, Cohort 1 is taken to be the youngest cohort of students to be enrolled and Cohort 8 the oldest. The throughput profile of each cohort is firstly defined by the percentage G_j of an intake graduating after j years of study. The throughput profile is secondly defined by the percentage D_j of an intake dropping out from the system after j years of study (part of a year is assumed to be a full year). The following equations will then apply:

where N is the average cohort size of the throughput system.

On average, the number of years taken by students to graduate at the end of the year (ranging between 4 and 8) is given by:

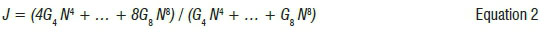
It is noted that lines of constant J are defined by different G_j values all being proportional to one another. The number of years on average taken by students dropping out at the end of the year (ranging between 1 and 8) is given by:

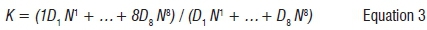
The headcount of students eventually graduating can be calculated as:

and for those students who will eventually drop out of the degree, the headcount is calculated as:

The total headcount as a system variable is therefore defined in terms of the independent sets of system properties G_j and D_j for a given set of Nⁱ as:

The annual number of successful module credits earned by students who will eventually graduate can be calculated as:

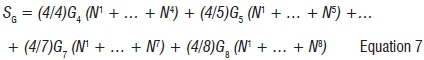
and for students eventually dropping out of the degree, the annual successful module credits are given by:

where C=0.25 is the number of successful credits earned on average by each of these students per year as is explained in the main paper. The total successful module credits earned annually by all students is therefore defined in terms of the independent sets of system properties G_j and D_j for a given set of Nⁱ as:

 

Relationship between H and S in the generalised throughput system

In the main paper it was demonstrated that for stationary throughput systems, only those combinations of H and S belonging to the admissible region of the H-S plane can be realised. As shown in Figure 1 of the main paper, the admissible region is a triangle WXZ which is bounded by the line WX (J=4), the line WZ (J=8), and the horizontal lines of G=0% and G=100%. The parameter K was set equal to 4, and this value also defines the position of the pivot W of lines of constant J and their intersection with the line G=0%. The perfect throughput system is located at X defined by H=4N=S. In triangle WXZ for a specific value of K, each combination of H and S will correspond to a unique combination of G and J, and vice versa.

 

 

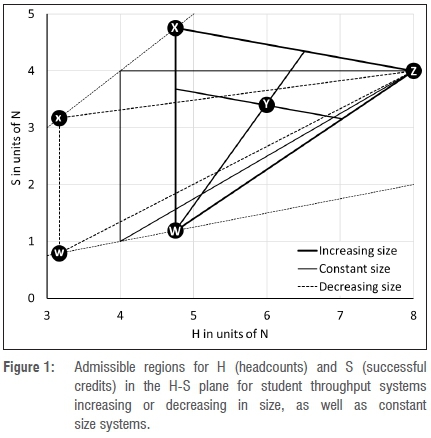
In generalising these results, a distinction will now be made between student throughput systems increasing in size as defined by:

and student throughput systems decreasing in size as defined by:

Student throughput systems where

will be referred to as constant size throughput systems. The latter include stationary throughput systems as described in the main paper where all cohorts are of equal size.

Figure 1 demonstrates how the shape of the triangular admissible region for stationary throughput systems with K=4 (solid line triangle) is skewed in the case of student throughput systems increasing in size (triangle WXZ for compound growth of 10% pa) and in the case of student throughput systems decreasing in size (triangle wxZ for compound growth of -10% pa). In triangle WXZ, for example, the coordinates of the vertices of the triangle (in units of N) become:

Vertex X where G = G₄ = 100%:

Vertex W where D = D₄ = 100% and G = 0%:

Vertex Z where G = G₈ = 100%:

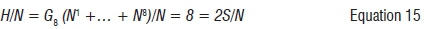
The triangle WXZ is again bounded by the line WX (J=4) along which G₄<100% and G₅=G₆=G₇=G₈=0%. The admissible region is also bounded by the line WZ (J=8) along which G₈<100% and G₄=G₅=G₆=G₇=0%. The boundary XZ represents the line G₄+G₅+G₆+G₇+G₈=100% which in general is an irregular line shaped by the specific values of Nⁱ.

It is interesting to note that the vertex X of all student throughput systems, whether increasing or decreasing in size, all lie on the line S/H=1 which is the condition defining a perfect throughput system. Hence, this condition not only applies to stationary throughput systems, but also to student throughput systems of increasing and decreasing size in general, which emphasises the importance of the condition S/H=1 as a general measure of student throughput performance. Likewise, the vertex W of all student throughput systems, whether increasing or decreasing in size, all lie on the line S/H=0.25.

The throughput configuration Y in the triangle WXZ with the values H/N=6.0 and S/N=3.4 is produced by G=70% with (G₄;G₅;G₆;G₇;G₈) = (3%;15%;28%;19%;5%) for K=4 and a given set of Nⁱ values. As in the case of the simplified throughput model, each combination of G_i values corresponds to a specific value of J, which in this case is equal to J=6.0. Lines of constant G and constant J are also shown in Figure 1.

Finally, the number of graduates produced (G₄N⁴+…+G₈N⁸) by the throughput system, is also a throughput system variable to be measured in units of N.

 

Conclusions

The present study has shown that the generalised theory on student throughput systems provides for a more fundamental understanding of real throughput systems with the simplified theory indeed a special case of the generalised theory. More specifically, the behaviour of student throughput systems depends on whether these systems are increasing, decreasing, or remain constant in size. The total size of the youngest four student cohorts N¹, N², N³ and N⁴ relative to the average size of all student cohorts, determines the size and shape of the admissible triangle of H and S values in the H-S plane. One of the advantages of the generalised theory is that the position of the relevant admissible domain in the H-S plane can be positioned accurately, instead of relying on assumptions about constant annual intakes of students. Otherwise, the main characteristics of the generalised student throughput theory appear to be logical extensions of the simplified theory. The ratio S/H again emerges as a measure of throughput process efficiency with unchanged roles assigned to the generalised values of J, K and G.

Regarding the practical use of the generalised theory, a few examples have already been discussed in the main paper to show how the simplified theory could find application to advance our understanding of student throughput systems. The generalised theory certainly would add a further dimension to our understanding of such systems, and would permit the analysis of even more complex student throughput systems which could not have been analysed before.

 

Reference

1. Stoop LCA. Relationships between student throughput variables and properties. S Afr J Sci. 2015;111(7/8), Art. #2014-0336, 5 pages. http://dx.doi.org/10.17159/sajs.2015/20140336        [ Links ]

 

 

Correspondence:
Lucas Stoop
Email: Lcastoop@gmail.com

Received: 19 Jan. 2017
Revised: 13 June 2017
Accepted: 28 Aug. 2017