Services on Demand
Article
Indicators
Related links
-
 Cited by Google
Cited by Google -
 Similars in Google
Similars in Google
Share
South African Journal of Science
On-line version ISSN 1996-7489
Print version ISSN 0038-2353
S. Afr. j. sci. vol.110 n.5-6 Pretoria Mar. 2014
RESEARCH ARTICLE
A technique to determine the electromagnetic properties of soil using moisture content
Petrus J. Coetzee
Department of Electrical, Electronic and Computer Engineering, University of Pretoria, Pretoria, South Africa
ABSTRACT
Accurate electromagnetic ground constants are required for applications such as modelling of ground wave propagation of radio signals and antennas above a real, imperfect earth and for use in geological surveys and agricultural applications. A simple method to determine the ground parameters (conductivity and relative dielectric constant) for any radio frequency is outlined here. The method has been verified over the 2-30-MHz frequency range but should be applicable up to several GHz. First, a low cost, commercial soil moisture meter using time domain reflectometry techniques is used to determine the soil moisture percentage. Then previously published universal soil models implemented on a programmable calculator or a PC are used to calculate the required constants at the frequency of interest according to the measured moisture percentage. The results obtained by this method compare favourably with those obtained by the input impedance of a low horizontal dipole technique. The received signal strength of a ground wave, HF transmission also compares favourably with that predicted by GRWave using ground constants calculated by the soil moisture technique. This method offers significant advantages in terms of simplicity, speed and cost when compared with current techniques.
Keywords: electromagnetic properties; soil moisture; time domain reflectometry; ground constants
Introduction
Accurate electromagnetic ground constants are required for many applications including modelling of ground wave propagation of radio signals, calculating clutter and reflection in radar applications, calculating the soil penetration of an electromagnetic wave and modelling antennas above a real, imperfect earth. Electromagnetic ground constants also are widely used in geological surveys and agricultural applications.
Various sources and techniques are available to determine the required constants but these approaches are generally cumbersome, may require specialised instrumentation and may not be applicable to the frequency of interest. The International Telecommunications Union (ITU) published various graphs for relative permittivity (εr) and conductivity (σ) in ITU-R Recommendation 527-3.1 However, the validity and usefulness of these ITU publications are questionable. The Institute of Electrical and Electronics Engineers (IEEE) made the following statement regarding the published data2:
The International Telecommunications Union (ITU) has published world surface conductivity maps for a number of frequency bands, although these are no longer being updated. The curves of conductivity and relative permittivity in ITU-R Recommendation 527-3 exhibit no dispersion in the band 3-30 MHz, whereas measured values show significant dispersion in the band for which surface soils typically can show characteristics from lossy conductors to lossy dielectrics. The real and imaginary parts of the complex relative permittivity form a Hilbert transform pair. As a result, the conductivity and relative permittivity are not independent variables. Their mutual coupling is described by the Kramers-Kronig relations. Therefore, the ITU values for the HF band are inconsistent with the results of complex variable theory and are in error.
This statement relating to a professional ITU standard is extraordinary and illustrates the problem with conventional values for soils at radio frequencies. The source of this misconception seems to be a report by Pearce et al.3 in which they incorrectly concluded that the relative dielectric constant is basically constant from 50 MHz to over 500 MHz. The message is clear: published graphs and tables, even by international bodies like the ITU, are not reliable or accurate enough and a better solution is required. In this paper, work done in obtaining a universal soil model is reviewed and measured data using this model are presented.
Universal soil model
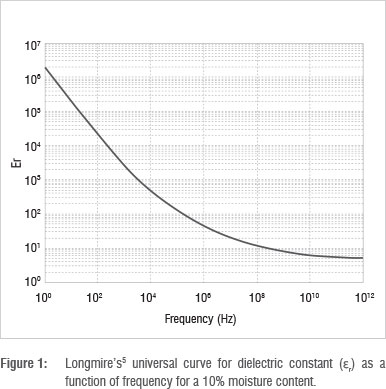
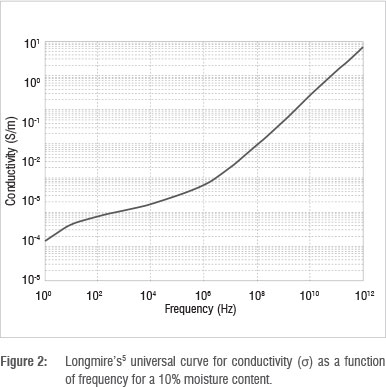
During the 1970s, Conrad L. Longmire and H. Jerry Longley, working for the Mission Research Corporation, developed a universal soil impedance model4 for the Defence Nuclear Agency. During October 1975, Longmire and Ken S. Smith expanded this model, making it valid from 1 Hz to 10 GHz.5 This research was conducted to quantify the effect of an electromagnetic pulse generated by a high altitude nuclear explosion coupling into structures and underground cables through the soil.
Electromagnetic pulse is a very fast nanosecond time domain pulse with a frequency spectrum beyond 100 MHz. Longmire modelled soil as a resistor-capacitor (RC)-transmission line with the variation of conductivity and the dielectric constant with frequency as a function of water content. Thus, if one knows the water content of the soil, one can predict with good accuracy what the value of a and εr will be at a specific frequency. Longmire and Smith based their research on work done by Scott6 in 1971.
Scott6 has presented results of measurements of the electrical conductivity and dielectric constant, over the frequency range of 100 Hz to 1 MHz, for many samples of soil and rock. He noted that the results for the many samples could be correlated quite well in terms of just one parameter: the water content. By averaging his data, he produced a set of curves, εrr(f) and σ(f), as functions of frequency (f) for various values of water content. Thus, if one knows the conductivity or the dielectric constant at one frequency, one can estimate both as functions of frequency using Scott's 'universal' curves. If the water content of a soil is known, it is possible to predict what its dielectric constant and conductivity will be at a specific frequency with generally useful accuracy.
Longmire observed that all of Scott's curves for εrr(f) would very nearly coincide with each other if displaced to the right or left, that is, that there is just one curve for εrr(f/f0), where f0 scales with water content. Longmire's contribution was thus to show how to use the frequency-dependent parameters to formulate a time-domain treatment of electromagnetic problems. The time-domain method solved Maxwell's equations in dispersive soils, based on the assumption that each volume element of the soil could be represented by an RC network. The real and imaginary parts of this model are related to the conductivity and the dielectric constant, respectively. A consequence of the RC network model is that the variation of dielectric constant and conductivity with frequency are not independent. It is also clear that the dielectric constant increases with frequency and conductivity decreases with frequency. In terms of the RC network, this means that as the water content is varied, only the R values change, while the C values remain fixed.
Wilkenfeld measured the conductivity and dielectric constant of several samples of grout and concrete over the frequency range 1-200 MHz. From the data published by Scott6 and Wilkenfeld, Longmire and Smith developed a universal soil impedance (actually, admittance) model that operates from 1 Hz to 10 GHz and includes both Scott's and Wilkenfeld's data. The 10% moisture curves are taken as references and are scaled to the left or right for different moisture values.
The surface wave component of an electromagnetic wave propagates along and is guided by the earth's surface, similar to the way in which an electromagnetic wave is guided along a transmission line. Charges are induced in the ground by the surface wave. These charges travel with the surface wave and create a current in the ground. The ground carrying this current can be represented by a leaky capacitor (a resistance R shunted by a capacitive reactance C). The characteristics of the ground as a conductor can therefore be represented by an equivalent parallel RC circuit, where the ground's conductivity can be simulated with a resistor and the ground's dielectric constant by a capacitor. Figure 3 is a generalised version of the Debye model.7 At medium-wave frequencies (300 kHz-3 MHz) the soil characteristics are dominated by the resistance, but at higher frequencies the soil is both resistive and capacitive.
In Figure 3, R0 is the resistance at zero frequency (direct current) and is the capacitance at infinite frequency. The other branches provide transient responses with various time constants.
Determining the soil moisture content
Various sources and techniques are available to determine the required parameters - relative dielectric constant and ground conductivity - but they all tend to be cumbersome in some way or another. According to the IEEE the main techniques are:
- direct current resistivity methods
- surface impedance methods using very low frequency (up to 20 kHz) signals
- propagation studies in which the receiver is sometimes located underground (also limited to very low frequency)
- wave tilt method
- self-impedance methods
- mutual impedance methods
- time domain reflectometry
The drawback of all these techniques, with the possible exception of time domain reflectometry, is that they cannot easily provide the required soil parameters at a specific frequency.
Time domain reflectometry
Time domain reflectometry (TDR) uses a narrow pulse of electromagnetic energy at one end of a parallel transmission line located in a lossy material. The characteristics of the reflected waveform are influenced by the dielectric properties of the medium. In the 1980s, Clarke Topp, a soil physicist working for Agriculture Canada, was approaching the problem from the time domain direction and, without knowledge of Longmire's work, used TDR to measure soil conductivity and dielectric constant and from there determined the moisture content.8 Soil physicists have since extensively researched and documented TDR methods to obtain soil moisture content.
Originally used primarily for testing high-speed communication cables, TDR is a complex electronic technology. The early development of TDR for the unusual application of measuring water content in soils began somewhat by chance and continued almost in spite of the goals set by the supporting organisations. TDR was originally used on coaxial transmission lines filled with soils in the laboratory. However, coaxial transmission lines are not practical for measurements in the field and techniques were developed to use parallel transmission lines consisting of two parallel rods placed in the soil. Originally, TDR cable testers were used for measurements in the field and the TDR waveform from the oscilloscope screen was later manually measured using a ruler. Clearly there was a need for a TDR instrument that could measure soil water content directly instead of recording travel time as cable lengths.
With the advent of powerful microprocessors it became possible to perform the entire full waveform signal processing on a single integrated circuit and directly display the soil moisture content on a liquid crystal display. These modern microprocessors are very compact and power efficient, making battery-powered portable equipment possible.
Commercial equipment employing the TDR technique to measure soil moisture content is now freely available and is used to measure the soil water content on golf courses and in the agricultural sector.
It is thus now possible to easily and accurately determine soil moisture content using RF techniques (TDR) and to use the moisture percentage in conjunction with Longmire's soil impedance model to determine the conductivity and dielectric constant for the applicable frequency of interest. This technique is a major breakthrough and promises enhanced accuracy for the modelling of the effect of a real, imperfect ground on electromagnetic signals.
The low horizontal dipole
In free space, a half-wave (λ/2) dipole antenna has an impedance of very nearly 72 + j0 Ω at resonance. This input impedance changes as the antenna is brought closer to the surface of an imperfect earth.
An antenna can thus be used as a 'geological probe'. Nicol9 published graphs to determine the electromagnetic parameters of soil (εr and σ) from measuring the input impedance of a thin, half-wave dipole at heights of 0.05 λ and 0.02 λ. Note the interdependency of all the variables, including operating frequency, in Nicol's work.
An antenna modelling program (EZNEC)10 based on numerical electromagnetic code (NEC-2) can be used to calculate the input impedance of a thin dipole close to the ground, according to the defined ground constants. Using the graphs of Longmire and Smith5 to determine the soil constants for 10% soil moisture at a frequency of 5 MHz yields εr = 24.61 and σ = 0.0046. The wavelength at 5 MHz is nearly 60 m; a half-wave dipole antenna is thus nearly 30 m in length. If the height is taken as 0.02 λ (1.2 m) EZNEC calculates the input impedance at 5 MHz as: Z = 79.72 + J69.71 Ω. According to the graph published by Nicol9 the input impedance is approximately 80 + j70 Ω. The values correlate rather well. The values also correlate well when the process is repeated for a dipole at a height of 0.05 λ. It is, however, a bit difficult to read the values accurately from Nicol's graphs. Longmire and Smith's technique is considerably more exact.
Practical application of time domain reflectometry
The received power level of a ground wave, high-frequency (3-30 MHz) signal between Pretoria and the National Antenna Test Range at Paardefontein,11 north of Pretoria, was measured and compared to the value calculated by the GRWave computer model. GRWave is based on the theory of Rotheram12,13. The program was modified for execution on a PC by Dr John Cavanagh of the Naval Surface Warfare Centre in July 1988.14 Later, the CCIR adopted the program to compute ground wave transmission loss.15 GRWave is published by the ITU.16 The program can be used to determine transmission loss and field strength transmission loss from the designated transmitter to the designated receiver. The GRWave model considers a smooth (no terrain obstacles), homogeneous (a single set of ground constants), spherical earth bounded by a troposphere with exponential height variation. GRWave uses three different methods to calculate field strength depending on wavelength (A), path length (d) and antenna height (h) relative to the earth's radius (a). At longer distances (d > λ1/3a2/3 and h < λ2/3a1/3), the residue series is used; at shorter distances (h > λ1/3a2/3 and h < λ2/3a1/3), the model employs the extended form of the Sommerfeld17 flat-earth theory, and geometric optics are used to calculate field strength at distances not covered by either residue series or the Sommerfeld theory (h > λ2/3a1/3 and d within the radio horizon). GRWave requires frequency, polarisation, power, ground relative dielectric constant and conductivity, lower and higher antenna heights, and distance as inputs.
A 100-W HF transmitter in conjunction with a wideband, monopole antenna with known characteristics operating against a ground screen was used at the Pretoria site. A continuous wave signal was transmitted on the selected frequency under command of the receive site.
A calibrated receiving antenna (Rohde & Schwarz HFH2-Z1, Munich, Germany) and a test receiver (Rohde & Schwarz ESH 3) were used to measure the received field strength (in dB/µV/m) at Paardefontein. The measured field strength was converted to received power (dBm) and compared to the value calculated by GRWave for the specified operating conditions.
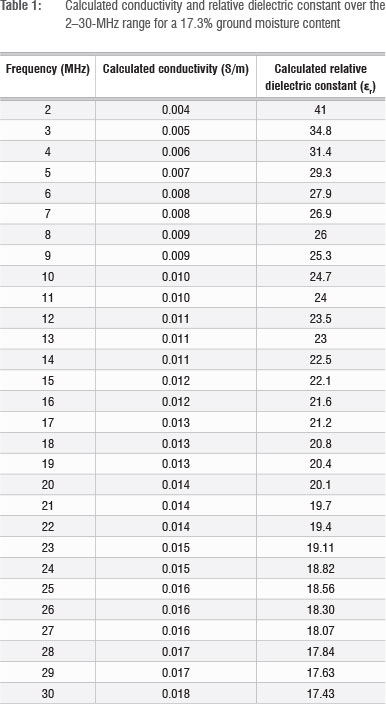
A Spectrum Technologies Field Scout TDR 300 (Aurora, IL, USA) soil moisture meter was used to determine the moisture percentage at various points between Pretoria and Paardefontein. The average moisture content was 17.3%, from which the conductivity and relative dielectric constant were calculated for the applicable test frequency.
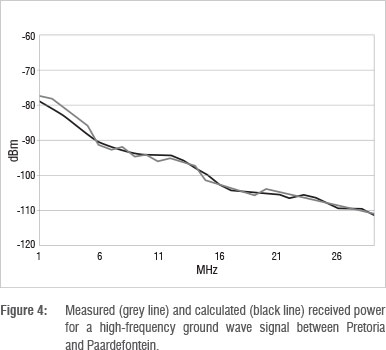
The tests were conducted over a distance of 23.56 km. The measured and the GRWave calculated received power levels are compared in Figure 4. As seen in Figure 4, the measured and calculated results correlate very well. The fact that Paardefontein is located in open countryside with no major mountains between Paardefontein and the test transmitters' location in Pretoria contributed positively to the result. Hilly terrain would probably have a negative impact on the results.
Conclusion
The universal soil impedance model and moisture percentage technique offers significant advantages in terms of simplicity, speed and cost in determining the electromagnetic properties of soil (ε r and σ) at any frequency of interest when compared with current techniques.
With the correct electromagnetic ground constants for the applicable frequency, it is now possible to more accurately model ground wave propagation, transmitter area coverage, ground penetration of a RF signal, radiation patterns and input impedances of HF antennas as well as ground reflections at reflective antenna test ranges such as the National Antenna Test Range at Paardefontein.
Acknowledgement
I gratefully acknowledge the support of my colleague Dion F. Janse van Rensburg in the development of the above technique.
References
1. ITU-R. Recommendation P. 527-3: Electrical characteristics of the surface of the earth. ITU-R Recommendations P Series (Radiowave Propagation). Geneva: ITU; 2000. [ Links ]
2. IEEE. Guide for measurements of electromagnetic properties of earth media. IEEE Std 356-2001. New York: Institute of Electrical and Electronics Engineers; 2002. [ Links ]
3. Pearce DC, Hulse WH, Walker JW. The application of the theory of heterogeneous dielectrics to low surface area soil systems. IEEE Trans Geosci Electron. 1973;11:167. http://dx.doi.org/10.1109/TGE.1973.294311
4. Longmire GL, Longley HJ. Time domain treatment of media with frequency-dependent electrical parameters. Theoretical Notes, note 113. Santa Barbara, CA: Mission Research Corporation; 1973.
5. Longmire GL, Smith KS. A universal impedance for soils. Topical report for period 1 July 1975 to 30 Sept. 1975. Report no. MRG-N-214. Santa Barbara, CA: Mission Research Corporation; 1975.
6. Scott JH. Electrical and magnetic properties of rock and soils. Electromagnetic Pulse Theoretical Notes, note 18. AFWL EMP 2-1. April 1971. [ Links ]
7. Debye P. Polar molecules. Mineola, NY: Dover; 1929.
8. Topp GC, Davis JL, Annan AFP Electromagnetic determination of soil water content: Measurements in coaxial transmission lines. Water Resour Res. 1980;16:574-582. http://dx.doi.org/10.1029/WR016i003p00574 [ Links ]
9. Nicol JL. The input impedance of horizontal antennas above an imperfect earth. Radio Sci. 1980;15:471-477. http://dx.doi.org/10.1029/RS015i003p00471 [ Links ]
10. Lewallen RW. EZNEC v.4.0.39. Beaverton, OR: EZNEC; 2007. Available from: www.eznec.com [ Links ]
11. Armscor Defence Institutes. The South African National Antenna Test Range [homepage on the Internet]. No date [cited 2013 Nov 21]. Available from: http://www.paardefontein.co.za [ Links ]
12. Rotheram S. Ground wave propagation I: Theory for short distances. Proc IEE Part F. 1981;128:275-284.
13. Rotheram S. Ground wave propagation II: Theory for medium and long distances and reference propagation curves. Proc IEE Part F. 1981;128:285-295.
14. Cavanagh JF. GRWAVE release 2. Washington DC: Naval Surface Warfare Centre; 1985.
15. CCIR Report 714-2. Ground wave propagation in an exponential atmosphere. Geneva: ITU; 1990.
16. ITU-R. rsg3-grwave. Geneva: ITU; 2009. Available from: http://www.itu.int/oth/R0A0400000F/en [ Links ]
17. Sommerfeld AN. The propagation of waves in wireless telegraphy. Ann Physik. 1909;28:665-736. http://dx.doi.org/10.1002/andp.19093330402
 Correspondence:
Correspondence:
Petrus Coetzee
Department of Electrical, Electronic and Computer Engineering
University of Pretoria, Private Bag X20
Hatfield 0028, South Africa
hcoetzee@gew.co.za
Received: 22 July 2013
Revised: 21 Nov. 2013
Accepted: 24 Nov. 2013














